Overview of Section 2.2 Circles Inscribed in Polygons

If a circle is inscribed in a polygon, the circle is tangent to each of the sides of the polygon. This equivalent to saying a polygon circumscribes a circle. We also say a polygon is circumscribable, meaning a circle can be inscribed in a polygon.

Theorem 2.5: Inscribed circle of a triangle and its incenter. The angle bisectors of any triangle intersect at a single point O which is the center of the inscribed circle. The distance from O to any of the sides of the triangle is the radius of the inscribed circle.

The inscribed circle is also called the incircle. PROOF covered previously in Chapter 1 . . . find it.

 

Corollary 2.5: The angle bisectors of the two exterior angles on one side of a triangle are concurrent with the angle bisector of the opposite interior angle of the triangle. SEE Problem 1.2.9.

 

 

 

 

Theorem 2.6: From a point external to a circle, the two tangent segments are congruent.

That is, segments AP and AQ are congruent.

Proof plan: Show triangles APO and AQO are congruent by HL

 

Circles Inscribed in Quadrilaterals

 

Theorem 2.7: A circle can be inscribed in a quadrilateral if and only if the angle bisectors of the four angles of the quadrilateral are concurrent.

 

 

Proof.

If given a circle with center O inscribed in a quadrilateral, point O is equidistant from each of the sides so point O must lie on the angle bisector of each pair of sides.

Conversely, if O is a point on each of the angle bisectors of adjacent pairs of sides, then O is equidistant from each of those sides.

 

 

 

Example 2.2. Prove that a circle can be inscribed in every kite.

Comment: The proof is accomplished using Theorem 2.7 by showing the angle bisectors or a kite are concurrent. The strategy is to show pairs of congruent triangles in the kite and use the corresponding parts.

 

A Relationship Among the Sides of a Circumscribable Quadrilateral

Theorem 2.8: If a quadrilateral is circumscribable, then the sum of the lengths of two opposite sides equals the sum of the lengths of the sides of the two remaining sides.

The motivation and proof follows from Theorem 2.6. The two tangent segments from each vertex of the quadrilateral to the tangent points with the inscribed circle are congruent.

EQUIVALENT STATEMENTS for Theorem 2.8:

1. A necessary condition for a quadrilateral to be circumscribable is that the sum of the measures of two opposite sides is the same as the measures of the two remaining opposite sides.

2. If the sum of the measures of two opposite sides does not equal the sum of the measures of the remaining two opposite sides, then the quadrilateral is not circumscribable. (the contrapositive)

 

Example 2.3.

1. Prove that if a parallelogram is not a rhombus, it is not circumscribable.

Comment: Prove by using the contrapositive form of Theorem 2.8.

 

2. On the basis of the theorems proved so far, would a quadrilateral with sides of length 3, 2, 5, 6 be circumscribable?

This would require the converse of Theorem 2.8.

Theorem 2.9 -- The converse of Theorem 2.8

If the sum of the measures of two opposite sides of a quadrilateral equals the sum of the measures of the two remaining opposite sides, then the quadrilateral is circumscribable.

The proof provided in the text first proves the theorem for the special case when ABCD is a kite and AB+DC = AD + BC. That proof was in Example 2.2.

The next part of the strategy is to take the case when two adjacent sides, say AB and AD are not congruent. Let AB > AD

Then from AB + DC = AD + BC can be rewritten AB - AD = BC - DC.

Because we let AB > AD, AB-BD > 0 and so BC - DC > 0. This means BC > DC. For reference, we have this figure:

 

 

Next, construct E and F on AB and BC, respectively, so that

AD = AE and CF = DC.

Construct EF, ED, and FD.

We have isosceles triangles DCF and DAE

Thus AB - AD = BC - DC can be replaced by AB - AE = BC - CF.

So, BE = BF and triangle BEF is isosceles.

We are interested in the angle bisectors of the quadrilateral. But, the angle bisectors of angles A, C, and B are the perpendicular bisectors of the sides of isosceles triangle DEF and these are concurrent.

 

 

 

 

 

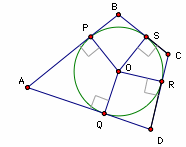
Let O, be the intersection and construct perpendiculars to each of the sides of the quadrilateral. We have established that O is a point of concurrency for the angle bisectors of angles A, B, and C. It remains to show that O is on the angle bisector of angle D.

Point O on the angle bisector of angle B implies OP = OQ.

Point O on the angle bisector of angle A implies OP = OS

Point O on the angle bisector of angle C implies OQ = OR

Therefore OS = OR and point O is on the angle bisector of angle D.

 

NOW SOLVE THIS 2.6

Why was it important (needed?) to prove the special case when the quadrilateral was a kite?

Could it be that the figure DEBF we used in the proof was a kite?

 

Theorem 2.10: A quadrilateral is circumscribable if and only if the sum of the lengths of two of its opposite sides equals the sum of the lengths of the other two sides.

Theorem 2.10 is a a combination of Theorem 2.08 (Necessary) and Theorem 2.09 (Sufficient) conditions.

 

Example 2.4.

Three different approaches to this proof are given in the text.

The first uses the idea that BC = CE and seeks a way to show triangle CED is isosceles.

The second uses the mid-segment theorem and the fact that OBCE is a kite.

The third shows that OC is parallel to AD. Click HERE for an available GSP sketch.

 

Example 2.5

COMMENT: The NOW SOLVE THIS 2.8 outlines a proof of this. I believe the labels provided are in error. In line 1, Angle APD should be angle ACD, and in line 2 angle ACD should be angle APB.

The strategy is to construct a triangle ACD by extending CP beyond P a distance of AP to a point D. Thus a new triangle ACD is created and if that triangle could be shown congruent to triangle ABP, the proof would be implemented. Click HERE of a GSP file.

 

 

Problem Set 2.2 Discussion and Solution hints.