

Overview of Section 4.3 Areas of Similar Figures
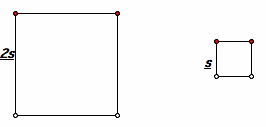
In this section, we move from similar polygons defined by a common ratio of corresponding sides and corresponding angles congruent to an exploration of the comparison of the areas. Intuitively, this relationship might be motivated by examining specific cases. For example, if we have two squares such than the side length of one is twice the other, the ratios of the sides will be r = 2. The ratio of the areas will be 4.
If we take any triangle and construct the segments cutting the midpoints, from the mid-segment theorem for triangles we know that each of the mid-segments is half the length of the third side and parallel to it. From this we can conclude that the triangle is subdivided into 4 congruent triangles, each similar to the original triangle. Thus the ratio of the sides is 2 and the ratio of the areas is 4.
Subdividing each side of a triangle or a square into thirds and constructing segments parallel to the sides will produce similar figures with sides one-third the length of the original. In each case there are 9 similar figures formed. So the ratio of the sides is 3, the ratio of the areas is 9.
Clearly, this strategy could be extended to dividing a side into n parts. We will do a proof in two steps. In the first case, it is established that the ratio of areas of similar triangles equals the square of the ratio of the corresponding sides. Then we will extend the theorem to any polygon by dividing the polygon into triangular regions. First, a couple of lemmas.
Lemma 4.1. If two triangles are similar with ratio r, then their corresponding altitudes are in the same ratio.
Proof: Given similar triangles ABC and A'B'C'. Construct the corresponding altitudes CD and C'D'. Now triangle ADC is similar to triangle A'D'C' by the AA similarity condition.
Lemma 4.2. Similar convex polygons in the plane can be partitioned into a set of similar triangles by constructing the diagonals from any vertex.
Corollary 4.2: The diagonals of similar convex polygons in the plane are in the same ratio.
Proof: Left as an exercise . . . Essentially use the SAS similarity condition to show that two sides of the similar polygons subtending and corresponding diagonals will give similar triangles.
Theorem 4.13: The ratio of areas of similar triangles equals the square of the ratio of corresponding sides.
In the triangles ABC and A'B'C' (figure in Lemma 4.1), Let S be the area of triangle ABC and S' the area of triangle A'B'C. Then
QED
Theorem 4.14: The ratio of areas of similar polygons equals the square of the ratio of corresponding sides.
NOTE: Although the statement of the theorem here and in the textbook is not explicit, I think we are assuming this is for convex polygons in the plane. Additional care would be needed to establish the theorem for concave polygons in the plane, for polygons in the plane that were not simple (i.e. polygons whose sides might cross), or for non-planar polygons.
Proof: Use Lemma 4.2. Take two similar polygons with areas of S and S'. Since the polygons are similar,
Now Solve This 4.10
Is it true that the viewing area of a 35-inch television screen is about twice the viewing area of a 25-inch television screen. Justify your answer.
Assume the two screens are similar rectangles. From the Lemmas and Theorems 4.13 and 4.14, we know that the ratio of the corresponding sides is the same as the ratio of the diagonals and so r = 35/25. The ratio of the areas is thus 1.96, which is 'approximately' equal to 2.
Let S be the area of triangle ABC. OBSERVE THAT THE AREA OF THE PARALLELOGRAM CQPM WILL BE
Example 4.5.
Given Triangle ABC, construct a line parallel to the base that divides the triangle into two equal areas.
Thinkaboutit: If the ratio of the areas of two similar triangles is 2, what is the ratio of the sides?
Link here for a GSP file with a script tool for this construction: GSP File
Construction:
GENERALIZATION
SEE GSP FILE for These constructions and Script Tools
An extension: For any triangle ABC a segment is drawn that is parallel to the base. A small triangle in the center is formed, obviously similar to ABC. What is the ratio of the areas of this small triangle and triangle ABC.
GSP File Solution from Jackie Ruff.
See Half the Area of the Triangle from EMAT 6600 for more . . .
Another extension: Construct a line parallel to the parallel bases of a trapezoid to divide the trapezoid into two equal areas.
Allison Hallman has a solution . . .
See http://jwilson.coe.uga.edu/emt725/IsosTrapEqAr/IsosTrapEqAr.html
I found it useful to find an algebraic expression for the length of the segment parallel to the bases that divides the trapezoid into two equal areas.
The formula to c suggests beginning with a right triangle having legs a and b to accomplish the construction.
Theorem 4.15: If three similar polygons are constructed on the three sides of a right triangle, then the area of the polygon on the hypotenuse equals the sum of the areas of the polygons on the other sides.