This page continues a discussion of the effect of the linear coefficient (b) on a quadratic function of the form:
Assignment 2, Parabolic Vertices,
discussed the value of b's effect upon the position of the graph of the
parabola. To view this assignment, Click Here.
Graphs on the xb-plane
To continue the discussion, this page will analyze how the value of b is related to the quadratic formula and the solution of quadratic equations in the form:
One way to look at the effect of b upon the solutions of an equation in this form is to graph it with respect to x and b. This provides a different graph, describing a family of equations, as opposed to the singular graph seen when the Cartesian plane (with x and y axes) is used. Refer to the graph below:
The graph of: 
It is important to consider the axes
of this graph. The horizontal axis is the x-axis, as it is usually named. The horizontal
axis, however, is the b-axis. This graph shows the value(s) of x for any given
value of b.
This is useful for several reasons.
The Roots of a Quadratic Equation
First, if an arbitrary value of b is chosen, it is possible solve any equation of the form graphed above. For example, a visual representation of this solution is presented below for the value b = 4.
The value of b = 4 is represented by the red horizontal line. Note that it intersects the previous graph in two places. The x-coordinates of the points of intersection are the roots, or solutions to the equation
These solutions correspond to the roots
of the parabola formed by graphing the function 
Click Here to view a graph of this parabola.
Discriminants and the Number of Solutions (Roots)
The graph above provides insight regarding the value of the discriminant of the quadratic equation. If you need to refresh your memory about discriminants, Click Here.
As you can see below, there are some values for b where the horizontal line intersects the graph at two points (such as b = 4), some values for b where the horizontal line intersects the graph at exactly one point (b = 2 and b = -2), and some values for b where the horizontal line does not intersect the graph (b = -1).
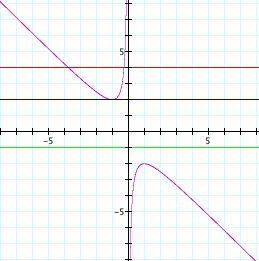
Thus, a real solution to the quadratic
equation is achieved when the absoute value
of b
is greater than or equal to 2.
Can you verify this result algebraically?
Click Here to see a verification.
Changing the value of c
What happens if the value of c is changed? The blue graph below represents the function
, reflecting
c = -1.
Note that any horizontal line drawn will
intersect this new graph, indicating that real solutions exist
for all values of b.
The Symmetric Nature of Roots
In the Cartesian plane, the axis of symmetry of a parabola is the vertical line dividing the parabola into two congruent halves. The x-value that describes the location of the axis of symmetry is the average (arithmetic mean) of the roots of the parabola. How can the axis of symmetry be incorporated into the xb-plane graphs used in this investigation?
First, it is helpful to know the equation of the Cartesian axis of symmetry. It is the found within the quadratic formula and can be stated as:
Since a = 1, this formula can be transformed into the linear equation 2x + b = 0. By including the graph of this equation with the above graphs in the xb-plane, the relationship between the axis of symmetry and the roots of a Cartesian parabola is confirmed.
Note that the horizontal distance, in either direction, from each point of the line representing the axis of symmetry (green) to either of the previous curves is uniform.