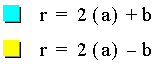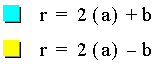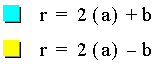
Make the following observations about the graphs
of the equation when k is odd and the go on to investigate
graphs of this equation when "k is even."
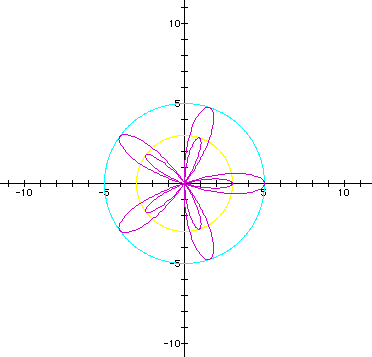
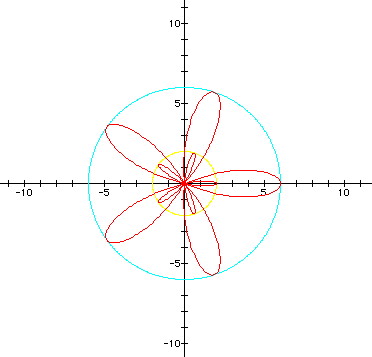
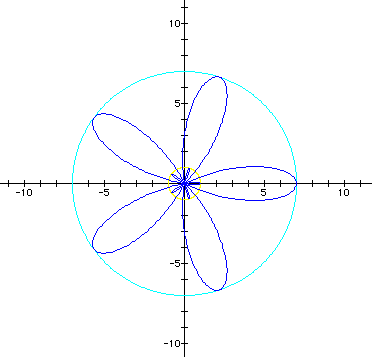
Since k is odd, recall that there are k petals with a petal lying on the positive x-axis. We can classify the graphs into three groups:
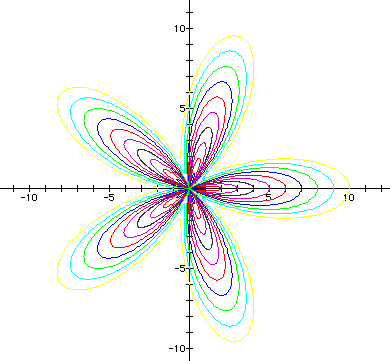
1. Single roses with closed petals that converge at the origin. This occurs when b = 0 and when b = 2a. The radius of the rose is given by r = 2a + b.
(graphs in black and green).
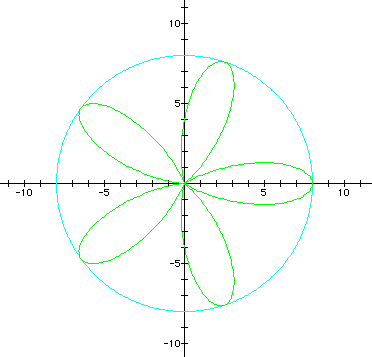
2. Double roses composed of outer and inner petals. This occurs when b < 2a. There are two radii to consider: the radius of the (1) outer rose is given by r = 2a + b. (2) the inner rose is given by r = 2a - b.
(graphs in purple, red, and blue)
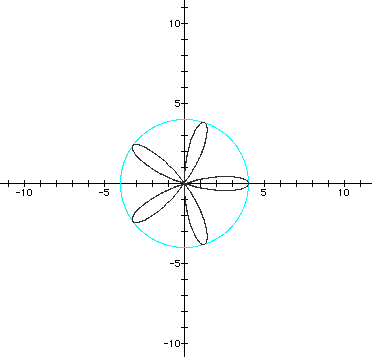
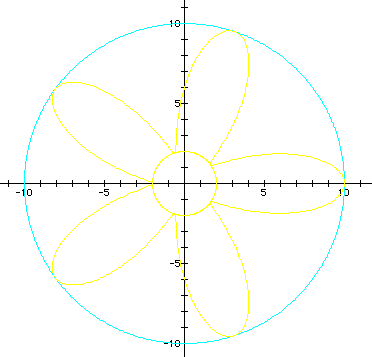
3. Open-petaled roses with an opening or core in the center. This occurs when b > 2a. There are two radii to consider: the radius of the (1) rose is given by r = 2a + b. (2) the core is given by r = 2a - b.
(graphs in cyan and yellow)
NOTE: The graphs of the equations with negative values of b look the same. Perhaps they are simply reflections about the y-axis. In cases where the radius was r = 2a + b in a positive b graph, the radius for a negative b graph has a radius of r = 2a - b. And in cases where the radius was r = 2a - b in a positive b graph, the radius for a negative b graph has a rdius of r = 2a + b.
Also, recall that if a is negative, the graph is reflected about the y-axis. And remember that a negative value for k has no effect on the graph since cos Q = cos (- Q).