

Assignment #1
Explorations of Linear Functions
by
Kim Burrell
The study of functions is taught across the world. Mathematical operations can be applied to functions. A linear function is often represented by f(x). The graph of a linear function, f(x) = x, is a straight line (figure below).
The following is a visual investigation of the linear functions and four mathematical operations on them. We will now take a look at graphs when the coefficients of the linear functions are greater than 2. Explorations will include when the coefficients are both positive, both negative, and one of each sign (one positive & one negative).
I will use the following notation:
h(x) = f(x) + g(x) represents addition,
h(x) = f(x)g(x) represents multiplication,
h(x) = f(x)/g(x) represents division, and
h(x) = f(g(x)) represents composition.
When the coefficients are both positive
Let's let f(x) = 2x + 4 and g(x) = 4x + 6. These graphs are shown below. Compare these graphs with the linear function of f(x) = x. What is different? What is similar? What type of funtion is formed? If a linear function, is the slope positive or negative?

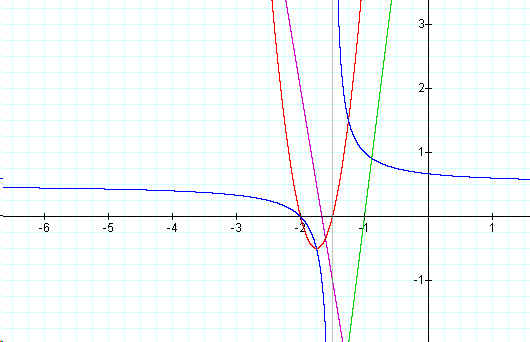
When the coefficients are both negative
Let's let f(x) = -2x - 4and g(x) = -4x - 6. These graphs are shown below. Compare these graphs with the linear function of f(x) = x. What is different? What is similar? What type of funtion is formed? If a linear function, is the slope positive or negative?
When the coefficients have different signs
Let's let f(x) = -2x + 4and g(x) = 4x - 6. These graphs are shown below. Compare these graphs with the linear function of f(x) = x. What is different? What is similar? What type of funtion is formed? If a linear function, is the slope positive or negative?

Make a conjecture about the functions f(x) and g(x) with the above mentioned operations performed on them.
How do you think the graphs of f(x) = 2x - 4 and g(x) = -4x + 6 will look like when the above operations are performed on them?
Summary
From the above observations, you can see that the graphs of functions are different for different signs of the coefficients.
When the coefficients are both positive, the graph by addition is still a linear function with positive slope. The graph by multiplication is a quadratic function. The graph by division is a hyperbola. The graph by composition is a linear function with positive slope, but not the same slope as the graph by addition.
When the coefficients are both negative, the graph by addition is still a linear function with negative slope. The graph by multiplication is a quadratic function. The graph by division is a hyperbola. The graph by composition is a linear function with positive slope.
When the coefficients are different signs, the graph by addition is still a linear function with positive slope. The graph by multiplication is a quadratic function. The graph by division is a hyperbola. The graph by composition is a linear function with negative slope.