Setting the values of a and b to 1, we can take an initial look at the parametric equations .

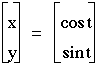
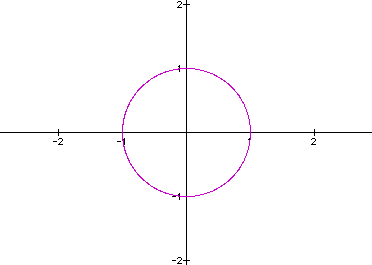
We recognize these equations to be a circle of radius 1. We can convert these equations to rectangular form if we care to confirm this.

As we increase the value of a, we can see the graph expand in the x-direction creating an ellipse. The graphs below are for integer values of a from 1 to 5.

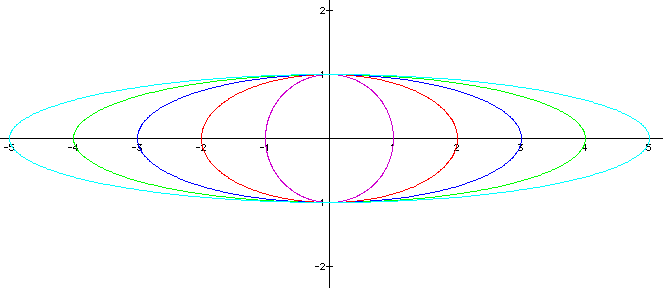
The longer distance from one vertex to the other (in the x-direction in this case) is called the major axis. The shorter distance from one vertex to the other (in the y-direction in this case) is called the minor axis. How long will the major axis be if the value of a=7?

Will this trend continue if we use non-integer values of a? What will happen when ? Results

What will happen if we use negative values for a? Explain (you might want to try a graph that does not have so much symmetry).

What will be the result if we set a=1 and vary the value of b? Results

Continue to play with the values of a and b both independently and jointly. What trends do you notice? What happens when a=b?

What will happen if we change the range of our t-values?

We can extend this type of exploration of parametric equations indefinitely. You might next want to take a look at the equations .