Polar Equations
Investigate the graph of:
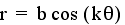
This is a special case of the graphs
of 
. In theses cases, a = 0.
Begin by investigating the graphs when
b=1 and k varies among the integers.
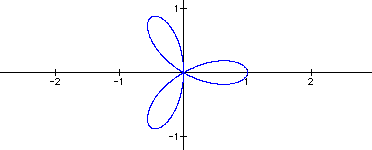
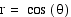, k
=1
, k
= 2
, k
= 3
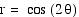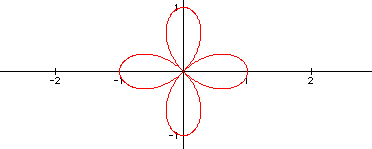
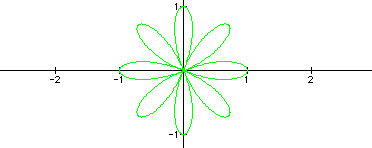
, k
= 4
To view other values of k, open this animation
on Graphing Calculator.
We can already note some similarities
and differences if we compare these graphs to those with a constant
of a added to them.
First, the graphs where k = 3, 5, 7,
etc. (odd integers) have the same number of leaves as those of
the form 
. Thus, when k is odd, the rose
has k leaves. The angle between the leaves is the same as before
as well, i.e., equal to 
. When k is odd,
the graph is symmetric with respect to the x-axis.
When k is even, however, the number
of leaves is equal to 2k. In addition, the angle between each
leaf is equal to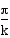 
. When k is even,
the graph is symmetric with respect to both the x-axis and the
y-axis.
When k = 1, the graph is no longer a
leaf of a rose, but a circle with a radius one-half of k.
Next, investigate the graphs of 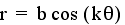as we vary b and keep k constant.
Below are examples where k = 4 and b
= 1,
2, and
3. Notice
how the length of the leaves changes in each case. The length
of each leaf is equal to b. This makes sense since the length
of each leaf for the equations is equal to
and we are considering the special
case where a = 0. In general, the length of each leaf of the graph
of 
is equal to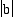 
.
Negative b?
Since the graph is symmetric with respect
to the y-axis when k is even, a negative value of b will produce
the same graph as the positive value of b. Thus, we need only
to investigate negative b-values for equations when k is odd.
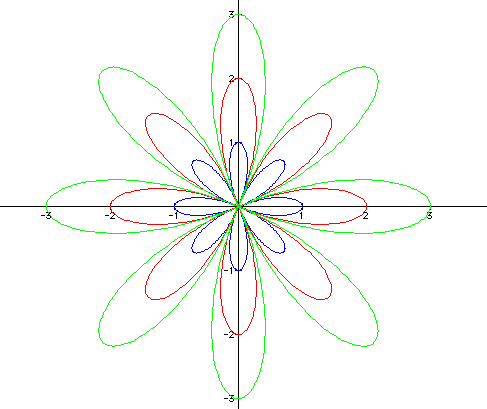
Below is an illustration of the graphs when k = 3 and b = -3, -2, -1, 1, 2, and 3. When b is negative,
the graph is rotated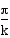
radians from the graph when b is
positive. This is the smallest angle of rotation that will produce
the graph for a negative b-value. The graph could also be rotated
by 
radians. In other words, the graph
is reflected across the y-axis. This same phenomemnom occurs with
the graphs of . Notice that the length of each leaf is equal
to 
.
To summarize my findings for the graphs
of :
- The graph is always symmetric with
respect to the x-axis. The graph is symmetric with respect to
the y-axis when k is even. If k is odd, a negative b-value reflects
the graph across the y-axis.
- If k is odd, the rose has k leaves,
each separated by
radians.
- If k is even, the rose has 2k leaves,
each separated by
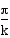radians.
Click here to
view the graph of and change the
values of b and k for yourself!
Return to the
graphs of .