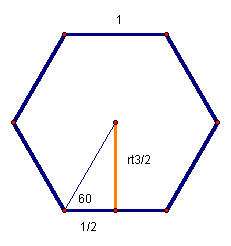

Let one side of a regular hexagon be 1. Area of a regular polygon = 1/2 Pa where P is the perimeter and a is the length of the apothem. Thus area of the hexagon = 1/2 (6) (√3/2) = 3√3/2.
Draw a hexagon inside the original hexagon.

Extend a perpendicular to create a 30-60-90 triangle. Thus the area of a yellow triangle is A = 1/2 (1-x)(x√3 /2). Since there are 6 congruent shaded triangles we multiply by 6 to get 3(1-x)(x√3 /2) = 3/2(x√3 - x²√3). To get the area of the inner hexagon, we find the difference in the area of the original hexagon and the 6 triangles: 3√3 /2 - 3/2( x√3 - x²√3). The ratio of the areas is thus (3√3 /2 - (3x√3 /2 - 3x²√3 /2) / (3√3 /2) = (3√3 /2 - 3x√3 /2 + 3x²√3 /2) / (3√3 /2) which simplifies to 1-x + x².
RETURN to essay 2