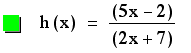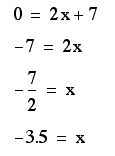DIVISION OF LINEAR FUNCITONS

Here is the algebraic representation of dividing these linear functions.

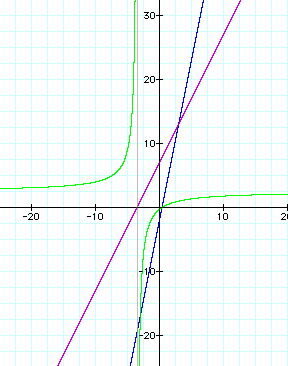
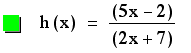We definately get something interesting here.

There is an asymptote for the quotient function h(x), which is pictured as a grey line on the graph.

How do we know where this asymptote will be?

Well, as we saw on the first page, we know that the denomenator cannot equal zero.

So, what the asymptote represents is each side of the graph approaching that point when the denomenator equals zero.

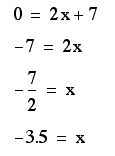It is a bit easier to see in this picture that the asymptote crosses at -3.5, just as our algebra tells us.

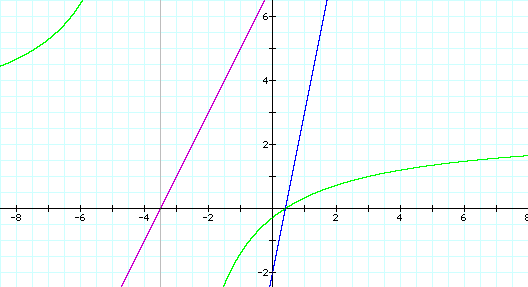To know that this line represents an asymptote rather than just a hole in the graph it may be helpful

to look at a chart that numerically shows what x does as it approaches -3.5 from both sides.

 

X	h(x)
-3.6	100-3.5
-3.55	197.5
-3.51	977.5
-3.49	-972.5
-3.45	-192.5
-3.4	-95

From this chart we can see that the closer we get to -3.5, the numbers begin to approach infinity.

 

So, we can conclude that from the quotient of two linear functions we will get and asymptote and two hyperbolas.

Discontinuity will occur when the denominator equals zero, and the asymptote will be at x= -d / c fro our general equations.

 

Return to Main Investigation