EMAT 6680 Assignment 4

Centers of a Triangle

Problem #7

By

Laura King 

ORTHIC Triangles

Orthic Triangles for Acute Triangles

The ORTHIC triangle is a triangle made up of the feet of the altitudes for the original triangle.Below are some examples of ORTHIC triangles for acute triangles.

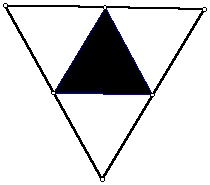
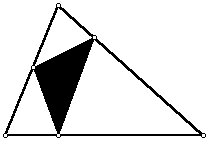
The ORTHIC triangle in each picture is colored black.As the triangle gets closer to a right triangle the ORTHIC triangle gets smaller.In the next picture we have a right triangle with the ORTHIC triangle drawn into the triangle.

 ORTHIC Triangle for Right Triangles

As you can see there is not a triangle drawn inside of the right triangle.There is only a blue point on the hypotenuse that represents the ORTHIC triangle.The ORTHIC triangle does not exist for right triangles.Next we will look at the ORTHIC triangle for obtuse triangles.

 ORTHIC Triangle for Obtuse Triangles

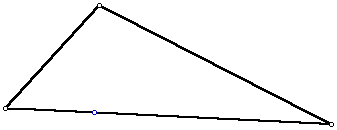
As you can see from this drawing, the ORTHIC triangle also does not exist for obtuse triangle.We also have a blue point on this drawing that represents the ORTHIC triangle.This point is on the side opposite the obtuse angle.

Therefore, now we have surmised from our investigations that the ORTHIC triangle only exists for acute triangles.Next we will look at the centroid ( G ), orthocenter ( H ), circumcenter ( C ), and incenter ( I ) for the ORTHIC triangle.
 
 

 Centers for an ORTHIC Triangle

 First lets look at the G, H, C, and I for the original triangle.

 Next lets look at the ORTHIC triangle drawn into the original triangle.

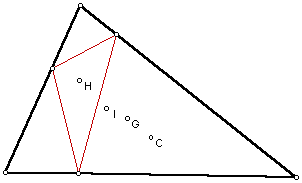
     The ORTHIC triangle is the red triangle drawn into the figure.Next we will look at the G, H, C, and I for the ORTHIC triangle.

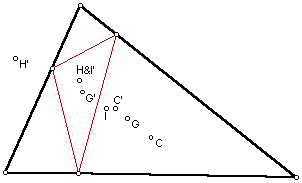
In the drawing, the ORTHIC triangle centers have the symbol (‘) on them.In the ORTHIC triangle the orthocenter (H’) and the crcumcenter (C’) is outside of the ORTHIC triangle because the ORTHIC triangle is an obtuse triangle.Any time you have an obtuse triangle the orthocenter and the circumcenter will be outside of the triangle.

The ORHTIC triangles incenter (I’) is the same as the original triangles orthocenter (H).Also, the ORTHIC triangles circumcenter (C’) is very close to the original triangles incenter (I).Therefore, we can see from the drawing that the ORTHIC triangle and its centers are very closely related to the original triangle.

Return