We know that if the original triangle is equilateral, then the triangle of its medians is also equilateral.

Question?: Will an isosceles original triangle generate an isosceles triangle of its medians?

My guess, before I explore, would be yes; but we need to use GSP to see if I'm correct in my assumption. First, I'm going to construct an isoceles triangle. Next, I will construct the triangle of its medians. Then, I'm going to measure to see if the lengths of the sides are equal to the medians. Let' see how this looks:

Well, I constructed the medians triangle to have one side the same as one of our original medians (blue), then constructed the other two sides parallel to their respective medians. By this construction, it is obvious we would still have an isosceles triangle. However, I measured just to make sure and, indeed, two sides and their corresponding angles are equal.

Question?: Will a right triangle always generate a right triangle of medians?

Again, let's use GSP to our advantage to find out what happens. First, I'm going to construct a right triangle. Then, I'm going to construct a triangle of its medians. I will use GSP to measure and see if the triangle of medians has a 90 degree angle. Let's see what happens:

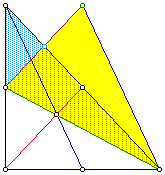
To show that the medians triangle of a right triangle does not always generate a right triangle, we need only to show a counterexample.We have done that. Clearly, the medians triangle (yellow) is not itself a right triangle, when constructed my original to be so. Just to check, I measured the angles of the medians triangle and the largest angle is 75 degrees.

Question?: What if the medians triangle is a right triangle?

Well, let's see how a graph that satisfies this question might look:

Well, I guess what this shows me is that for the medians triangle to be a right triangle the original does not need to be a right triangle. Here is a good example of that. While the medians triangle is a right triangle the original is obtuse.

Question?: Under what conditions will the original triangle and the medians triangle both be right triangles?

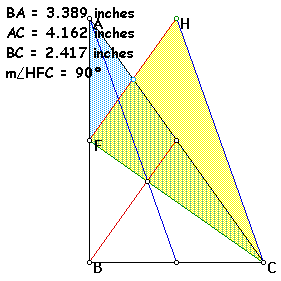
Here, we see a problem where both triangles are right triangles