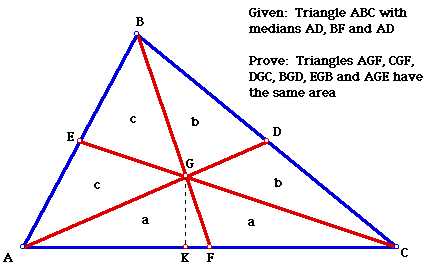
Theorem: The medians of a triangle separate that triangle into 6 smaller triangles of equal area.

Proof:

(Auxiliary line segment GK has been constructed perpendicular to side AC.) Since BF is a median of triangle ABC, F is the midpoint of side AC and AF = FC. Both triangles AGF and CGF share the same altitude GK. Since triangles AGF and CGF have the same base length and height, they have the same area. The variable a represents this area in the drawing above. Similar reasoning could be used to demonstrate that triangles DGC and BGD have the same area (represented by the variable b) as well as triangles EGB and AGE (area represented by c). We already know that triangles ABF and FBC have the same area (see theorem 1 on the previous page). This implies that a + 2c = a + 2b. This equation simplifies to c = b. Obviously, a = b and a = c could be demonstrated by similar arguments. Thus, triangles AGF, CGF, DGC, BGD, EGB and AGE have the same area.

(Reference: Coxeter, H. S. M. & Greitzer, S. L. (1967). Geometry Revisited. Washington D. C.: The Mathematical Association of America.)