Investigating centers of a Triangle
The Circumcenter
and the Centroid of a Triangle
Introduction
In this write-up, I examine some centers of a triangle; Thecircumcenter and the centroidof a triangle.
First, I construct the circumcenter and the centroid of a triangle using GSP. Then, I will explore each center's location for shapes of triangles and properties. Finally, I investigate the relation between the circumcenter and the centroid of a triangle.
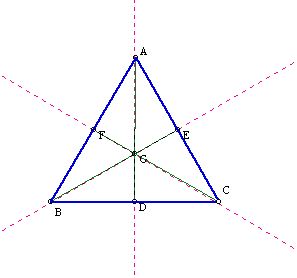
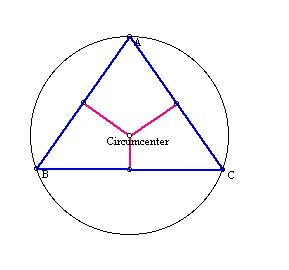
The circumcenter of a triangele is the intersection point of three perpednicular bisectors of each side in a triangle.

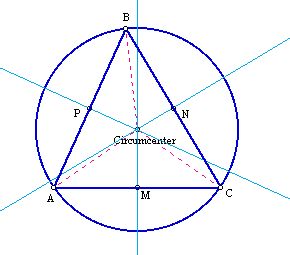
Since triangles APC and BPC are congruent(SAS), triangles BNC and DNC (SAS) and triangles AMC and DMC, AC=BC=DC.
So, any circle with C as center and passing through one of the vertices will go through all of them.
The circle is called circumscribed circle(circumcircle).
| Inside | On side | Outside | |
| Right triangle |
|
||
| Acute triangle |
|
||
| Obtuse triangle |
|
||
| Scalene triangle |
|
|
|
| Isosceles triangle |
|
|
|
| Equilateral triangle |
|
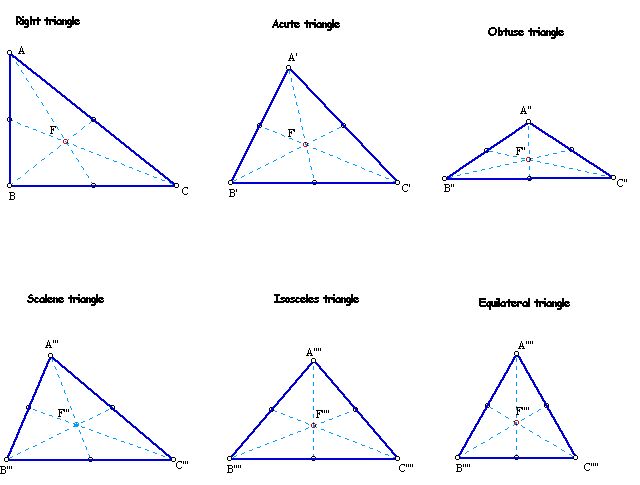
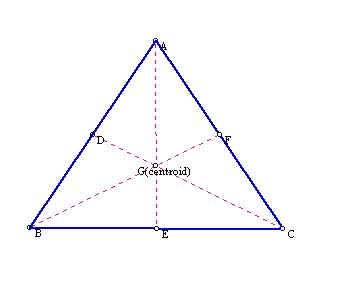
| Inside | Onside | Outside | |
| Right triangle | x | ||
| Acute triangle | x | ||
| Obtuse triangle | x | ||
| Scalene triangle | x | ||
| Isosceles triangle | x | ||
| Equilateral triangle | x |
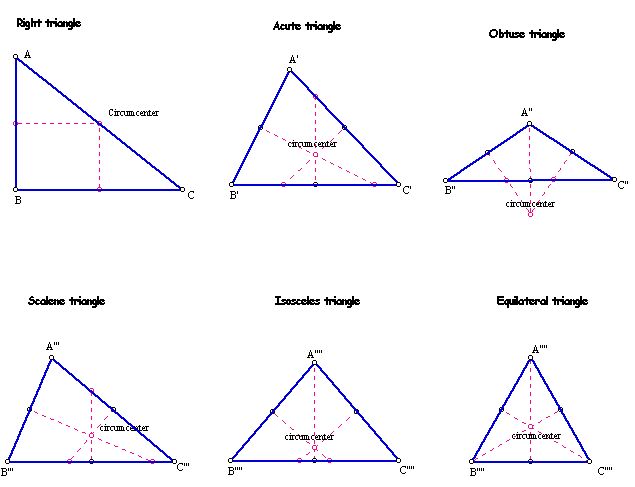
The interesting properties of the centroid
1) Distance
Centroid in a triangle divides each median into two parts,
the ratio whose lengths is 2:1.
AF : FE = BF : FG = CF : FD = 2:1
2) Area
The six areas of the interior triangles formed by the
three medians in the triangle are all the same.
area CEF= area GFC =area AFG= area = ADF= area FEB= area
DBF

For an equilateral triangel, the circumcenter and the centroid are the same point(G).
* perpendicular bisectors (pink dished line)
* medians (green line)