Explorations with Tangent Circles
This discussion is a summary of my explorations
with the basic idea of finding a circle tangent to two given circles.
Sounds simple enough right? Brother, you don't know the half of
it...
I am going to discuss this from a student's
discovery standpoint, as I myself hadn't done anything like this
before. I'm hoping to use my own experiences in working with this
problem to help me understand how something like this could be
used in the classroom. Here we go!
The first part of the assignment is
to construct a circle tangent to both given circles who are contained
within each other.

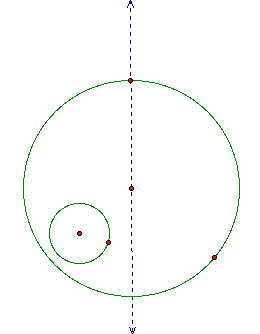
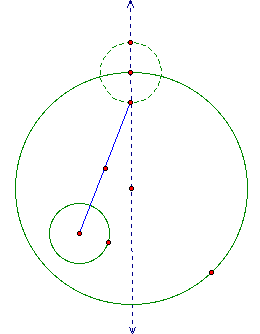
The construction of this circle comes
from using isosceles triangles. The idea is to locate the center
of the third circle. We know that the center of the third circle
has to lie on the line containing the center of the large circle
and the designated point of tangency on this circle.
We also know that the circles center
has to be equidistant from the point of tangency on the large
circle and a tangency point on the smaller circle. To find this,
we are going to construct an isosceles triangle and find the perpendicular
bisector of its base. We know from high school geometry that the
perpendicular bisector of an isosceles triangle is the collection
of all points equidistant from each endpoint of the segment. Finding
where the perpendicular bisector intersects the line through the
point of tangency will give us the location of the circle for
that particular location.
In order to make our triangle isosceles
we will construct a circle whose radius is equal to our smaller
circle and center it on the point of tangency on the large circle.
We find where this circle intersects the diameter of the circle
containing the tangency point, and use this point and the center
of the original smaller circle to form the base of our triangle.
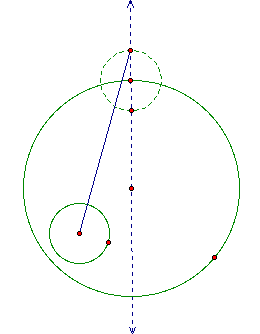
Then we construct the perpendicular
bisector of this segment. Where it intersects the line is the
center of our circle (A).
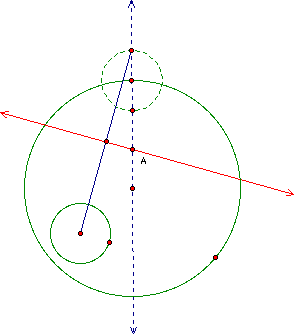
And, we have our circle.
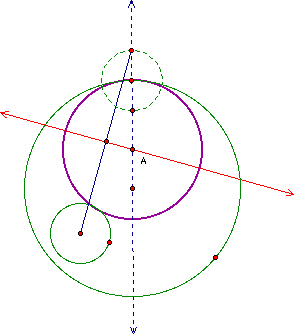
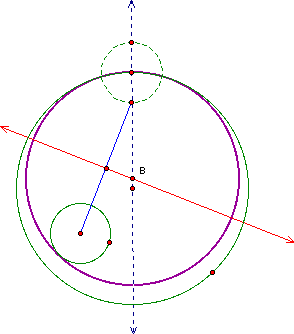
Having been given the information to
accomplish this construction, we were asked to construct the purple
circle such that the smaller circle is inside of it. Here is where
the exploring comes into play. We need the tangent point on the
small circle to be on the far side of it. How can we move our
circle the equivalent of one diameter of the small circle? Hmm...
We know the basic idea is to form an
isosceles triangle, but this time, we need the base of our triangle
to end not at the far edge of the circle, but on the closer intersection
point. This will accomplish our "one diameter closer"
idea.
Now when we find the perpendicular bisector,
and its intersection, we should have exactly what we need.
But constructing these circles is only
the first part of the exploration. More interesting is to look
at the collection of all of these circles. We have found the center
of one of the circles, but by moving the point of tangency on
the large circle, we can see all of the possible locations of
the center of the purple circle. This collection of points is
known as the locus of points for the center. We can use
GSP to show the path the center takes.
If we look at the locus of points where
the purple circle is tangent between the two green circles, we
find this path, colored blue.

We can see that the path formed is an
ellipse. The foci of the ellipse appears to be the centers of
the two circles. Interesting...
If we look at the locus for the case
where the purple circle is around the smaller circle, we see the
following.

Once again the locus of points forms
an ellipse, also appearing to have the center of the circles as
its foci. You may notice that the first ellipse is more round
than the second. This measure of roundness, or more correctly
ovalness, is known as the eccentricity of the ellipse. It is determined by the distance
from the center of the ellipse to one of the foci (c), and the
distance from the center to one of the vertices (a) . The ratio
of c / a is always between 0 and 1. The closer the ratio is to
0, the more circular the ellipse is, the closer the ratio is to
1, the more elongated the ellipse is.
In both cases the eccentricity is a
function not only of the size of the circles, but also their location
within the original circle. For the case where the smaller circle
is contained within the purple circle, the locus of points will
remain an ellipse with foci at the centers of each green circle,
as long as the two circles remain inside of the other and do not
intersect. Once the green circles intersect, the ellipses are
replaced by...

a hyperbola!
Notice here that the centers of the
circles are still foci, however, for a hyperbola instead of the
ellipse.
In the case where the purple circle
is between the green circles, intersection does not change the
general shape of the locus. In fact it isn't until the circles
are completely disjoint that the locus becomes hyperbolic.
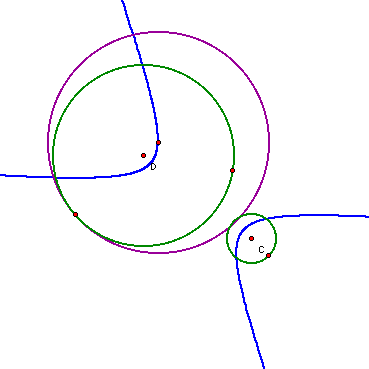
Once again, we see that the centers
of the circles are the foci for the hyperbola.
We see what happens when the circles
are completely on the interior and when they are completely disjoint.
what happens when the circles are tangent to each other?

It appears that as the circles are tangent,
that the locus of points appears to collapse into a line segment
connecting the two centers of the green circles.
Let's continue our exploration with
these two circles. Now lets change the location of the smaller
circle and see how it effects the shape of the locus.

Notice please, that as we have moved
the circle farther away, the less severe the curve of the hyperbola
is. As we get closer, the curve becomes more severe.
Now lets look at the second case, where
the purple circle is constructed to encircle the smaller circle.
The severity of the curve changes as the circles move farther
apart. That is until, the circles are disjoint. At this point,
the hyperbola does not appear to change.
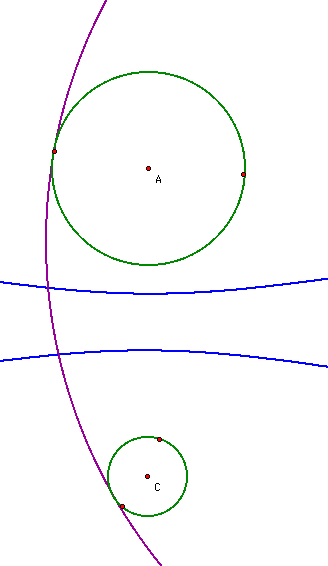
However, once the circles were disjoint,
I decided to start changing the size of the circles to see how
that might effect the locus. I found something I thought was interesting.
The larger that circle C became, the
closer the parts of the hyperbola became.
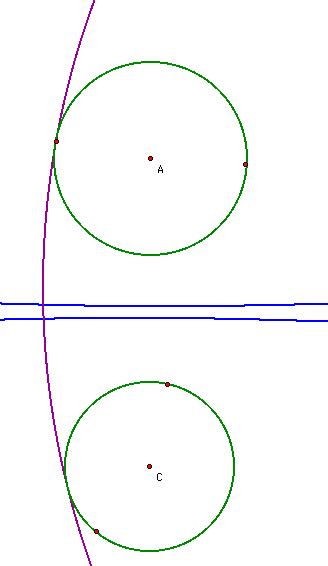
This continued to happen until...
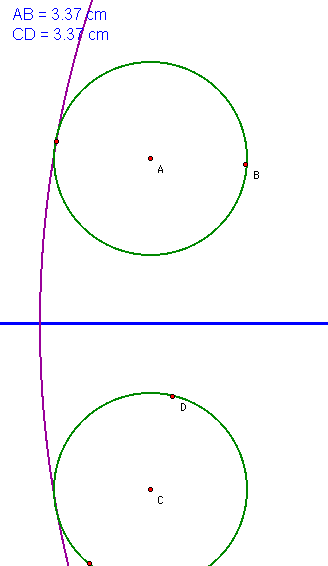
The hyperbola collapsed into a line.
I am hypothesizing that this is true when the circles are congruent.
Which I show by measuring the radii of each circle above.
Then, I wanted to see what would happen
if I moved the circles back towards each other until the circles
again intersected.
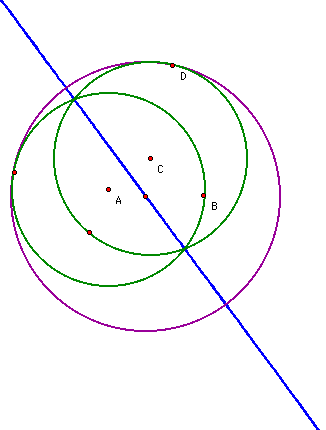
The line remained! Notice also
that it went though the points of intersection of the two circles!
What would happen if I made the two
green circles concentric?
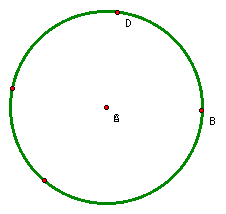
All three circles are congruent, and
the locus of points for the purple circle is now only one point,
the center of the two green circles. Cool!
I see this approach to the assignment
as beneficial to a high school student in the following ways.
1. As a way to be introduced to the
concept of locus of points.
2. As a means of reviewing constructions
or the concept of tangency.
3. As a way of showing relationships
between all of the different conic sections.
If any or all of these ideas are goals
of a lesson, I recommend using a GSP exploration as a means to
accomplish them.


















