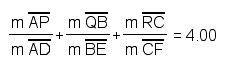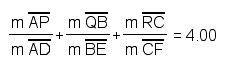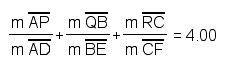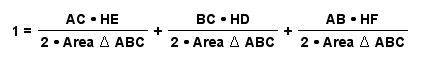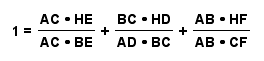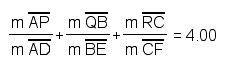Altitudes and Orthocenters
In this assignment, I worked with Lauren Wright in exploring
the altitudes and orthocenter of an acute triangle. What began
as a simple "ask your office neighbor for some help"
on her part, turned into an obsessive need to find an answer to
a geometric proof on my part. And, since I figured I was going
to finish the proof anyway, I decided I would write about it in
my writeup of assignment 8 as well.
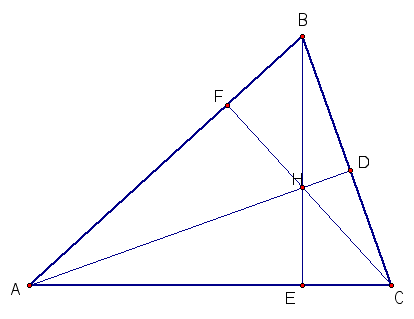
Our story begins with a simple acute triangle. We construct
each of the altitudes for the sides of the triangle, and label
their intersection point the orthocenter.
Our proof is about this equation and its result: 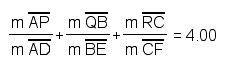
I used GSP to explore this relationship and found that it
holds for a variety of acute triangles. But, as we all know, a
GSP demonstration is not a proof, so I guess we should write one.
First, it would be a good idea to make some triangles and
to draw the circumcircle of our triangle. We will also label the
points of intersection from our altitudes and the circle.
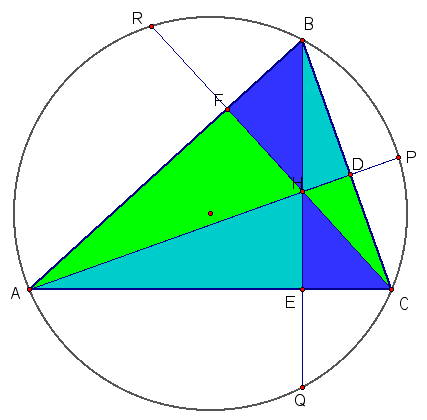
Lets begin our proof by building some relationships between
triangles. The triangles which are colored alike can be shown
to be similar. Each pair of triangles contains a right angle from
the altitude, and a second angle pair will be congruent by vertical
angles. This means triangles AFH and CDH, triangles AEH and BDH,
and triangles HFB and HEC are all similar by AA similarity. We
can use the definition of similarity to conclude that all three
angles of all pairs of triangles are congruent.
This tells us something about angles, but nothing about sides,
so we are going to draw some construction lines and look for more
triangle relationships.
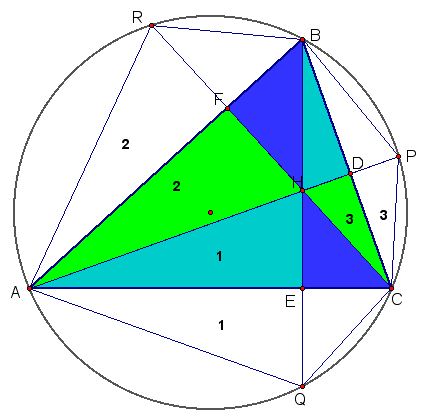
We can show that each pair of numbered triangles are congruent
by ASA. Let me break it down for you...
Let's begin with triangles AHE and AQE. We know that they
share segment AE, and that they both contain right angles at E.
To show that angles HAE and QAE are congruent, we need to be a
little sneaky.
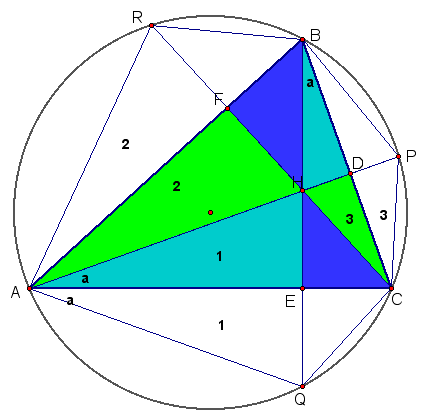
As you can see in the diagram, the angles labeled as "a"
will be congruent. We have already shown that HAE is congruent
to HBD from the similar triangles. Now we will use the fact that
QBC and QAC both subtend the same arc of the circle. Since this
is true, those angles must be congruent. By transitivity, we can
state that HAE and QAE are congruent, thus giving us the triangles
congruent by ASA.
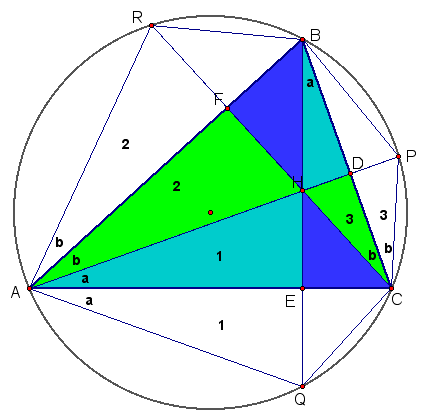
Using similar arguments, we can show the other pairs of triangles
congruent as well. In these cases, the angles marked as "b"
will all be congruent by the same combination of similarity of
triangles, inscribed angles subtending the same arc, and the transitive
property.
So, what is the point of all of this? It is all prelude to
the main event, but necessary so we can state one crucial fact.
That is that since the pairs of triangles are congruent, the definition
of congruency tells us that the corresponding parts are all congruent.
In this case, what we need is that:
HE = EQ, HD = DP, and HF = FR
Now we can get back to our original goal, proving that 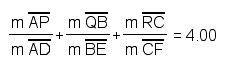
We begin by using segment addition to rewrite some
of our values, namely:
AP = AD + DP
QB = BE + EQ
RC = RF + FC
Now we use substitution from our above equalities
to write:
AP = AD + DP
QB = BE + EH
RC = CF + FH
Which means our new fractions look like this:

Which means our original equation becomes

We want this to equal 4, so we can infer that we need
to prove that

In order to do this we are going to need to approach it a
bit differently than just manipulating fractions. We will use
what we know about area of these triangles to prove this statement.
We begin by recoloring our triangles to show that the area of
the three small triangles will equal the area of triangle ABC.

We know that the area of a triangle equals 1/2 bh. And we
know that since areas of polygons are additive, we can write the
following equations:

so

If we multiply both sides by 2 to eliminate fractions,
we see that
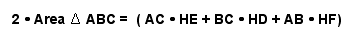
We want this to equal 1, so we divide both sides by
2 times the area of ABC
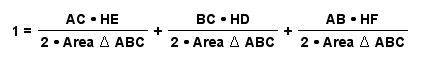
Now, we know that the area of triangle ABC can be
calculated by using 1/2 bh, so there are three different ways
we can express the area, and more specifically, two times the
area.
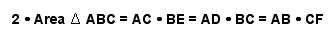
So, lets use substitution into the above fractions,
using the appropriate value of the area of triangle ABC to ensure
our fractions become what we desire.
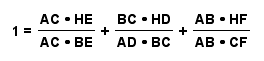
You should notice that in each fraction, we will be
able to reduce by a common factor, this leaves us with:

Which, is exactly what we needed to show. Therefore,
we can conclude that for all acute triangles: