The Pedal Triangle
and the Sim(p)son Line

Homer Simpson creates the Simson
line - Look mom! No ruler!
Okay, so we are not going to talk about
Homer's contributions to mathematics. But in a topic almost as
risky as when Homer became the "Beer Baron", we will
be discussing explorations with the pedal triangle and the true
Simson line.
Lets begin with the construction
of the pedal triangle. For any triangle ABC , the pedal triangle
is formed by first choosing the pedal point, an arbitrary point
P in the same plane as ABC. Then, construct the perpendiculars
from P to each of the sides of triangle ABC. (You may need to
extend the segments of the triangle into lines.) The pedal triangle
is formed by connecting the three points where the perpendicular
lines intersect each side of the triangle, in the case below,
R,S,T.

One of the amazingly cool
things about pedal triangles, is that as you move the pedal point,
your triangle changes as well. Click
here to open a GSP file where you can manipulate the location
of the pedal point above.
One of the things you may
have noticed as you moved the pedal point of the triangle is at
times, the pedal triangle seems to compress, or degenerate, into
a line segment. This segment is a part of the famous "Simson
Line".

There are various locations
in which the pedal point can be to form the Simson line for a
given triangle. The question is, what is the set of all of these
points? Some places that are usually discovered for the degenerate
triangle are at each of the vertices of the triangle. Hmmmm, what
set of points do we know that contain all of the vertices of the
triangle? How about the circumcircle of the triangle? Lets construct
it and see.

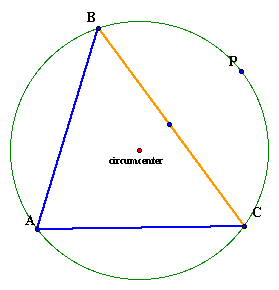
As you can see, when P is
on the circumcircle of triangle ABC, the pedal triangle becomes
the Simson line. Click here for
a GSP file to see the animation of the pedal point.
As you watched the animation,
you might have noticed that there are times when the Simson line
coincided with one of the sides of triangle ABC.
What is it about these
points on the circle that allow this to happen? Let's explore
a bit and find out...
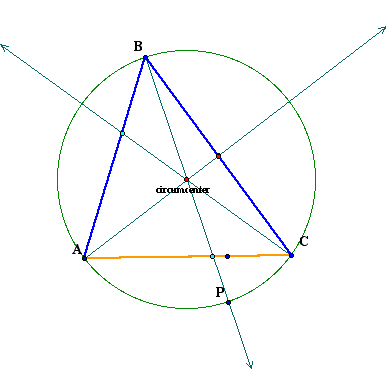
It appears that there is only
one point for each side of the triangle, that can produce the
simson line on that segment. That point is located somewhere on
the arc of the circumcircle cut off by that side of the triangle.
In the case above, for the simson line to be segment BC, P must
be in a particular place on arc BC. It appears that when P is
in the above location, that it lies on the ray containing A and
the circumcenter of the triangle. Lets construct that ray and
check our hypothesis.

It does indeed appear
to be on that ray. Let's check the pedal point on the other two
rays as well.

Okay, so we now know that when the pedal point
coincides with the point of intersection of the ray containing
the opposite vertex and the circumcenter, and the circumcircle
of the triangle, that the simson line will be the side of the
triangle opposite of the vertex. There must be a simpler way to
describe these points, but to my knowledge, these rays do not
have a special name such as median, or angle bisector. They do
contain "cevians", which are segments drawn from a vertex
of a triangle to any point on the opposite side.
The term cevian, was coined by an
Italian mathematician, Giovanni Ceva, who proved a very cool theorem
known today as Ceva's theorem. The theorem states the following:

Since we drew each of these rays through the
same point, the circumcenter, we know that the three rays are
concurrent. This fact would allow us to state that for our given
triangle, the above ratios would hold. Of course, this would imply
we could prove Ceva's theorem. Click
here for a link to a proof of Ceva's theorem.









