

This discussion is based on the following picture with A the center of the red tangent circle, B the center of the blue tangent circle and C the arbitrary point used to construct the circles.
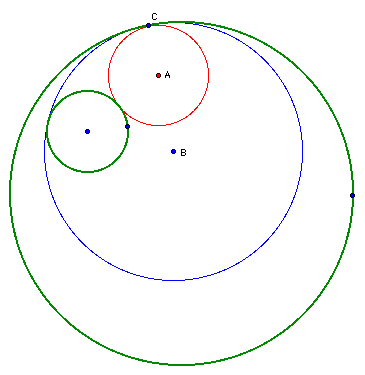
Now, we want to figure out what happens to A and B when C moves around the circle. Using GSP, there are two differnt ways to look at this. First, one can use the animation feature and animate C around the circle while tracing the points A and B. This is the method That I am going to use. The second is to use the locus feature of GSP. Either way, one will get the same picture. I choose the animate feature because it allows one to see the locus being constucted. The locus command just creates the locus without the user seeing where it came from. So, based on other constuctructions, one would expect the loci to be an ellipse. Which as can be seen below, the loci are ellipses.
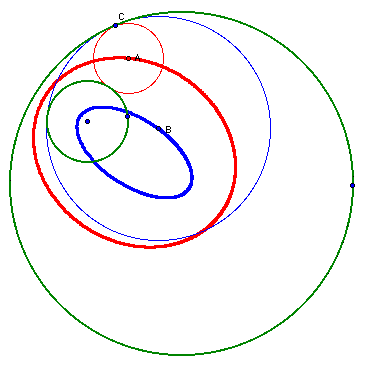
What happens if the circles intersect? Would one expect the loci to remain ellipses? Here, the locus of A does remain an ellipse but the locus of B changes into an hyperbola which can be seen below.
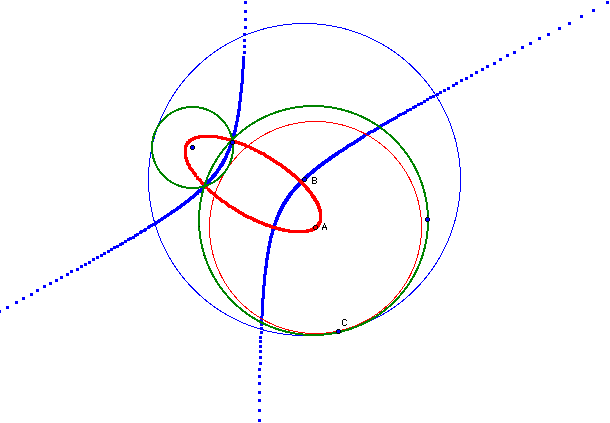
Now, what happens if the original circles are disjoint? Will we get two ellipses? An ellipse and a hyperbola? Or something else? In this case, one should get two hyperbolas. A picture of this is below.

One other loci that I found interesting are the midpoints of the segments used to make the perpendiculars that found the center of the tangent circles. These points are now marked D and E. I expected their loci to work the same fashion as the points A and B. To start with two ellipses, then get an ellipse and an hyperbola, and finally to have two hyperbolas. When I found these loce, it was surprising to find out they are circles in all three of the different situtations. An example of this is below.

Click here to link to a GSP sketch that will allow one to manipulate the circle and see all of the different loci.