

Let's begin by contructing the centroid of a triangle.
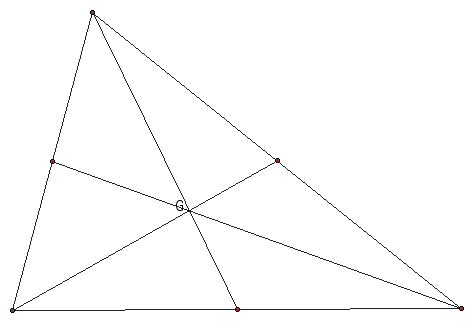
The centroid (G) of a triangle is the common intersection of the three medians of a triangle. A median of a triangle is the segment from a vertex to the midpoint of the opposite side.
The median divides the triangle into two areas. When we construct all three medians, we end up with six internal triangles.
Now, let's find the orthocenter of a triangle.
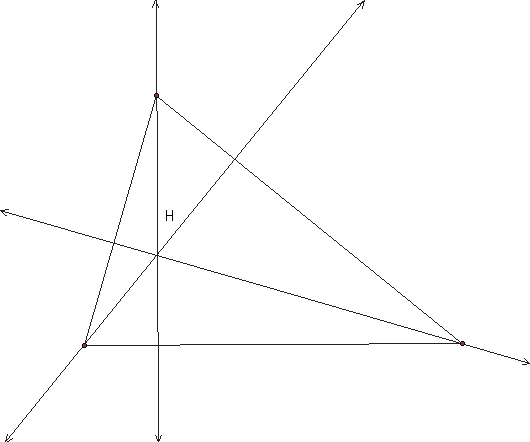
The orthocenter (H) of a triangle is the common intersection of the three lines containing the altitudes. An altitude is a perpendicular segment from a vertex to the line of the opposite side.
Let's construct the circumcenter of a triangle.
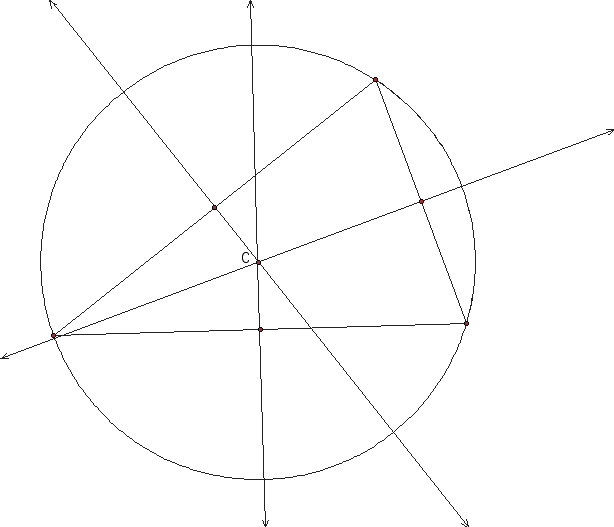
The circumcenter (C) of a triangle is the point in the plane equidistant from the three vertices of the triangle. Since a point equidistant from two points lies on the perpendicular bisector of the segment determined by two points, (C) is on the the perpendicular bisector of each side of the triangle. Note (C) may be outside the triangle.
Let's look at the incenter of a triangle.
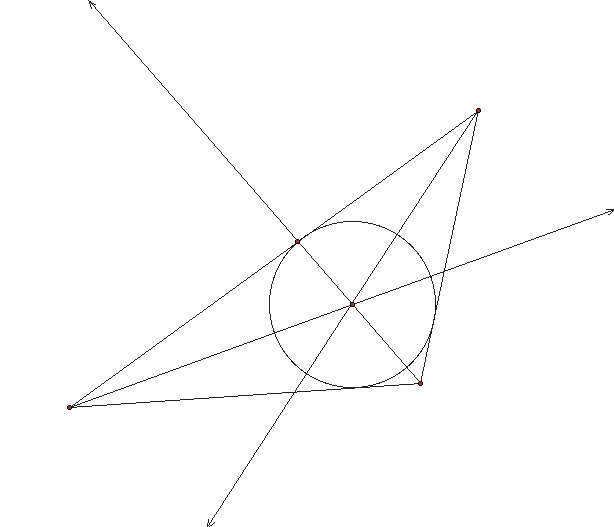
The incenter (I) of the triangle is the point on the interior of the triangle that is equidistant from all sides. Since a point interior to an angle that is equidistant from the two sides lies on the angle bisector, then (I) must be on the angle bisector of each angle of the triangle.
Now, let's see if there is a relationship between the centroid, the orthocenter, the circumcenter, and the incenter of a triangle.

When G,H,C, and I are constructed together, we can see that H,G, and C form a line. When the triangle changes shape they still remain in a line.
What if we look at the centroid, orthocenter, circumcenter, and incenter of the medial triangle and compare it to the original triangle.
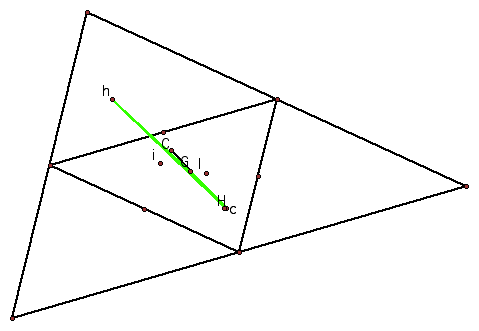
The medial triangle was drawn by connecting the midpoints of the original triangle. H,C,G, and I were constructed on the medial triangle, and h,c,g, and i were constructed on the original triangle. G and g were found to be the same point meaning that the centroid is the same for the original triangle and the medial triangle.
What if we look at the orthic triangle and find the centroid, orthocenter, circumcenter, and incenter? Is there a relationship between those of the orthic triangle and the original triangle?
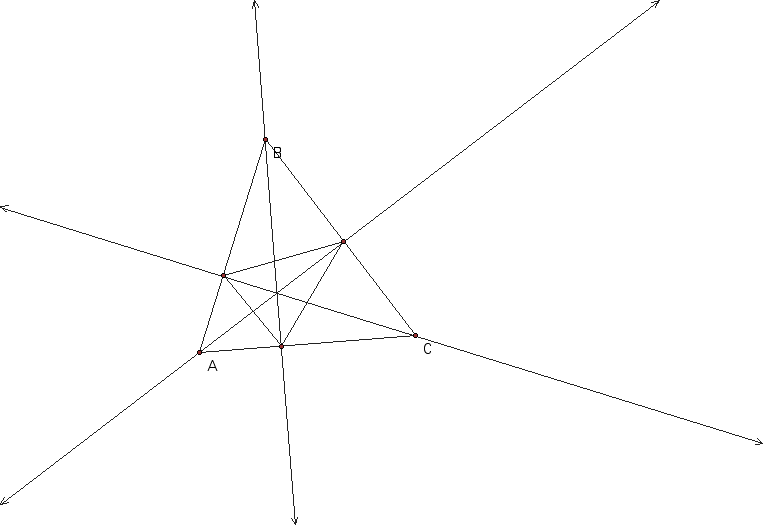
This drawing is of the orthic triangle. It was constructed by connecting the feet of the altitudes of the original triangle. Let's look at G,H,C, and I for the orthic triangle and conpare it to the original triangle.
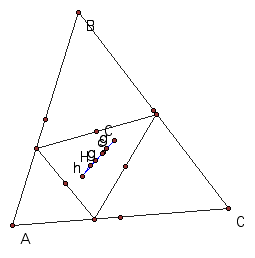
The H,G,C, and I all appear to stay in the same straight line in the orthic triangle and the original triangle. What if we change the triangle to a right triangle and an obtuse triangle. Let's look and see.
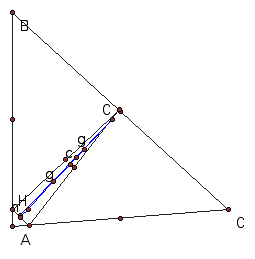
For the right right triangle, it appears that the points stay in their same straight line dividing the triangle into two equal areas.
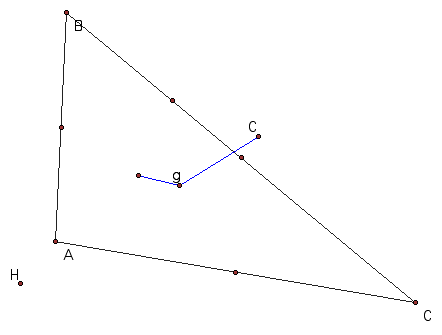
For the obtuse triangle, our orthic triangle is gone. The circumcenter has moved outside the triangle, and the orthocenter has moved outside the triangle. The points no longer appear to be in a straight line. The labels seem confusing, so I will construct the points again on this triangle.
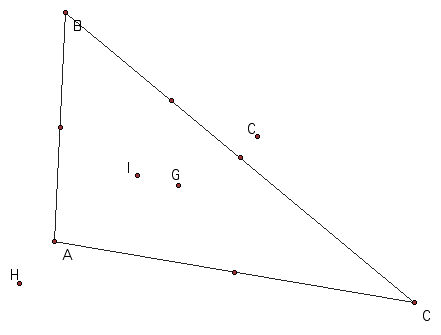
The points were correct, so I can assume that H, G, and C always remain in a line; however, the incenter does not appear on that line.
Now let's construct the the three secondary triangles, the medial triangle, the orthic triangle, and the triangle around the orthocenter.

Take the acute triangle ABC. Triangle GHI is the medial triangle, and triangle DEF is a triangle constructed by drawing HA, HB, and HC and constructing a triangle by connecting their midpoints. How can we prove that triangle DEF is similar to triangle ABC and congruent to triangle GHI?
First let's find the G,H,C, and I for each triangle.
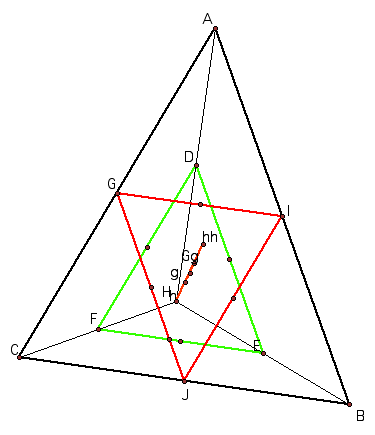
The G,H, and C stayed in the same line in all three triangles. Many of the points were the same for two of the triangles.
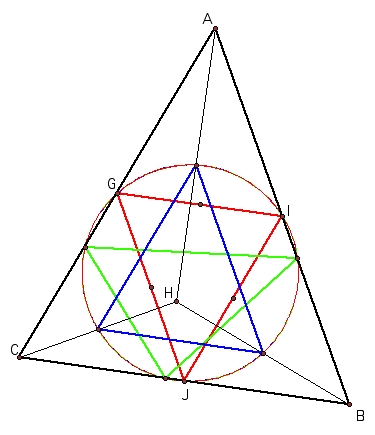
When the circumcircle of each of the three secondary triangles is constructed, they are the same circle. The red triangle was constructed by connecting the midpoints of the sides of the original triangle. The blue triangle was constructed by finding the orthocenter of the original triangle, connecting the orthocenter to the three vertices, finding the midpoint of each side, and then connecting those three midpoints. The circumcircle was constructed around each of the three secondary triangles, and all three circumcircles were the same circle which is difficult to see on one construction.
Let's look at the nine point circle. It is constructed through the three midpoints of the sides, the three feet of the altitudes, and the three midpoints of the segments connecting the vertices to the orthocenter. (N) is the center of the nine point circle.
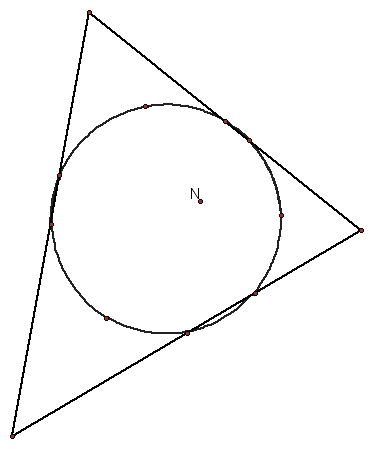
Let's see how (N) is related to (G), (H), (C), and (I).
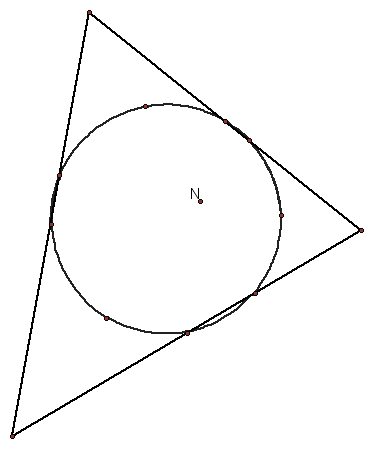
It appears as though (N) is on the same line as (G),(H), (C), and (I). Let's see if it still on the same line when the triangle changes shape.
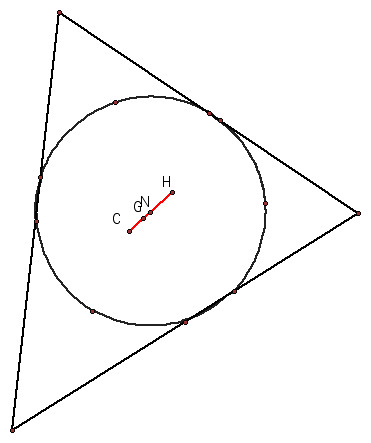
The center of the nine point circle remains on the same line as the orthocenter, circumcenter, and centroid of the circle.
Let's look at the three perpendicular bisectors of a triangle.
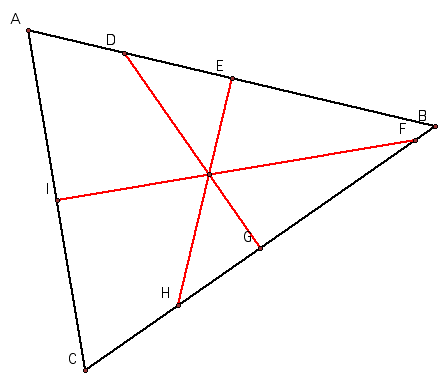
DG, EH, and IF are perpendicular bisectors. The perpendicular bisectors of a triangle are perpendicular lines through the midpoints of each side.
Let's look at the three altitudes of a triangle.
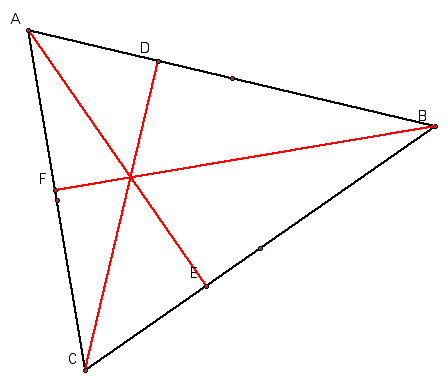
DC, FB, and EA are the three altitudes of the triangle. The three altitudes of a triangle are perpendicular lines from the verteces to the opposite sides.
Let's look at the three medians.
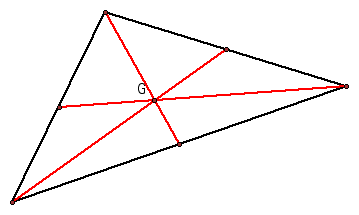
The three medians are lines connecting the midpoints of the sides to the opposite vertex.
Now, let's look at the three angle bisectors.
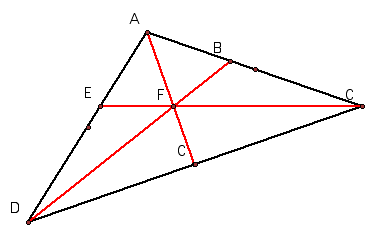
Can we prove that the three angle bisectors of the internal angles of a triangle are concurrent?
CE is an angle bisector of angle ACD, and DE is an angle bisector of angle CDA. Because point F is on both angle bisectors and is equidistant from each side of the triangle, it is also on AC which is the angle bisector of angle DAC. Since the angle bisector is equidistant from all three sides, it would have to be the same point. Therefore the three angle bisectors of a triangle are concurrent.
Take the point of concurrence and construct a circle tangent to all three lines.

We can see that this circle tangent to all three sides has points of tangency at the intersection of each angle bisector and the opposite side.