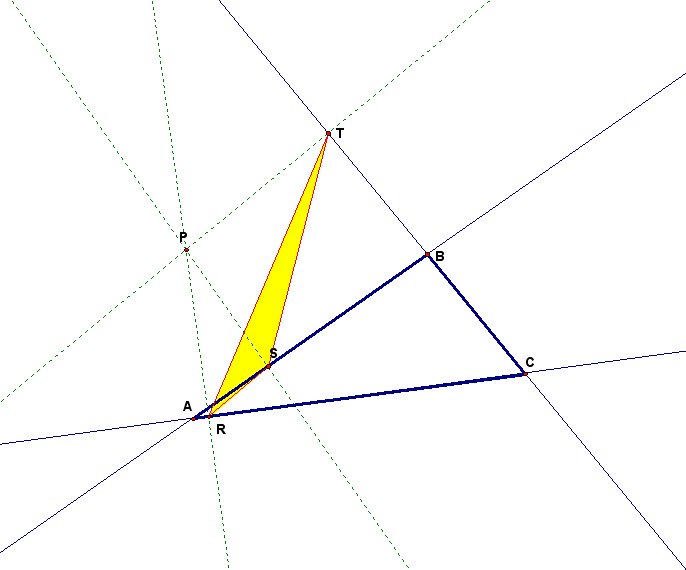
For any given Triangle ABC, we can construct the Pedal Triangle for any arbitrary point in the plan, called the Pedal Point by constructing the perpindiculars from the pedal point to each of the sides of Triangle ABC (sides extended if necessary), and finding the intersections, say R, S, and T. Then Triangle RST is the pedal triangle for this particular pedal point. See the following illustration.

Click here for GSP tool for constructing pedal triangles.

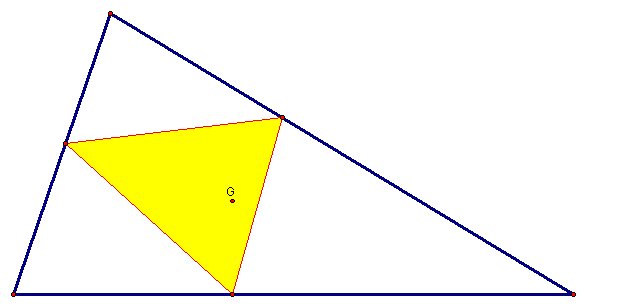
Now let us observe some special cases of the pedal point. We shall use our familiar triangle center. First we have the pedal triangle when the centriod of our given triangle is the pedal point.

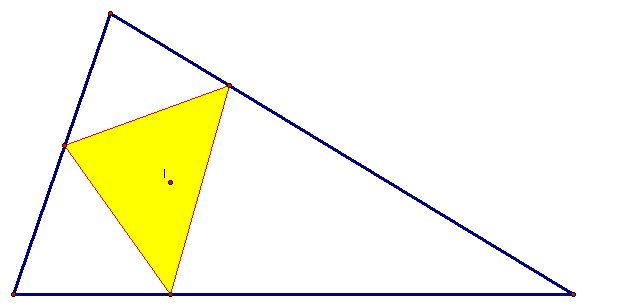
Next we use the incenter of our given triangle to obtain the following pedal triangle.

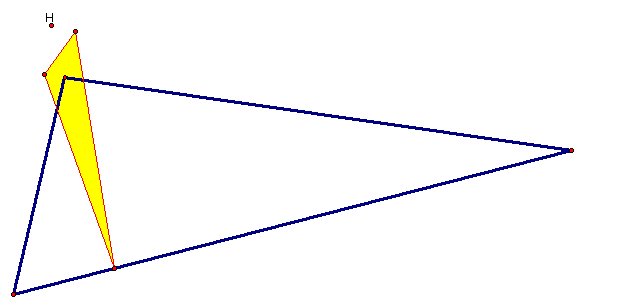
Let's select the orthocenter to be our pedal point. We have seen previously that the orthocenter does not necessarily have to be inside of our triangle. First we have the case where the orthocenter is contained in our triangle.

We also have the case where the orthocenter is outside our given triangle.

As with the orthocenter, we have seen that the circumcenter need not be contained in our triangle. Here is an example of the pedal triangle when the circumcenter is the pedal point and is inside our given triangle.

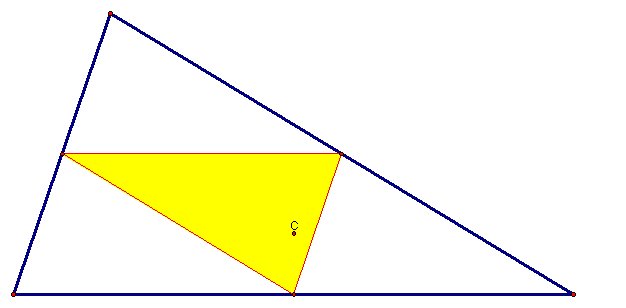
An example of the pedal triangle when the circumcenter is the pedal point and is outside the given triangle.

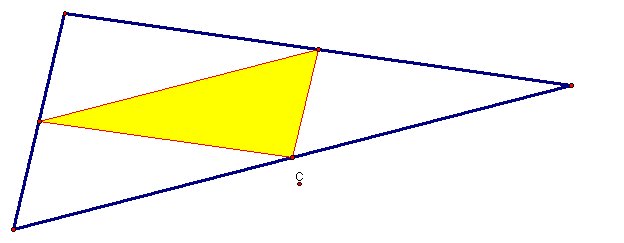
Our last triangle center is the center of the nine-point circle. Here is an illustration of the pedal triangle when the center of the nine-point circle is the pedal point.

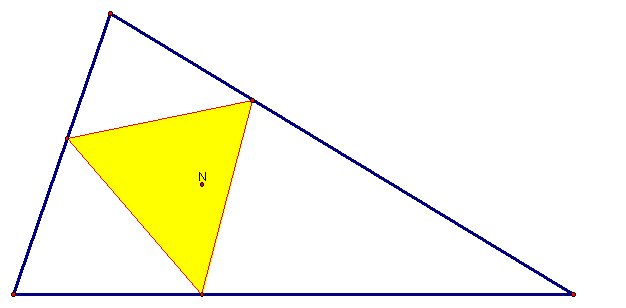
The following is an example when the pedal point is on a side of the given triangle.

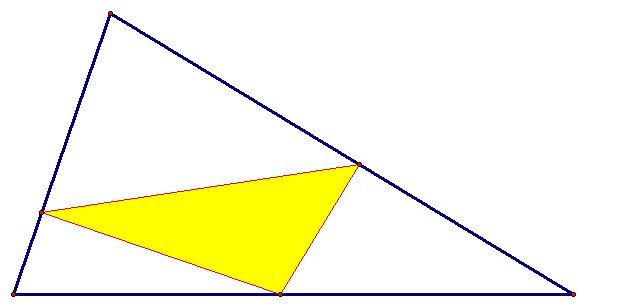
There is an interesting case of the pedal triangle when the pedal point lies on the circumcircle of the given triangle. In this case, all of the points on the pedal triangle are colinear. This is called the Simson Line.

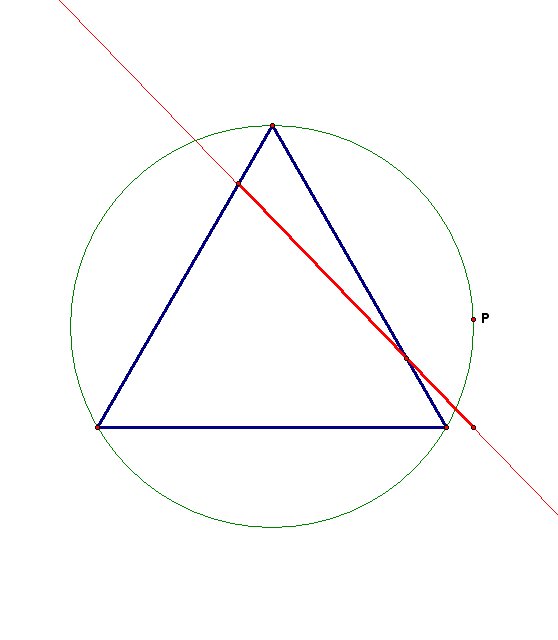
Here is a trace of the Simson Line as the pedal point is moved along the circumcircle of the given triangle.

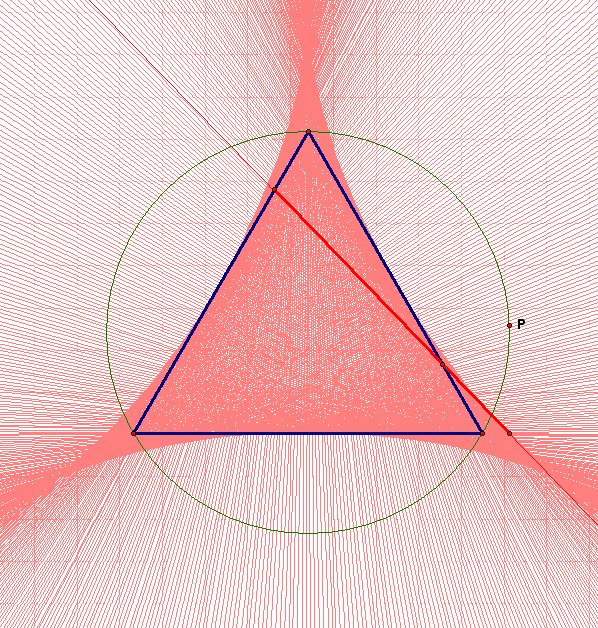
We close with two interesting traces of the sides of pedal triangles. First we have the trace of the sides of the pedal triangle when the pedal point is on a circle whose center is the circumcenter of the given triangle, but whose radius is smaller than the radius of the circumcircle. The result is the following.

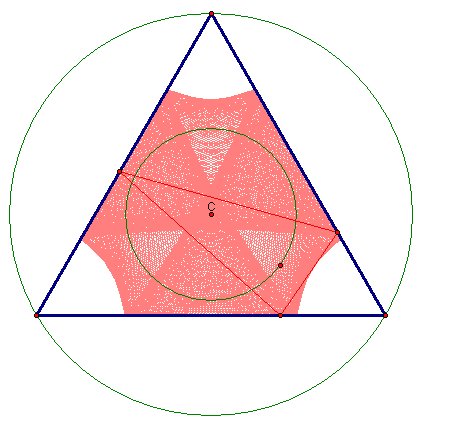
Here we have the trace of the sides of the pedal triangle when the pedal point is on a circle whose center is the circumcenter of the given triangle, but whose radius is larger than the radius of the circumcircle. The result is the following.