Assignment 11 Writeup

Polar Equations

 

Let's investigate the polar equation below:

 

 

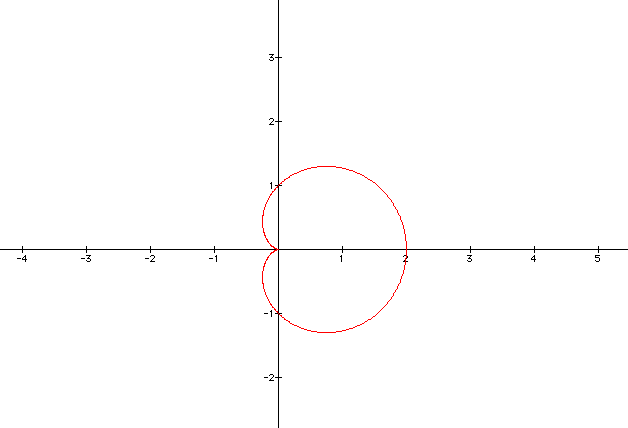
Here is the graph of this polar equation where a,b, and k are all equal 1 and theta is between 0 and 2 pi.

 

Now, let's keep a and b equal to 1 and alter values of k. The following illustration in blue shows the graph of this function. Notice how with k= 2 there are two leaves on the graph. These leaves cross the x-axis at the origin (0,0) and at the points (2,0) and (-2,0).

 

 

Now, again let's keep a and b = 1 but change k to a value of 3. Notice now there are three leaves in the graph of the polar function. Notice how the three leaves intersect at the origin (0,0).

 

 

Here, with k=10, we have 10 leaves. It appears that k alters the number of leaves that the polar function has.

 

There are lots of leaves on the polar function when k=100. These functions make very unusual and pretty flowers!

 

 

So, when a and b are equal, and k is an integer, the function forms an "n-leaf rose".

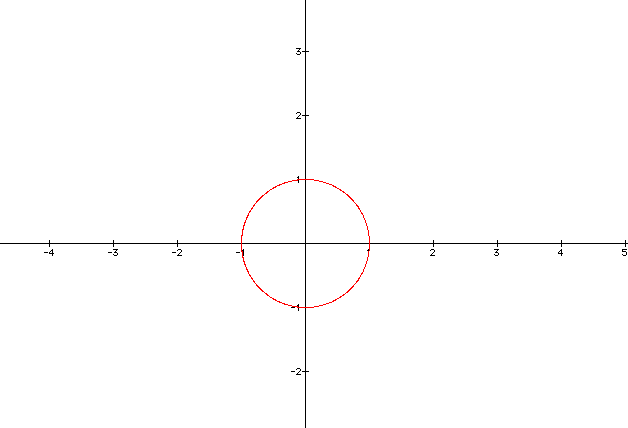
Now, let's take away the value in the polar function of a and look at the new function when k=0 and b = 1. This is just the graph of the cosine function on the polar coordinate map. Notice that at the origin, the cosine function is equal to 1. Notice, there is only one leaf present.

 

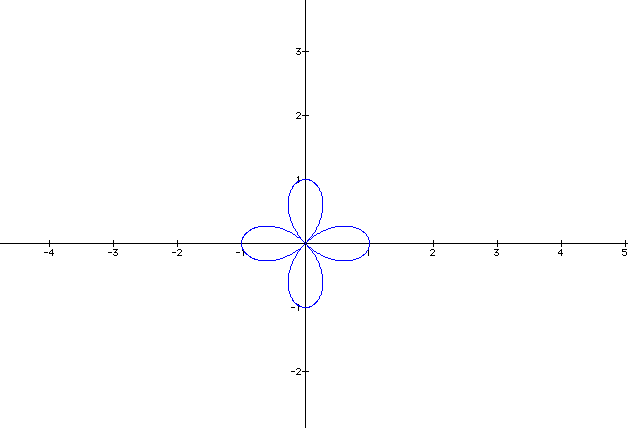
Now, for k=2 and b still at 1, the function has 4 leaves. It appears that taking the a value out of the equation causes the number of leaves present on the graph to be 2k. Let's see if this is true in other cases.

 

 

In the graph below, where k=3, I would expect there to be 6 leaves or 2k=2(3). However, this isn't the case. Maybe the leaves being equal to 2k only works when k is even. Maybe when k is odd, the number of leaves present is just k itself. Here, k=3 where k is odd and the number of leaves is just k itself, or 3.

 

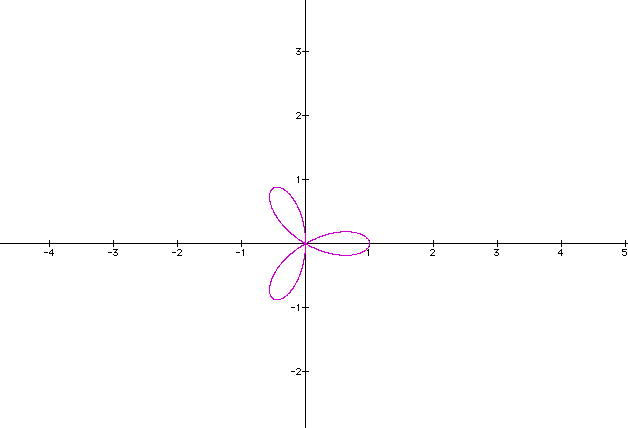
My intuition looks to be true because here, when k=10 and k is even, there are 20 leaves.

 

But, below, where k=13 and k is odd, there are exactly 13 leaves in the graph of the polar function.

 

 

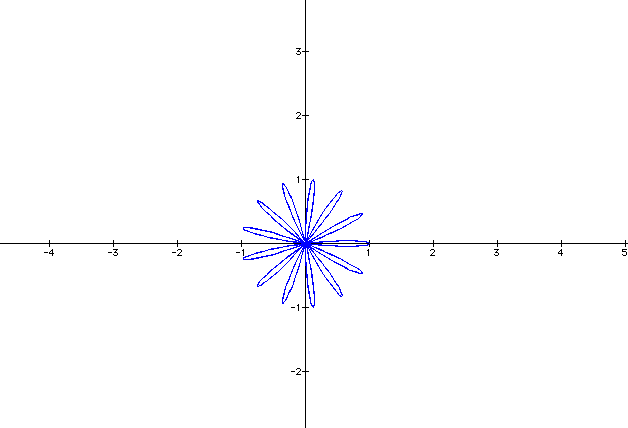
Finally, here, we have k=2 so we will expect there to be 4 leaves. However, I altered b here from 1 to 2. What effect does that have on the graph of the function?

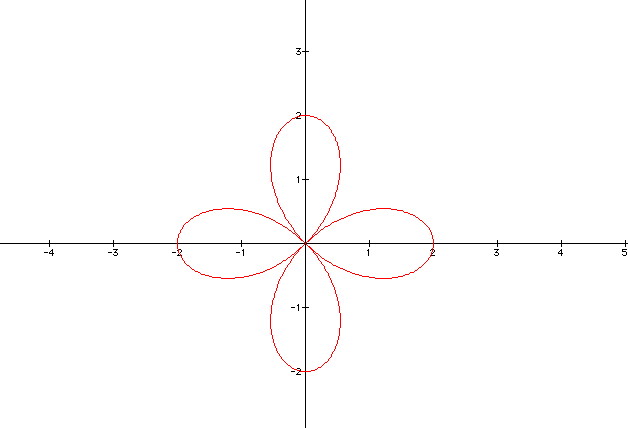
It appears that the value of b being change from 1 to 2 altered where the tips of the leaves intersected the axis. When b was 1, the leaves intersected the axis at the origin and the points (1,0),(0,1),(-1,0), and (0,-1). Now, that b equals 2, the leaves intersect the axis at the origin and the points twice that when b =1. So, the leaves intersect the axis at the points (2,0),(0,2),(-2,0), and (0,-2).

 

Here, when we have k=3 where k is odd, we will only have 3 leaves on the graph of the function. Since b is 4, we would expect the graph to be stretched out 4 times that when b was equal to 1. Here, it looks as though the tips of the three leaves end around the point 4 in different quadrants. When b was equal to 1 and k was equal to 3 earlier, the tips of the three leaves ended arounded the point 1.

 

Now, what happens if we try graphing the function . Here, I replaced cosine with sine. Let's see how this alters the graph of the polar function.

This graph only has one leaf when k=1 and b=1.

 

Here when k=2, let's see if the number of leaves produced will be 2k as with the cosine function. This, in fact, does look to be the case. K=2 and there are 2k=2(2)=4 leaves on the graph. Go back up the page and look at the cosine function when b=1 and k=2. It seems as if the x and the y-axis bisect the leaves in that graph. However, here it appears that the lines y=x and y=-x bisect the leaves of this graph. It doesn't appear that the points intersect at the origin as the cosine function did. It appears that there is an open area in that region.

 

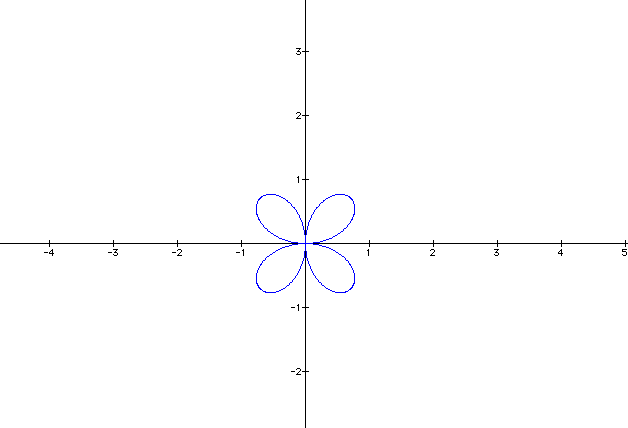
Now, k is equal to 5 and is odd. Let's see if the number of leaves will be equal to 5 instead of 2k as it was with the cosine function. It is true! There are only 6 leaves on this graph. The leaves are contained in the area from -1 to 1 along the x and y-axis.

 

 

Here, since k is equal to 6 and is even, there should be 12 leaves. Again, this is the case so it looks like that the number of leaves = 2k when k is even and = k when k is odd. This is the same as it was with the cosine function.

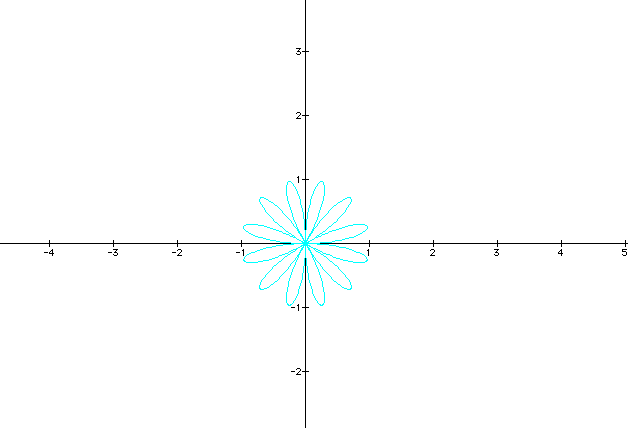
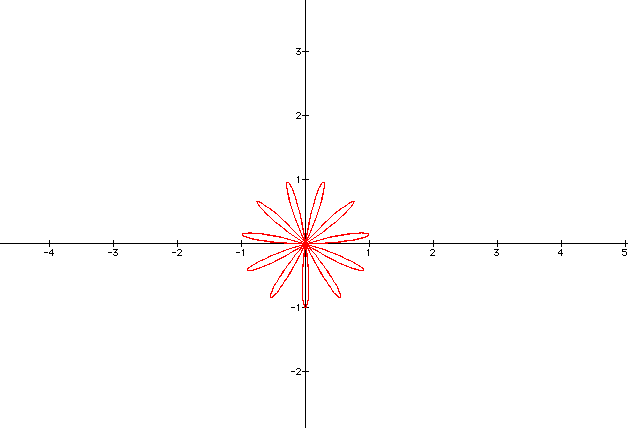
Here k=10 and is even, so there 20 leaves.

Now, we have k=11 and since k is odd, there should only be 11 leaves on the graph of the polar function.

Now, I decided to vary b as I did with the cosine function earlier and see what effect it has on the graph. With k=4 and b=2, the effect that the b has it to stretch the graph out by 2.

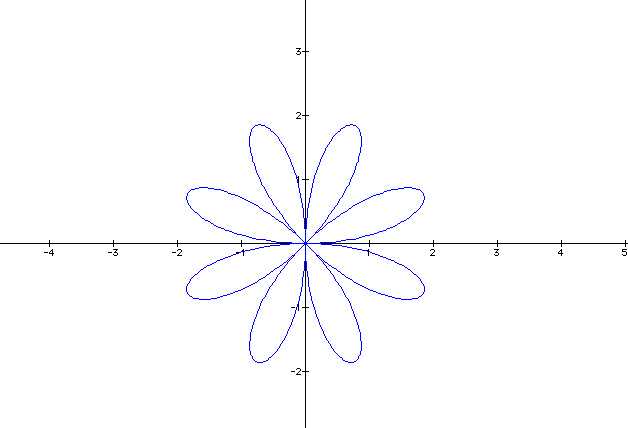
This is the case here as well. With k=4, there should be 8 leaves on the graph. With b=3, it should stretch the graph out by 3 from when b was equal to 1.

 

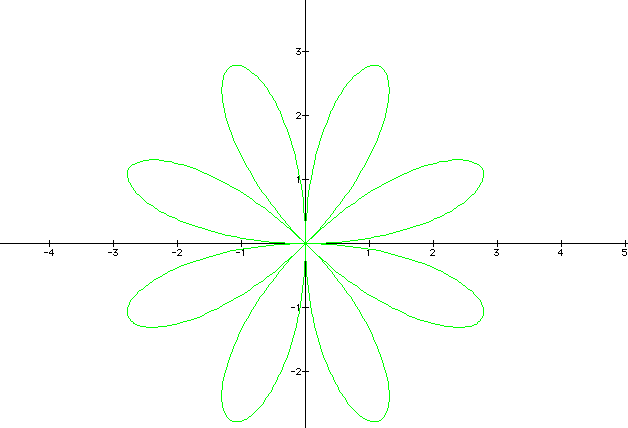
This concludes my investigation of polar equations.

 

 

 

RETURN