

Using the figure below, we will prove the Sine and Cosine Addition formulas for the two angles x and y.

Note
that ![]() are all right triangles.
are all right triangles.
The sine addition formula states that
sin(x+y) = cos(x)sin(y) + sin(x)cos(y).
Let's see if we can prove this is true using the above Figure 1.
sin(x+y)
= 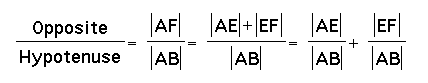 .
.
Since EDCF is a square, then segment EF is congruent to segment DC so...
sin
(x+y) =  .
.
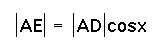 and
and
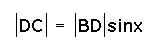 , then
, then
sin(x+y)
= 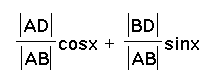 .
.
Finally,
since  , we are left with sin(x+y) = siny cosx + cosy sinx.
, we are left with sin(x+y) = siny cosx + cosy sinx.
Therefore, we have proved the sine addition formula does work!

Note
again that ![]() are all right triangles.
are all right triangles.
The cosine addition formula states that
cos(x+y) = cos(x)cos(y) - sin(x)sin(y).
cos(x+y)
=  .
.
Again, since EDCF is a square, then segment FC is congruent to segment ED, so substiting in for this, we get
cos(x+y)
=  .
.
Since
 , we get
, we get
cos(x+y)
=  .
.
And
since 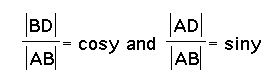 , we are left with
, we are left with
cos(x+y) = cosycosx - sinysinx.
We have proved how the sine and cosine addition formulas work!