Assignment 6: Problem Solving Using GSP

by

Melissa Silverman
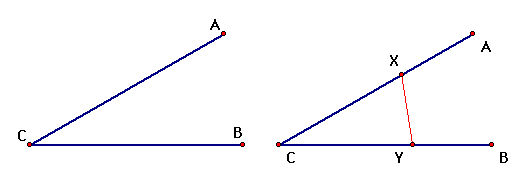
Given any angle ACB, construct a line XY such that AX=XY=YB.

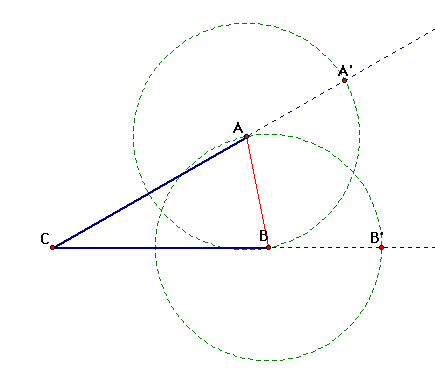
The first strategy to solve the problem that I tried was circles, because all radii of a circle are equal. I have to find equal lengths, so I thought I could use circle radii to solve it. In the picture below, given angle ACB, I can use circles to solve for A' and B' so that A'A=AB=BB'. But that is not what the problem is asking. So strategy #1 failed.

I then decided to modify the circle technique to stay more in line with the problem. I revised the positioning of my circles to try and create a winning construction. To my dismay, this failed as well.

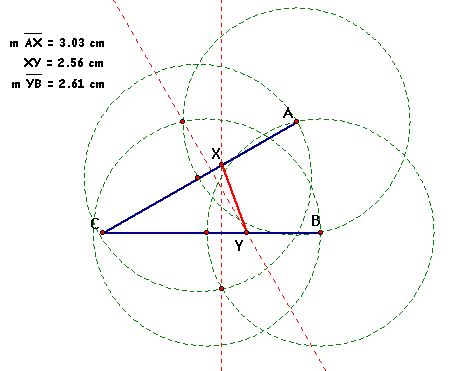I began by making a circle with center A and AB as its radius. Then I made a circle with center B and BA as its radius. I then marked where those two circles intersect the original angle. I made additional circles from there and found myself in a big mess of circles. I made perpendicular lines from a point where circles intersect to the original angle, which looked like they would be close to points X and Y. So I constructed X and Y. When I measured the segments, they were close in length but not equal. So strategy #2 failed.

Circles, I decided, was not going to be the way to an answer. I started thinking about other shapes that might work. What about triangles? So I began tinkering with triangles. If angle ACB is 60 degrees, then, using equilateral triangles, you can find XY (indicated by the red line) so that the three segments are equal.

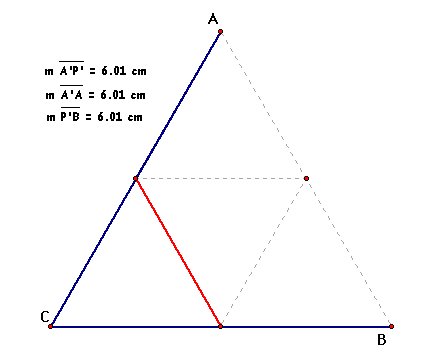But again, this only works for one angle, and the problem implies that it needs to work for any angle. So strategy #3 has failed.

So I am afraid that my many attempts to this problem failed. But that is a learning process in itself. GSP is difficult in that something that seems very easy to solve becomes more complex than you realize. As a student that has worked a lot with GSP, I often find that I can't construct the answers to what seem like simple problems because the way to solve it seems too off the beaten path. I know the solution to this problem has something to do with similar figures, for that was the clue given in class. On my own brain power, the strategy of using similar figures would never have occured to me. And even after trying to use the clue, I could not create a winning construction.

Thinking about future middle school students, I think this process might be really frustrating for them (as it is frustrating for me too!). A problem that appears easy seems to always have a really complicated construction to go with it. It seems as though problems always have their own "tricks" which are too hard to figure out on your own. If I use GSP problem solving in the classroom, I need to make sure that I choose problems that I know the students will be able to answer without excessive stress and frustration, so that the experience remains positive.

I could have thrown all of these ideas out and tried to solve another problem, but I thought that maybe showing everything that I thought of on my own to complete this problem would make for an interesting write-up. On GSP I often find myself going through this process, the process of trial and error. To get the desired product, it sometimes takes a string of ideas to get there. And sometimes even with multiple attempts, the solution is something that I just would never have seen. I think it is ok for students to know this, that sometimes problems take a few tries. But the thoughts along the way are just as valuable as the answer, because you never know what you might learn from taking a detour.
Assignment 5 | Home Page | Assignment 7