

CONSTRUCTING A NINE POINT CIRCLE.
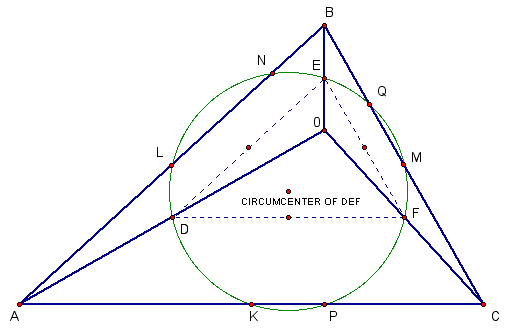
METHOD 1, using the ORTHOCENTER of the given triangle ABC. I first detrmined the midpoints of AB, AC and BC. These were named K,L and M in the above diagram. I next determined O, the orthocenter of he given triangle ABC. I then joined OA, OC and OB. Next, I determined the midpoints of OA, OC and OB. These were then named E, D and F. The Triangle EDF was next constructed. This is the ORTHIC triangle. I next dropped perpendiculars from the vertices of the ORTHIC triangle to the opposite sides. The points where these orthogonal lines intersected triangle ABC were named N,Q and P. I now had nine points K L M D E F P Q N which seem to lie on a circle. Where would the center of such a circle lie?
Well, I tried to see what the circumcircle of the Orthic circle would look like. Lo and behold! The circumcircle of the ORTHIC circle is the NINE POINT circle.
METHOD 2, using the CIRCUMCIRCLE of triangle ABC. I first determined the orthocenter of triangle ABC and constructed the circumcircle. Please see diagram 2, below. Next, I dilated the circumcircle by a ratio of 1:2 about O, the Orthocenter of triangle ABC. And guess what? It turned out that this circle passed through the same NINE points that I had used in the previous venture. This is the NINE point circle!
