

In this section, we construct a triangle whose vertices are on the sides of of another triangle. We then observe the ratio of the area of the inner triangle to that of the parent triangle. Below are four such triangles. Notice that the area ratio varies from as low as four to well over twentyfive. It never seems to go below 4.





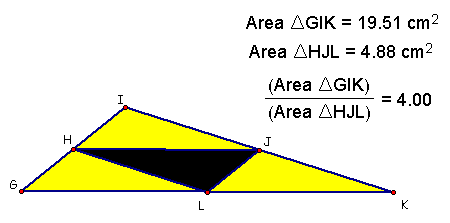
We notice that the ratio remains constant at four! This holds good if any of the two vertices of the inner triangle are located at the midpoints of the sides of the parent triangle. Thus, when the point "P" is the CENTROID of the parent triangle, the three vertices of the inner triangle would be the midpoints of the sides of the parent triangle and result in the ratio of the area of the parent triangle to the inner triangle to be equal to FOUR.
