Exploring Quadratic Equations

By

Janet M. Shiver

EMAT 6680

Investigation: Construct graphs for the parabola for different values of a, b and c. Determine the affect each of the coefficients has on the shape and

position of the graphs.

We will begin our investigation by looking at what effect the coefficient “a” has on the graph. To do this we will hold the other coefficients constant while we vary a. For the following graphs we will assign b=1 and c=1, giving us the equation .

First let’s examine what happens when a is a rational number greater than zero.

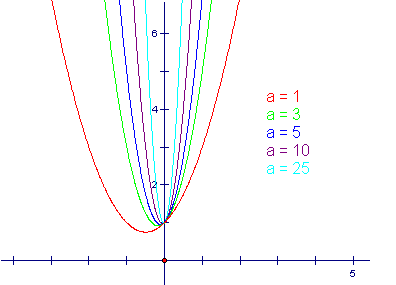
Looking at the parabolas above we notice that each parabola passes through the point (0,1). Since each parabola passes through the same point, it does not appear that any vertical or horizontal translation has effected its location. However, we will notice that the vertex of each parabola is in a different location for each of the parabolas. The location of the x coordinate of the vertex of the parabolas can be found using the formula , where a and b are the coefficients of and x respectively. The most interesting result from the change in a, however, seems to be its effect on the width of the parabola. It appears that as the coefficient, a, grows larger the breadth of the parabola seems to narrow.

Now let’s examine what happens when a is a rational number greater that zero but less than one.

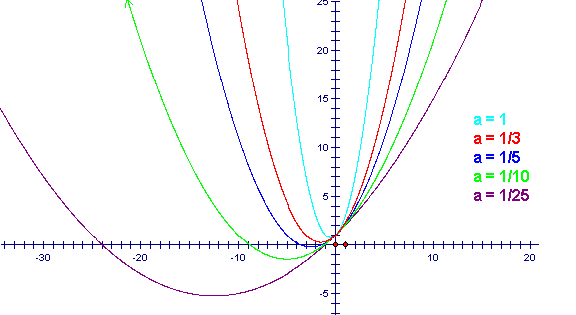
Looking at the parabolas above we notice that each parabola passes through the point (0,1). Since each parabola passes through the same point, it does not appear that any vertical or horizontal translation has affected its location. However, we will notice that the vertex of each parabola is in a different location. The location of the x coordinate of the vertex can be found using the formula , where a and b are the coefficients of and x respectively. Again the most interesting result from the change in a seems to be its effect on the width of the parabola. It appears that as the coefficient, a, grows smaller the breadth of the parabola seems to become wider.

Next, lets see what happens when a is a number less than zero. (Please note that the red parabola is determined when a =1 and is there as a reference.)

Looking at the parabolas above we notice that each parabola once again passes through the point (0,1). Since each parabola passes through the same point, it does not appear that any vertical or horizontal translation has affected its location. However, we will notice that the vertex of each parabola is in a different location. The location of the x coordinate of the vertex can be found using the formula , where a and b are the coefficients of and x respectively.

The greatest effect on the parabola when making “a” negative, seems to be its concavity. The negative coefficient appears to make the parabola concave down while the positive coefficient make it concave up. Another interesting observation is the variation in the widths of the parabolas. It appears that the width of the parabola is affected by the absolute value of “a” and not the negative. It seems that the negative only affects the direction not the width.

Finally, we need to see what happens when a is zero.

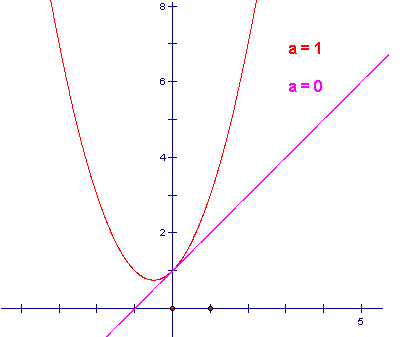
When “a” is assigned a value of zero, the graph of the equation is linear not parabolic. We can see this in the equation or when simplified y = x + 1.

One similarity to the other cases is that the graph once again passes through the point (0,1). Since the graph of the line, as well as all of the parabolas previously discussed, passes through the same point, it does not appear that any vertical or horizontal translation has affected its location.

After examining several graphs, we see an interesting trend.

As the value of a approaches 0 from both the positive and negative directions, we can see the graphs of the parabolas approaching the graph of the linear equation y = x + 1, where a =0. It appears that as the a coefficient gets closer to 0, the parabolas become more and more linear.