Assignment 10

Parametric Curves

By

Mandy Stein

A parametric curve in the plane is a pair of functions, called the parametric equations of a curve,

x = f(t)

y = g(t)

where the two continuous functions define ordered pairs (x, y). The extent of the curve will depend on the range of t.

Here is an example of the parametric curve defined by

x = cos(t)

y = sin(t)

for 0 < t < 2p

Problem 3.

For various a and b, investigate

x = a cos(t)

y = b sin (t)

0 < t < 2p

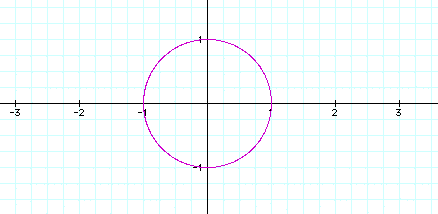
First, let's look at the graph when a =1 and b = 1.


x = cos (t)
y = sin (t)

The graph is a circle with a radius of 1.

Next, let's examine what happens when we set a =1 and b = -5.


x = cos (t)
y = -5 sin (t)

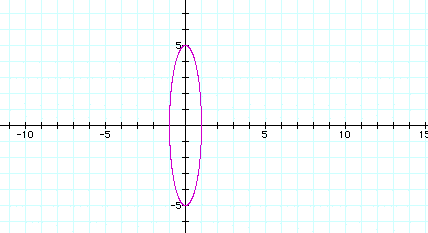
Now we have an ellipse instead of a circle. Looking at this graph, it appears that the b value alters the vertical component of the graph and that the ellipse crosses the y-axis at the positive and negative b values.

Let's look at a few more values of b to confirm this speculation.


x = cos (t)
y = -3 sin (t)

 


x = cos (t)
y = -sin (t)

 


x = cos (t)
y = 3 sin(t)

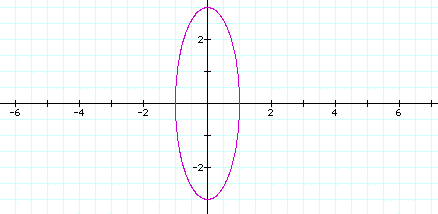
Looking at those graphs confirms that the b value alters the vertical component of the graph and that the graph crosses the y-axis at the positive and negative b values. Another interesting thing noticed is that the graph is the same for a positive and negative values of b.

Next, let's set b =1 and observe what happens when we change the value of a. First, let's set a = -5.


x = -5 cos (t)
y = sin (t)

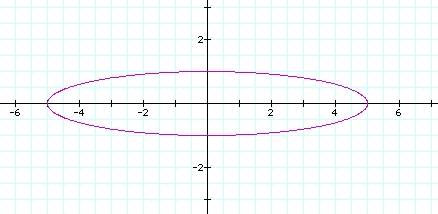
Once again we have an ellipse. Looking at this graph, it appears that the a value alters the horizontal component of the graph and that the ellipse crosses the x-axis at the positive and negative a values.

Let's look at a few more values of a to confirm this speculation.


x = -3 cos (t)
y = sin (t)

 


x = - cos (t)
y = sin (t)

 


x = 3 cos (t)
y = sin (t)

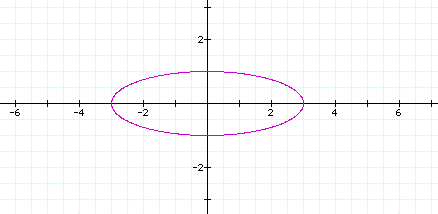
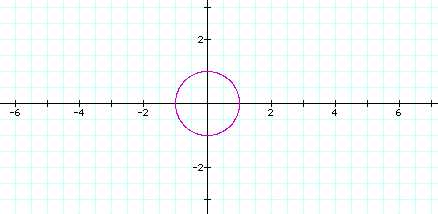
Looking at those graphs confirms that the a value alters the horizontal component of the graph and that the graph crosses the x-axis at the positive and negative a values. We also notice that the graph is the same for a positive and negative values of a.

Now that we know the a value alters the horizontal component of the graph and the b value alters the vertical component of the graph, let's change both values at the same time and see what happens.

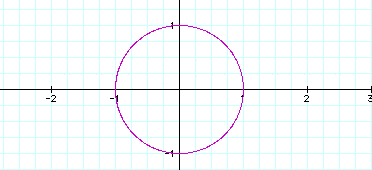
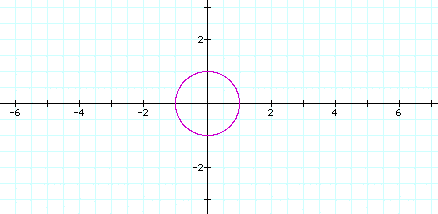
First, let's set a and b to the same value.


x = 3 cos (t)
y = 3 sin (t)

 


x = 5 cos (t)
y = 5 sin (t)

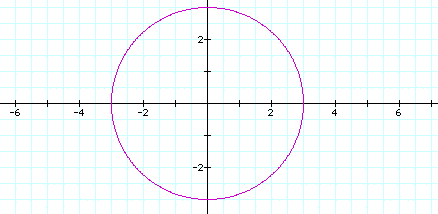
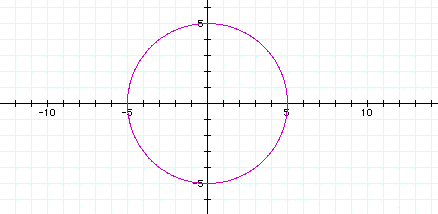
We notice from these graphs that if a and b are the same value then the graph is a circle with a radius equal to the value of a and b.

Next, let's examine what happens when we set a = 0 and change the value of b.


x = 0 cos (t) = 0
y = 5 sin (t)

 


x = 0 cos 9t) = 0
y = -2 sin (t)

Looking at these graphs we see that when a = 0 the graph becomes a vertical line with length 2b.

Next, let's examine what happens when we set b = 0 and change the value of a.


x = 5 cos (t)
y = 0 sin (t) = 0

 


x = -3 cos (t)
y = 0 sin (t) = 0

Looking at these graphs we notice that when b = 0 the graph collapses to a horizontal line with length 2a.

Return