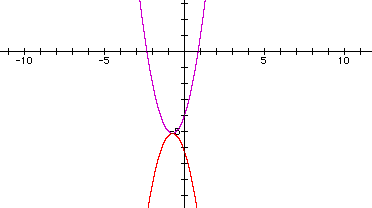
Consider the graph of y = 2x^2 + 3x - 4 (shown below in purple) with vertex (-3/4, -41/8).
Substituting (x + k) for x in the equation moves the graph horizontally along the line y = -41/8. For positive k, the graph is moved |k| units to the left. For negative k, the graph is moved |k| units to the right.
For example, the graph of y = 2(x - 4)^2 + 3(x - 4) - 4 = 2x^2 - 13x + 16 is shown below in red. Replacing each x in the original equation by (x + (-4)) moves the graph four units to the right.
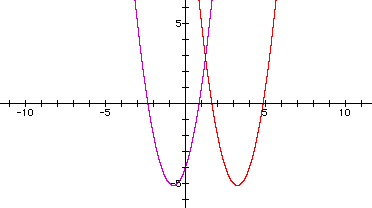
To move the vertex of the graph of y = 2x^2 + 3x - 4 into the second quadrant, we must replace the c-value (i.e., -4) with a number which will alter the equation in such a way that the vertex of its graph has a positive y-coordinate.
We know that the vertex of any graph of ax^2 + bx + c is
Replacing a by 2 and b by 3, we see that the vertex is
So, the vertex of the graph of y + 2x^2 + 3x + c is in the second quadrant when c is greater than 9/8. Below, in red, is the graph of the equation when c = 2.

Next, we consider how to produce a graph concave down that shares the same vertex with y = 2x^2 + 3x - 4. If we consider y = -(2x^2 + 3x - 4), then we observe that the graph is concave down and on the same axis; but, the vertices are not the same.
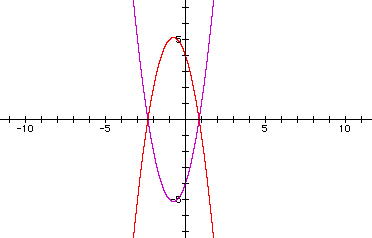
Thus, we seek a c-value for the equation y = -2x^2 - 3x + c, such that its vertex, (-3/4, (9 + 8c)/8), is one-and-the-same as (-3/4, -41/8). Solving the equation, (9 + 8c)/8 = -41/8, we determine that c must equal -25/4. Therefore, the graph of y = -2x^2 - 3x - 25/4 is concave down and shares the same vertex with the given equation.