Let triangle ABC be any triangle. Then if P is any point in the plane, then the triangle formed by constructing perpendiculars to the sides of ABC (extended if necessary) locate three points R, S, and T that are the intersections. Triangle RST is the Pedal Triangle for Pedal Point P.

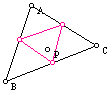
Click here for a GSP script for the general construction of a pedal triangle of triangle ABC where P is any point in the plane ABC.

Some special properties occur when pedal point, P, is in a certain location. We will discover some of these special properties and take a look at why some of them occur.

Let's suppose that the pedal point, P, is the incenter of triangle ABC. We can observe the following:

The pedal triangle is always inside triangle ABC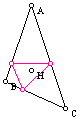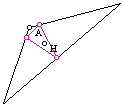

When triangle ABC is isosceles, the pedal triangle is also isosceles.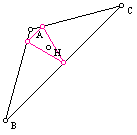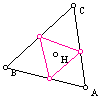

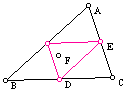
Let's suppose that the pedal point is the centroid. We can observe a few things by looking at a GSP sketch.

When triangle ABC is an equilateral triangle, so is the pedal triangle. When triangle ABC is an isosceles triangle, so is the pedal triangle.

Click here to try out this idea on GSP.

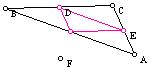
The last case we will consider is when the pedal point is the circumcenter. One of the first things I noticed by exploring on Geometer's Sketchpad was that the pedal triangle seemed to create four congruent triangles inside the larger triangle. Perhaps the pedal triangle is also the medial triangle. When I began to measure, I realized that in fact the vertices of the pedal triangle were the midpoints of the original triangle ABC. The reason for this is that the circumcenter is the center of the circumcircle. Thus, the circumcenter is equidistant from each of the vertices of triangle ABC. Thus, the circumcenter lies on the perpendicular bisector of each side. Since the perpendicular bisector of each side intersects each side at the midpoint of each side, then the pedal triangle formed is also the medial triangle. The four smaller triangles are also similar to the larger triangle, ABC by Side-Angle-Side similarity.

Extensions:

1. Look at the case where the pedal point is the orthocenter.

2. Look at the case where the pedal point is on one of the sides of the triangle ABC.

3. Look at the case where the pedal point is one of the vertices of the triangle ABC.

Return to April's home page