,there are some instances which produce some very interesting graphs. Some of my explorations and findings are included below.
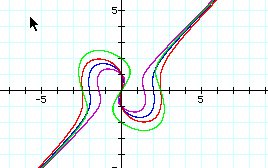
Let's look at the first case where a is not equal to b. This graph is a function as below. As a and b become smaller, the graph moves closer to the origin. As a and b become larger, the graph moves away from the origin. The graph below shows some examples.
The blue graph is of the equation
.
The purple graph is of the equation 
.
The red graph is of the equation 
.
The green graph is of the equation 
.
In the graph of , the square root of a is where the graph crosses
the x-axis. The square root of b is where the graph crosses the
y-axis.
The next case of interest is when a = b. This equation creates a graph with an oval and a line going through the oval as below.
The blue graph is of the equation 
.
The purple graph is of the equation 
.
The red
graph is of the equation 
.
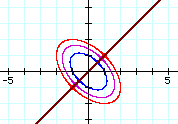
As one can see, as a and b increase, the graph moves away from the origin. The line is always the same line x=y. The graph crosses the x-axis at the square root of a and the graph crosses the y-axis at the square root of b.
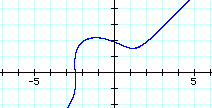
What happens if we add a constant to the right side of the
equation? The top dip no longer exists. For example, look at the
graph 
.
Let's also look at what happens if we add a constant to the left side of the equation. The lower dip no longer exists.
Looking at the graph of
we can see there is only an upper dip.
