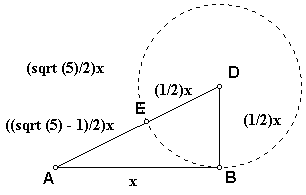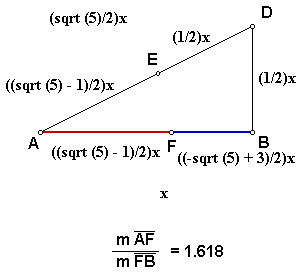Department of Mathematics Education
Dr. J. Wilson, EMAT 6690

Problem and Solution

1. Given any line segment AB.

• Construct segment AB and label it x.

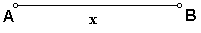
2. Construct a perpendicular segment BD usch that 2BD = AB.

• Construct a perpendicular line to segment AB through point B.
• Construct the midpoint of segment AB.
• Construct a circle by center and point with point B as the center and the midpoint of segment AB the point.
• Construct the intersection point of the circle and the perpendicular line to segment AB. Label one of the constructed intersection points D and construct the segment BD.
• Hide the midpoint of segment AB, the circle, the perpendicular line, and the other intersection point (not labelled D) of the circle and the perpendicular line to segment AB.
• Note: The described construction procedure is based upon Greek constructions. This construction could also be done using a rotation.
• Since AB is x, BD must equal (1/2)x.

3. Draw AD.

• Since segment BD is perpendicular to segment AB, triangle ABD is a right triangle.
• Using the Pythagorean theorem, the length of AD is calculated to be (sqrt (5)/2)x.

4. Mark point E on AD such that DE = BD.

• Construct a circle by center and point with point D as the center and point B as the point.
• Construct the point on intersection of the circle and segment AD and label point E.
• Since DE = BD, DE must equal (1/2)x. Since AE + ED = AD, AE + (1/2)x = (sqrt (5)/2)x.
  Therefore, AE = ((sqrt (5) - 1)/2)x.

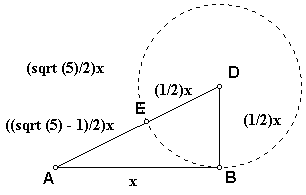
5. Mark point F on segment AB such that AE = AF.

• Construct a circle by center and point with point A as the center and point E as the point.
• Construct the point on intersection of the circle and segment AB and label point F.
• Since AE = AF, AF must equal ((sqrt (5) - 1)/2)x. Since AF + FB = AB, ((sqrt (5) - 1)/2)x + FB = x.
  Therefore, FB = x - ((sqrt (5) - 1)/2)x, or ((-sqrt (5) + 3)/2)x.

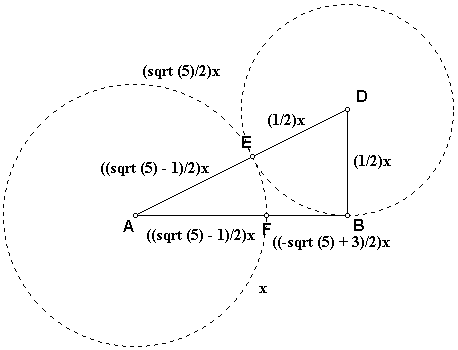
6. Find AF/BF.

• AF/BF = ((sqrt (5) - 1)/2)x / ((-sqrt (5) + 3)/2)x = (sqrt (5) - 1) / (-sqrt (5) + 3).
• Through rationalizing the denominator, AF/BF = (1 + sqrt (5))/2, which is approximately equal to 1.618.
• Therefore, point F divides segment AB into the golden ratio.

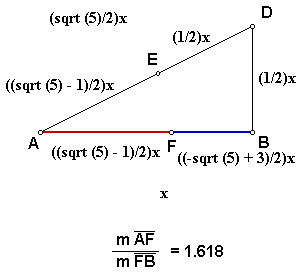
Click here to investigate a GSP sketch for yourself.

If you have any suggestions that would be useful, especially for use at the high school level, please send e-mail to esiwdivad@yahoo.com.

Return to my homepage.