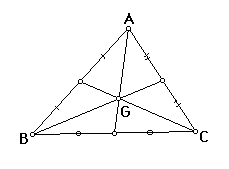
1. The CENTROID(G) of a triangle is the common intersection of the 3 medians.

A median of a triangle is the segment from a vertex to the midpoint of the opposite side.
2. The ORTHOCENTER(H) of a triangle is the common intersection of the 3 lines containing the altitudes.
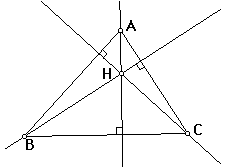
An altitude is a perpendicular segment from a vertex to the line of the opposite side.
Note: the foot of the perpendicular may be on the extension of the side of the triangle. It should be clear that H does not have to be on the segments that are the altitudes. Rather, H lies on the lines extended along the altitudes.
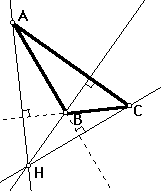
3. The CIRCUMCENTER(C) of a triangle is the point in
the plane equidistant from the 3 vertices of the triangle. Since
a point equidistant from two points lies on the perpendicular
bisector of the segment determined by the two points, C is on
the perpendicular bisector of each side of the triangle. Note:
C may be outside of the triangle. Here is a constructed circumcenter
C. C is the center of the CIRCUMCIRCLE (the circumscribed circle)
of the triangle. (Explanetion of
the circumcenter)
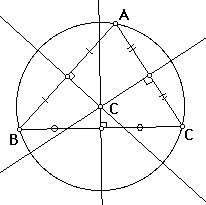
4. The INCENTER(I) of a triangle is the point on the interior of the triangle that is equidistant from the3 sides. Since a point interior to an angle that is equidistant from the two sides of the angle lies on the angle bisector, then I must be on the angle bisector of each angle of the triangle.Here is a constructed incenter I. I is the center of the INCIRCLE (the inscribed circle) of the triangle. (Explanetion of the incenter)
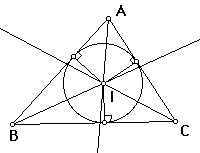
5. Using GSP to construct G, H, C, and I for the same triangle. What relationships can you find among G, H, C, and I or subsets of them?
Explore the relationships of G, H, C, and I with some sketch.
( Sketch 1 : move A along the line, Sketch 2 : move A along the circle, Sketch 3 : G, H, C on the line.)
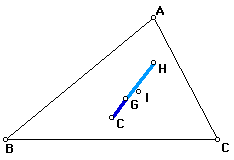
As we move the three vertices and the three side of the triangle,
the positions of G,H,C and I change.
But we find that there is an interesting relationship among G,H
and C, the vertices A,B and C are moved anywhere.
Look the movements of the figures and the values of length and
ratio at the same time!
G,H,C are on the same line, and the ratio of the length CG:GH=1:2,
CG:CH=1:3 does not change.
The colored line is called the Euler Line.
6. The Nine-Point circle for any triangle passes through the three mid-points of the sides, the three feet of the altitudes, and the three mid-points of the segments from the respective vertices to orthocenter. Here is the Nine-Point circle, the constructed center (N) and the nine point circle.
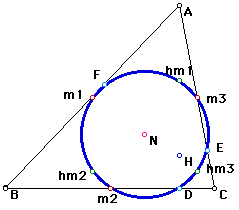
There are Nine-Points, three mid-points of the sides(m1, m2, m3), the three feet of the altitudes(D, E, F), and the three mid-points(hm1, hm2, hm3) of the segments from the respective vertices to orthocenter. Here is the constructed cicle with animation by GSP, and the script.