

Problem: Rational or Irrational
To see a detail statement of the problem, click here.
Let ![]() and assume that x is rational,
however it is not an integer. There should exist a minimal positive
integer n such that xn is an integer. Consider
and assume that x is rational,
however it is not an integer. There should exist a minimal positive
integer n such that xn is an integer. Consider ![]() . Since the fractional part of x,
. Since the fractional part of x, ![]()
Note that mis an integer for m=nx-n[x] which is an integer.
Also, 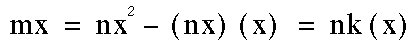 which is also an integer. Due to
the minimality of n, m=0. In other words, x =[x] and is an integer
in contradiction to our assumption.
which is also an integer. Due to
the minimality of n, m=0. In other words, x =[x] and is an integer
in contradiction to our assumption.
Reference:
http://cut-the-knot.com/content.html