

Part 1:
A. Consider any triangle ABC. Select a point P inside the triangle and draw lines AP, BP, and CP extended to their intersections with the opposite sides in points D, E, and F respectively.
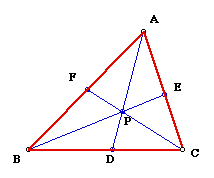
Explore (AF)(BD)(EC) and (FB)(DC)(EA) for various triangles and various locations of P.
Exploration:
Click here to explore triangle ABC.
(AF)(BD)(EC) was equal to (FB)(DC)(EA) for any point P inside or outside the triangle for any type of triangle.
B. Conjecture? Prove it!
Conjecture: (AF)(BD)(EC) = (FB)(DC)(EA)
Proof:

First, draw two lines parallel to segment AD through points B and C. Then extend segments BE and CF until they intersect the parallel lines at points H and I respectively.
This constructs 4 sets of similar triangles:
Triangles CBI & CDP; BCH & BDP; CHE & APE; and
BIF & APF are sets of similar triangles.
So,
CB/CD = BI/DP = CI/CP and
BC/BD = CH/DP = BH/BP and
CH/AP = CE/AE = HE/PE and
BI/AP = IF/PF = BF/AF.
Then,
(CB/CD)/(BC/BD) = (BI/DP)/(CH/DP).
So, (BD/CD) = (BI/CH).
But,
(BF/AF) = (BI/AP) and (CE/AE) = (CH/AP).
Therefore,
(BF/AF)/(CE/AE) = (BI/AP)/(CH/AP).
So, (BF)(AE)/(AF)(CE) = (BI/CH).
But,
(BD/CD) = (BI/CH).
So,
(BF)(AE)/(AF)(CE) = (BD/CD).
Therefore,
(BF)(AE)(CD) = (BD)(AF)(CE).
Thus,
(AF)(BD)(EC) = (FB)(DC)(EA).
Yes. This relationship can be generalized using lines instead of segments to construct the triangle ABC. The point P can be inside or outside of the triangle ABC and the relationship (AF)(BD)(EC) = (FB)(DC)(EA) is still valid.
Click here to see extended exploration of triangle ABC, point P, and the relationship between (AF)(BD)(EC) = (FB)(DC)(EA).
C. Show that when point P is inside triangle ABC, the ratio of areas of triangles ABC and DEF is always greater than or equal to 4. When is it equal to 4?
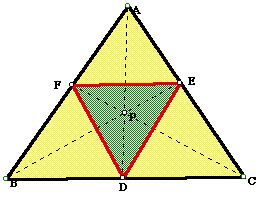
CLICK HERE to observe that the ratio of the areas of triangles ABC and DEF is always equal to or greater than 4 when point P is inside triangle ABC.
The ratio of the areas of triangles ABC and DEF = 4 when points D, E, and F are the midpoints of the sides of triangle ABC. Thus when triangle DEF is the medial triangle for triangle ABC the ratio of the areas of triangles ABC and DEF is equal to 4.
Part II:
The course evaluation was completed and sent as an email message to Dr. Hatfield.