


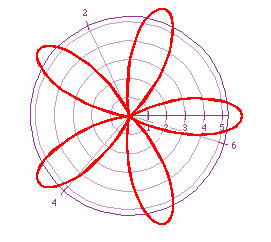
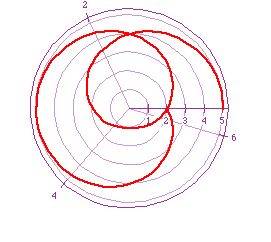
The next group of graphs show a = 2, b = 3 ( a < b) while k = 3
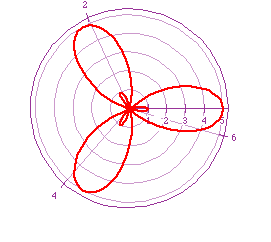

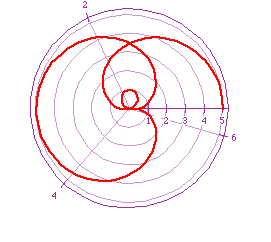
The next group show a = 3, and b = 2 ( a > b ) while k = 3,
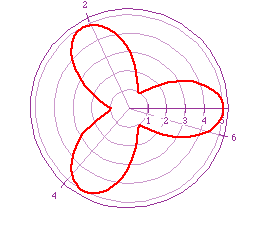
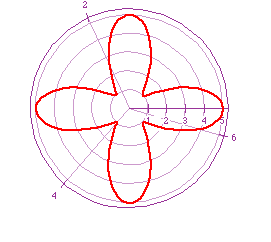
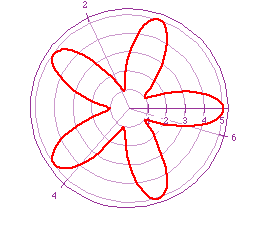
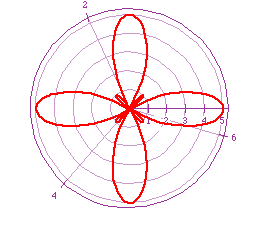
Wh en a = b, the k leaf rose emerges. With a < b, a k leaf rose and
a smaller k leaf rose appear. With a > b, a k 'finger' curve with a hole
in the middle appears.
k = 1/2, 3/2, 5/2,....... for values from 0 to 720 degrees inclusive
The first group show the variance when a = b = 3, and k = 1/2,
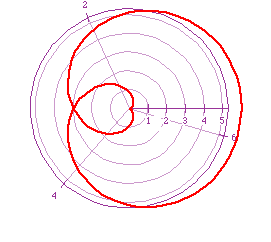
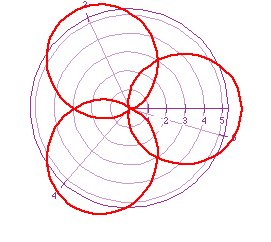

The next group shows a = 2, b = 3 ( a < b ) and k = 1/2,
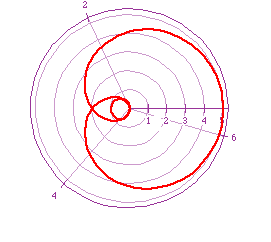
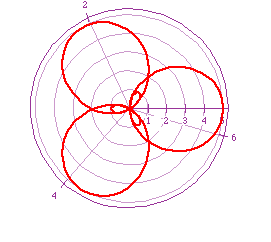
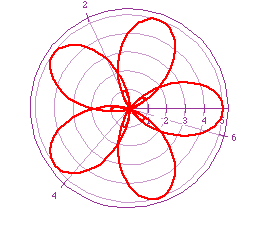
The next group show a = 3,b = 2, ( a > b ) and k = 1/2,
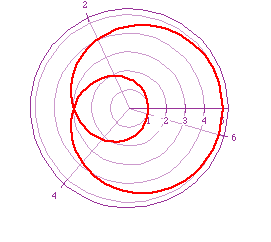
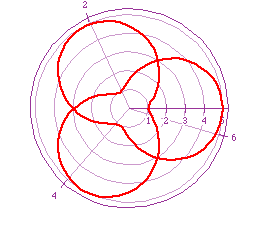

When a = b, the figure resembles 2k arcs with 2k petals in the arcs.
When a < b, the figure is similar to when a = b with a smaller petal
inside each of the first group of petals. When a > b, the arcs are enlarged
so that the petals do not join as 2k > 1. As 2k gets very large, the
arcs should form a nearly circular pattern near the outside of the grid.
k = 1/3,2/3,4/3,......for values from 0 to 1080 degrees inclusive.
The first group show a = b = 3, and k = 1/3,

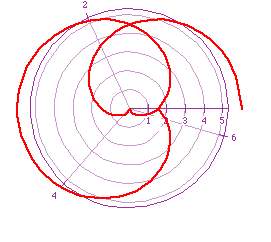
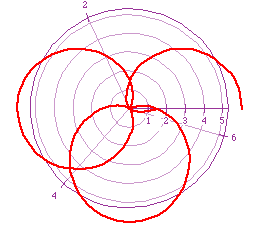
The next group show a = 2, b = 3 ( a < b ) and k = 1/3
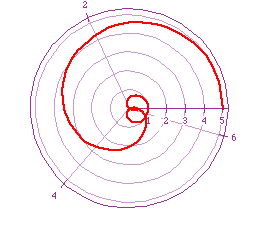
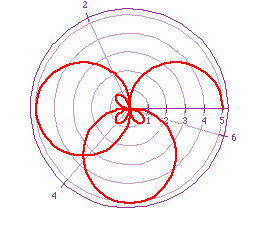
The next group show a = 3, b = 2 ( a > b ) and k = 1/3
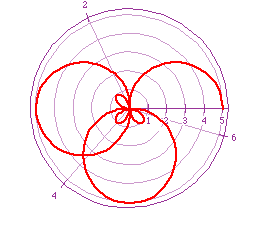
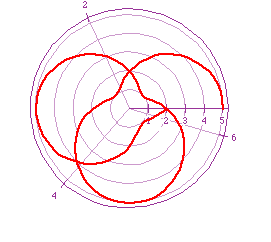
When a = b, the graph shows intersecting spirals, with a < b, a second
group of small spirals emerge outside the original set of arcs, and when
a > b, the second set of small spirals appear inside the larger set of
arcs.
The pattern shows a start of k leafs to variations where the leafs become
intersecting arcs and many more points must be plotted to show a smooth
curve.