and to overlay several graphs of
for different values of a, b, or c as the other two are held constant.
These graphs enable students to better understand a discussion of the patterns
for the roots of
.For example, if we set
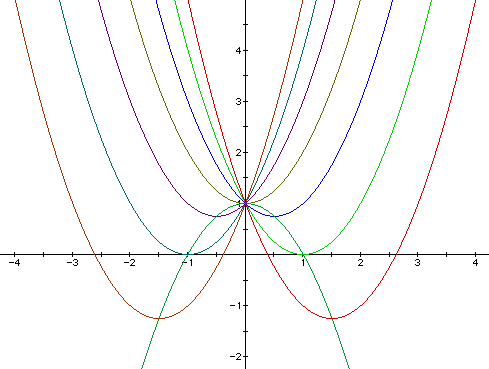
for b = -3, -2, -1, 0, 1, 2, 3, and overlay the graphs, the following
picture is obtained:
From the graph, we can have a very meaningful observation and discussion
of the movement of the parabola as b is changed. The parabola always passes
through the same point on the y-axis ( the point (0,1) with this equation).
In addition, students should be able to make the very important connection
between the solutions of this family of equations and the intersections
of their graphs with the x-axis. In particular, it is very important for
students to make the connection that a real root will always lie on the
x-axis, and imaginary roots will not. It would be a good idea here to solve
a few of the equations for the different values of b. This would require
factoring, completing the square, or the quadratic formula, and analyzing
the types of roots.
For b < -2 , we see from the graph that the parabola will intersect the
x-axis in two points with positive x values (i.e. the original equation
will have two real roots, both positive). For b = -2, the parabola is tangent
to the x-axis and so the original equation has one real and positive root
at the point of tangency. For -2 < b < 2, the parabola does not intersect
the x-axis -- the original equation has no real roots. Similarly for b =
2 the parabola is tangent to the x-axis (one real negative root) and for
b > 2, the parabola intersects the x-axis twice to show two negative
real roots for each b.
Now consider the locus of the vertices of the set of parabolas graphed from
.After carefully studying the locus of vertices for this set of parabolas,
it becomes apparent that the locus is another parabola. To find the equation
of this parabola, all we need is the vertex and another point that lies
on the parabola (a vertex of one of the parabolas above). We see from the
graph above that the vertex of the new parabola would have to be (0,1).
Next, we need another point, say (1,0). Since the general form of the equation
for a parabola is 
, where (h,k) is the vertex,
we can substitute (1,0) for (h,k), and (0,1) for (x,y). This gives us the
following equation:
.
Sure enough, our locus of vertices is the equation . The general
form of this locus is 
for any family of curves.
Consider again the equation
Now graph this relation in the xb plane. We get the following graph:
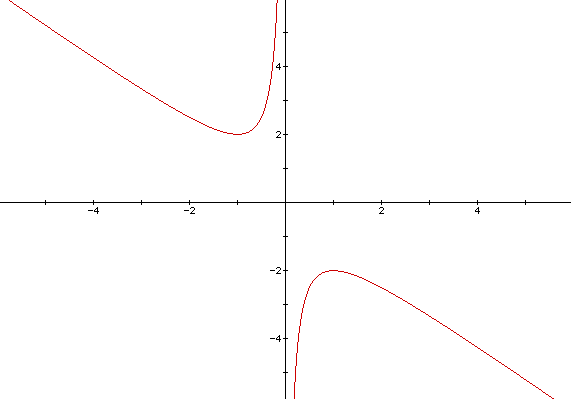
What we get here is a hyperbola, with the two variables being x and b,
instead of x and y. If we take any particular value of b, say b = 3, and
overlay this equation on the graph, we add a line parallel to the x-axis.
We can see from the graph below that the intersections of the curve and
any horizontal line, say b=3 , give us the roots of the equation in
the xb plane for that particular value of b. We have the following graph.
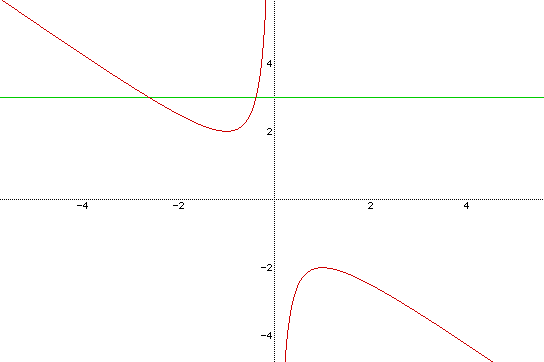
For each value of b we select, we get a horizontal line. It is clear
on a single graph that we get two negative real roots of the original equation
when b > 2, one negative real root when b = 2, no real roots for -2 <
b < 2, One positive real root when b = -2, and two positive real roots
when b < -2. Again, it is important here for students to make the connection
between real and imaginary roots, and the graphs of these equations.
Consider the case when c = - 1 rather than + 1.
In the following example the equation
is considered. If the equation is graphed in the xc plane, it is easy
to see that the curve will be a parabola. For each value of c considered,
its graph will be a line crossing the parabola in 0, 1, or 2 points -- the
intersections being at the roots of the orignal equation at that value of
c. In the graph below, the graph of c = 1 is shown. The equation
will have two negative roots -- approximately -0.2 and -4.8.
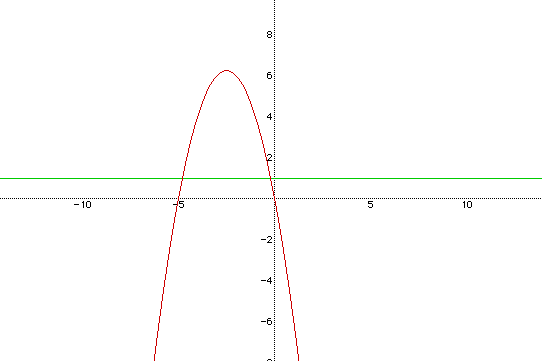
There is one value of c where the equation will have only 1 real root
-- at c = 6.25. For c > 6.25 the equation will have no real roots and
for c < 6.25 the equation will have two roots, both negative for 0 <
c < 6.25, one negative and one 0 when c = 0 and one negative and one
positive when c < 0.
Hopefully we have discussed several different and meaningful ways to examine
the equation by graphing and examining various patterns that occur
in relation to the roots of this equation. It is our main objective, with
technology, to enable students to graph many of these equations in a short
period of time. It has been my experience that activities of this nature
makes mathematics more accessible and valid to students.