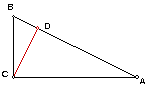
4a. Prove that the altitude on the hypotenuse of a right triangle is the mean proportional between the segments of the hypotenuse.
Proof:

Given right triangle ABC. Construct the altitude CD as shown. m(BAC) = m(DAC) by angle measure theorem. So, m(BAC) = -m(CAD) by angle measure theorem. m(ACB) = -m(ADCc) since both are right angles. So, triangle BAC is similar to triangle CAD by angle-angle theorem. Likewise, m(CBA) = m(CBD) by angle measure theorem. So, m(CBA) = -m(DBC) by angle measure theorem. m(ACB) = -m(CDB) since both are right angles. So, triangle BAC is similar to triangle BCD by angle-angle theorem. So, triangle CAD is similar [with s=1] to triangle BCD by transitivity. So, d(CD)/d(BD) = k and d(AD)/d(CD) = k by definition of similarity. So, d(CD)/d(BD) = d(AD)/d(CD) [by transitivity] which is equivalent to (d(CD))^2 = d(AD)d(BD). QED
4b. Given a triangle, construct a square with the same area.
Given any triangle ABC, construct an altitude such that every point along the altitude is inside the triangle. This is obvious, so I have included pictures to show this is true for the three types of triangles: acute, obtuse, right (l-r).

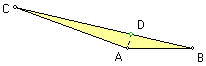
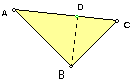
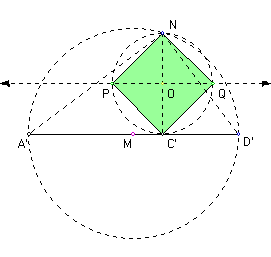
Construct a line and a point, A', on the line (recall we have shown in the beginning of the course that geometry objects are translatable). Construct a circle, c1, with center A' and radius = d(AC) [refer to acute angle drawing above for labels]. Construct one of the intersections, C', of the circle, c1, and the line. Construct the circle, c2, with center C' and radius = d(BD). Construct the intersection, D', of the circle, c2, with the line such that C' is between A' and D'. Construct the segment A'D' [by construction and by the distance theorem d(A'D') = d(A'C') + d(B'D') = d(AC) + d(BD)]. The reason that I have done this is because I will use the mean proportional; that is, d(AC)d(BD) = s^2 where s is the mean proportional between d(AC) and d(DB). To complete the problem, I still need to include a factor of 1/2 in this equation because 1/2 d(AC)d(BD) is the area of the given triangle.

Construct the midpoint, M, of A'D'. Construct a circle, c3, with center M and radius = d(MD'). Construct the line perpendicular to A'D' through point C'. Construct one of the intersections, N, of the circle, c3, and the perpendicular. So, m(A'ND') = 90 degrees since the triangle is inscribed in a semicircle (i.e., the angle A'ND' = 1/2 (180 degrees) = 90 degrees). Construct the segment C'N. We now have a right triangle A'ND' and its altitude C'N.
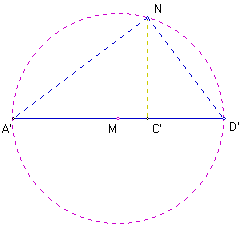
The mean proportional between A'C' and C'D' is d(C'N)^2 = d(A'C')d(C'D'). But we need s^2 = 1/2 d(A'C')d(C'D') or 2s^2 = d(A'C')d(C'D') where s is the length of the sides of the square. But if C'N is the diagonal of the square, then d(C'N)^2 = 2s^2 by Pythagorean theorem! So, to construct the square with diagonal C'N, construct the midpoint,O, of C'N. Construct the circle, c4, with center, O, and radius = d(OC'). Construct the bisector of C'N through point O. Construct both intersections, P and Q, of the circle, c4 and the perpendicular bisector. Construct the segments C'Q, QN, NP, PC'. Thus, the square C'QNP has the same area as the triangle ABC. By transitivity, we have s^2 = 1/2 d(A'C')d(C'D') which implies s^2 = 1/2 d(AC)d(CD) by substitution.
Here is the GSP file with the construction.
Here is a JSP page with an abbreviated construction (you need to have a java-enabled browser)
Return to geometry page