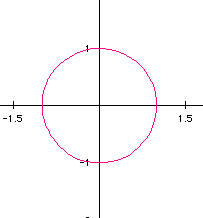
The purpose of this assignment is to investigate the parametric curve x
= a cos (t), y = b sin (t) and note the changes that occur by varying the
values of a and b. A good start would be to allow a = 1, b = 1.

It appears as though the figure is a circle with radius 1 and center
at the origin. We may also choose larger values of a and b.
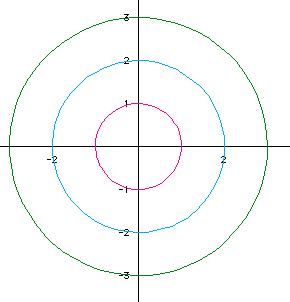
The pink circle resulted from a = 1, b = 1, the blue circle resulted
from a = 2, b = 2, and the green circle resulted from a = 3, b = 3. Hence,
as the values of a and b increase, the radius of the circle increases as
well. In fact, the radius of the circle is the same as the value of a and
b.
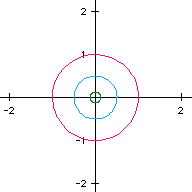
In the picture above, the blue circle is the result of allowing a = (1/2),
b = (1/2), and the green circle is the result of letting a = (1/8), b =
(1/8). It follows that if the value of a and b is a rational number between
-1 and 1, then the circle becomes smaller and the radius of the circle is
still the same as the value of a and b.
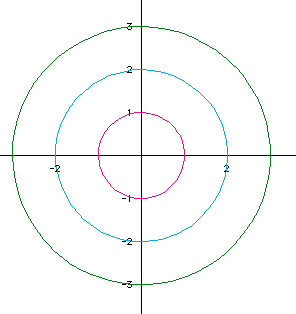
Here, the blue circle is the result of allowing a = -2, b = -2; the green
circle is the result of allowing a = -3, b = -3. Indeed, these are the same
graphs as before by letting a = b = 2 and a = b = 3, respectively. Provided
a and b are integers and a = b, the circle has its center at the origin
and the radius is given as |a|.
Given the function x = a cos (t), y = b sin (t), the graph results in
a circle centered at the origin. If a and b are integers and a = b, then
the radius of the circle is given by |a|. If a and b are rational numbers
between -1 and 1 and a = b, the result is a circle with its center at the
origin and its radius is | a |.