 Tangent Circles
By: Stephen Bismarck
Tangent Circles
By: Stephen Bismarck
Given two circles find a circle tangent
to both. This might be hard to imagine so here is a picture of
what I'm talking about.
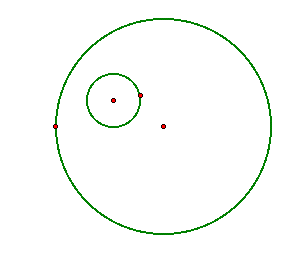 This is the case where one circle is
disjoint inside the other, you could have two circles that are
disjoint outside of each other too.
This is the case where one circle is
disjoint inside the other, you could have two circles that are
disjoint outside of each other too.
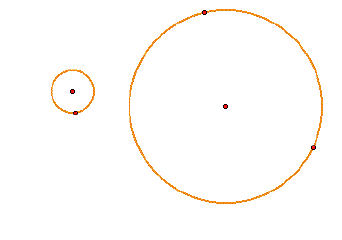 How many tangent circles are there
for these pictures?
That was sort of a trick question,
there are an infinite amount of tangent circles.
To figure out one tangent circle, there
has to be a given point on either of the circles that will be
on the tangent circle also.
Here are two GSP scripts that enable
you to get tangent circles.
Tangent
Circle
Tangent
Circle incompasing both given circles
If there is a circle inscride the other,
like in the first picture, what is the locus of the center of
the tangent circles?
How many tangent circles are there
for these pictures?
That was sort of a trick question,
there are an infinite amount of tangent circles.
To figure out one tangent circle, there
has to be a given point on either of the circles that will be
on the tangent circle also.
Here are two GSP scripts that enable
you to get tangent circles.
Tangent
Circle
Tangent
Circle incompasing both given circles
If there is a circle inscride the other,
like in the first picture, what is the locus of the center of
the tangent circles?
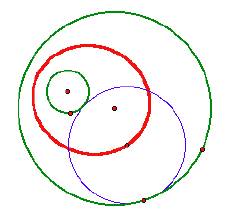 The red trace is the locus of the center
of the blue tangent circle. As you can see the locus is an ellipse
with foci at the center of the two original circles.
When the circles are disjoint the result
is much different.
The red trace is the locus of the center
of the blue tangent circle. As you can see the locus is an ellipse
with foci at the center of the two original circles.
When the circles are disjoint the result
is much different.
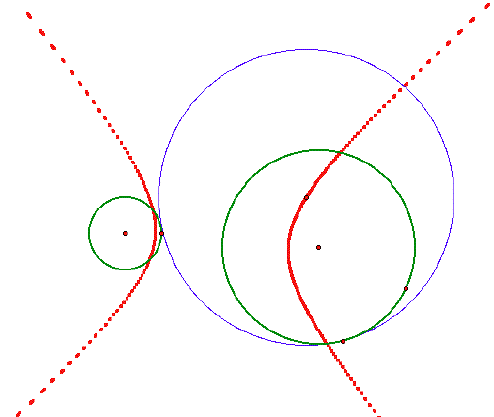 The locus in this case turns out to
be a hyperbola with the foci again the centers of the two given
circles.
I have found that it does not matter
what size the circles are, just if they are disjoint outside.
RETURN
The locus in this case turns out to
be a hyperbola with the foci again the centers of the two given
circles.
I have found that it does not matter
what size the circles are, just if they are disjoint outside.
RETURN





