EMT 469 Instructional Unit

**This unit was created using chapter seven from the text Geometry: An Integrated Approach, by Larson, Boswell, and Stiff .
In this chapter, you will be studying three basic transformations of figures in a plane:
* Reflections
* Rotations, and
* Translations.
Days One and Two:
Goal one: You should be able to identify the three basic transformations in a plane.
Goal two: You should be able to use transformations to identify patterns and their properties in real world situations.
Goal three: You should be able to properly use technology(Geometer's Sketchpad) to construct transformations.
There are three basic types of transformations:
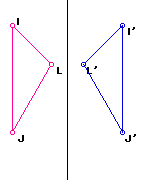
1. Reflection: Triangle ILJ is reflected about line Q. Triangle ILJ is called the preimage. It's reflection, triangle I'L'J', is called the image. Another way to think about reflections is that they are mirror images. A real life example of a reflection is a butterfly.
Q
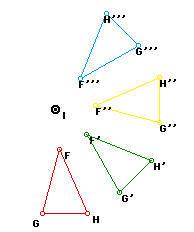
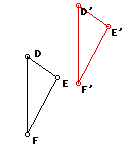
2. Rotations: Triangle FGH, the preimage, is rotated about the point I by 45 degrees. The new triangle F'G'H', the image, is the result of the rotation. Next the preimage, triangle FGH, is rotated about the point I by 90 degrees and then again by 135 degrees. As you can see, rotations move about a fixed point in a circular motion. A real life example is a sunflower.
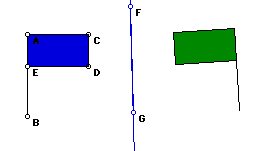
3. Translations: Triangle ABC, the preimage, is translated one inch by zero degrees.
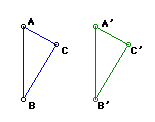
Triangle DEF, the preimage, is translated by 45 degrees and one inch.
A real life example of a translation is the girl scout emblem.
Questions
part 1
1. Give two real life examples of translations.
2. Give two real life examples of rotations.
3. Give two real life examples of reflections.
part 2
Identify the transformations shown below.
1.________________________
2.________________________

3.___________________________

Using Geometer's Sketchpad, construct your own reflection, rotation, and translation. Print your results out and have them ready for discussion in class tomorrow.
Days Three and Four: Reflections
Goal one: You should be able to use properties of reflections.
Goal two: You should be able to relate reflections and line symmetry and be able to construct these.
Goal three: You should be able to recognize reflections in real-life.
First, we will discuss their results from GSP (we want students to give actual construction techniques and problems that they ran into or even what they found easy to construct).
Look at line segment AB. Line segment AB is our line of symmetry. It can also be called the line of reflection. Another way to think about the line of reflection or in this case AB, is the body of a butterfly. The body of the butterfly is the line of reflection because it is the line that divides the two wings. One wing is the reflection of the other wing.

What do you suspect will happen if there is a point on AB and we reflect by AB.
Let's see.
Notice that the point stays in the same location.


Now try this activity with a sheet of paper. Draw any line segment. This will be your line of symmetry. Construct a point anywhere on this line. Now fold the paper along the line of symmetry. Notice that your point stays on that line.
Now let's see what happens when a point is not on the line. Let's examine our line segment AB again. This time point C is not on the line. Notice its reflection.
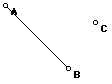

Point C is reflected along line segment AB to form C'.
Notice what happens when we connect C and C'.

AB perpendicularly bisects line CC'. This means that the distance from C to AB and C' to AB is congruent. This also means that C intersects AB in such a way that a perpendicular is formed.
This is not a coincidence!! When reflecting any point P through any line L: 1. If P is not on line L, then L is the perpendicular bisector of the line that is formed between P and its reflection.
2. If P is on line L, then P is the same as its reflection.
Now it is your turn. Construct two examples of reflection a figure through a line of symmetry. Then form a line from the preimage to the image ( like the above line formed from C to C'. Reflect and analyze your results for all constructions. Make sure to notice that your line of reflection perpendicularly bisects the line from your preimage to your image.
You should take notes on these steps before entering GSP!!!
How to construct a reflection using GSP:
step 1 : Construct any figure any way you like.
step 2 : Construct a line of reflection by constructing a line segment.
step 3 : Highlight or "click on" this line of reflection and go under the transform function and select "mark mirror."
step 4 : Highlight all points and segments on the preimage and also highlight the line of reflection.
step 5 : Go under transform again and select reflect. Your image should appear!
Days Five and Six: Rotations
goal 1 : You should be able to use properties of rotations.
goal 2 : You should be able to relate rotations and rotational symmetry.
goal 3 : You should be able construct rotations on GSP.
Below is an actual rotation. Point I is the center of rotation. Notice the distance from I to F is the same as the distance from I to F'. Notice the distance from I to F is the same distance as the distance from I to F''. Notice the distance from I to F is the same as the distance from I to F'''. In general the distance from the point of rotation to a point on the preimage is the same as the distance from the point of rotation to the corresponding point on the image.
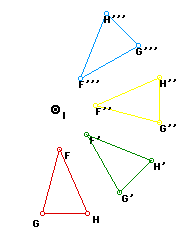
How many degrees has triangle FGH been rotated to get triangle F'G'H'?
We can find the angle using a protractor and straightedge or by using GSP. Using a protractor and straightedge we can make lines from F to I and from I to F'. This will form a measurable angle. We can also make lines form I to G and from I to G'. This new angle formed, GIG', will be the same as angle FIF'. These angles can easily be measured with a protractor.
To find the angle of rotation using GSP we first need to copy the figure. To copy, highlight the image you would like to copy, then go under edit and select copy. Now go to GSP and again go under the edit function and select paste special. Then click on OK. Construct lines FI and F'I.
Now highlight the points F, I, and F'. You want to highlight the points in the order that creates the angle you are looking for. Now that the points are highlighted, go under the measure function and select angle. This will give you the angle of rotation.
Find the angle of rotation from FGH to F'''G'''H'''.
Rotational Symmetry - A figure in the plane has rotational symmetry if the figure can be mapped onto itself by a rotation of 180 degrees or less.
An equilateral triangle has rotational symmetry. An equilateral triangle can be rotated 60 degrees and it will be mapped onto itself. ( The image will look the same as the preimage.)
Certain letters of the alphabet have rotational symmetry. For example: H, X, and S. Name three more letters that have rotational symmetry and tell how many degrees it needs to be rotated to give the same image.
Now its your turn ! Construct 3 examples of rotations. One rotation should have rotational symmetry. Another figure should have a rotation of 68 degrees. The last rotation can be rotated by any degree that you choose.
How to Construct a Rotation Using GSP :
step 1: Construct a figure any way you like.
step 2 : Click on or highlight all segments of the figure.
step 3 : "Click on" or highlight the point of rotation. Segments should remain highlighted.
step 4 : Now go to the transform function and select mark center.
( the transform function is found at the top of the screen)
step 5 : Then go under transform again and select rotate.
step 6 : You will be asked if you want to rotate by a fixed angle or by a marked angle. Select by fixed angle. The enter the angle you wish to rotate by and then select OK.
Days Seven and Eight: Translations
Goal one: You should be able to use the properties of translations.
Goal two: You should be able to use translations to solve real-life
problems.
Goal three: You should be able to construct translations using GSP.
Translation Facts
* A translation is an isometry that transforms by using vectors .
* A Vector is a force that moves points, lines, and figures a certain distance in a certain direction. For example, suppose we have a point p at (0,0), if our vector is <1,1>, then our point p moves to the right one unit and up one unit.
How to Translate
By a point: When translating a point, add the vector to the point in which you are translating. For example,
Preimage Vector Image
(2,1) + <1,3> = (3,4)
(-1,3) + <-1,2> = (-2,5)
By a figure: This is very similar to translations by a point. The only difference is that you translate each vertex by a vector. For instance, given triangle ABC where A is (2,1) , B is (0,0), and C is (3,0),translating by the vector <3,-4>, our new triangle (image) is A'B'C' where A' is (5, -3) , B' is (3,-4) and C' is (6,-4).
Now it's your turn! Using GSP, construct 3 examples of translations. One of these should be translated by the vector <-3,-2>. The other translation should be translated by the vector <-1,4>. For the last of these translations, you can choose the vector you want to translate by. After GSP shows your new images, make a printout and then show on paper how your new coordinates were found (use vector addition -- as shown above).
How to Construct a Translation Using GSP:
step 1: Create a figure any way that you want.
step 2: Highlight all points and segments in your figure, and choose the function transform.
step 3: Under transform, select translate.
step 4: Under translate, select "by rectangular vector". This will allow you to enter the first coordinate of your vector under horizontal and the second term of your vector under vertical.
step 5: Then click on "ok" and your image should appear.
More Explorations with Translations
In the following problems, please tell by what vector each quadrilateral has been rotated by.
1. What vector has the triangle DEF been translated by?
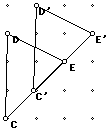
2. What vector has the parallelogram CDEF been translated by?
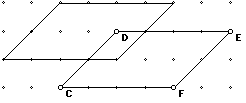
3. What vector has the pentagon FGHIJ been rotated by?
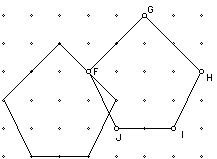
Day Nine: Test
1. .Give an example of where we see each of the following transformations in the real world.
Rotations:
Reflections:
Transformations:
2. Is this transformation an isometry? Why or why not?

3. Does a square have rotational symmetry? _________ If so, how many degrees must it be rotated in order to be mapped onto itself?_______
4. How many degrees have these triangles been rotated? ________
5. Take time now and construct your own rotation using GSP . Paste your result back to the space below. We want a scalene triangle rotated by 70 degrees. Color your pre-image green and your image yellow.
6. Reflect the letter A through the x-axis using GSP. Paste your results to the space below.
7. Using GSP, translate a triangle by the vector < 3, 1>. Paste your results below. Color your preimage light blue and your image black.
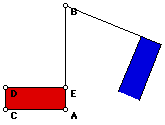
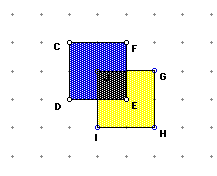
8. By what vector has the blue square been translated? _________
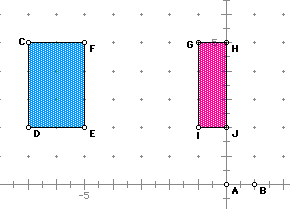
9. Using GSP, reflect a triangle by the y-axis. Paste your results below. Color your preimage red and your image green.
10. Is the light blue rectangle a reflection of the pink rectangle? Why or why not?