"Getting to know your calculator" lab
This lab is designed for you to become familiar with your calculators.
We will be using several in this class. The TI-34 is small but has many
capabilities. The more you know about it - the more confident you should
feel. Let's get started! Work in groups of two.
Activity One : Open up your calculator. Look at the keyboard layout.
Is there an on key? Why or why not? Record your results below.
.
.
.
Type in a number, say 420, and press the AC/ON key. What happens?
Type that same number again. This time press CE/C. What happens?
What can you say is the purpose of the two keys (record the results below).
You will probably want to get in the habit of using the CE/C key
more. You will see why later.
.
.
.
Let's try some simple operations to warm up. We are going to work on some
keys you already should know. Compute the following with your calculator
and record the results. (This will be a breeze!)
1) 123, 456 + 789, 012 =
2) 80, 000 - 456 =
3) 123.45 x 918.63 =
4) 189 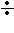 25.3 =
25.3 =
5) 6  0 =
0 =
6) 2 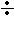 3 =
3 =
Check with your neighbors to see if your answers are the same. Look at number
5. What did you think the answer was going to be? Explain why you got the
answer you did below, maybe using a simple proof.
.
.
.
.
Look at your answer for number 6, work that one out by hand below. Do you
agree with the answer the calculator gives? Explain the difference between
the answers.
.
.
.
.
Activity Two: Now that you are figuring out some of the quirks of
the calculator and how to interpret the calculators results, let's try some
more.
First, you and your partner should discuss below how you could use the calculator
to answer the following:
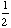 +
+ 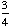
You are now going to learn how to add, subtract, multiply, and divide rational
numbers. Look for a key labeled a 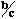 . We are going to enter
. We are going to enter
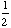 first. You need to first enter the numerator, next press the a
first. You need to first enter the numerator, next press the a 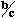 key, and finally enter the denominator. Draw below what comes up on
the
key, and finally enter the denominator. Draw below what comes up on
the
shows calculator's screen (it doesn't look exactly like what you are used
to):
.
.
.
Now you should be able to add  +
+ 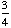 . Record your results below.
What do you think it means?
. Record your results below.
What do you think it means?
.
.
.
Your answer was a mixed number. Therefore, the calculator separates
the whole number from the fractional part by displaying a "_".
Now it is up to you and your partner to figure up how to add the following.
Write down the entire procedure you used to answer the following below so
you will have a reference for later (that means all the keys you used as
well):
1 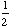 + 2
+ 2 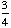
Procedure:
.
.
Now that you are an expert at using the "fraction" key, try the
following:
1) 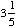 x
x 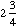 =
=
2) 
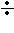
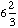 =
=
3)  x
x 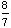 =
=
Check with your partner to see if you both agree with the answers. Can you
explain below why you got the answer you did for problem 2? What about problem
3? Could you have answered problems 2 and 3 without using the fraction calculator?
Answer below.
.
.
.
Activity Three: Now you are going to use the calculator to generate
patterns. You and your partner will decide how the calculator continues
the patterns.
First, enter 5 + 1 and press "=." Write the answer below.
Now press the "=" key again. Write down what you got next
to the first answer. Now press the "=" key repeatedly and
record the results below. What do you notice?
.
.
.
.
Next, enter 100 - 4 and press "=." Write the answer
below. Now press the "=" key again. Write down what you
got next to the first answer. Now press the "=" key repeatedly
and record the results below. What do you notice?
.
.
.
Write a brief statement below that describes how you can use the calculator
to generate a pattern of numbers.
.
.
.
Now see if you can continue the following patterns using the calculator.
1) 3, 5, 7, 9, ___, ___, ___, ___, ___, ___, ___, ___.
2) 246, 267, 288, 309, ____, ____, ____, ____, ____.
3) 1001, 991, 981, 971, ____, ____, ____, ____, ____.
We can also use the calculator to generate multiples. What is a multiple?
Define it below.
.
.
.
To generate all of the multiples of two we will start with zero and add
two. (Remember that zero is a multiple of all numbers.) If you continue
to press "=" you will get all the multiples of two. You should
have 0, 2, 4, 6, 8, 10, etc... Now you can generate the multiples of the
following numbers. (Remember to start with zero.)
1) Find the first ten multiples of 3.
.
.
2) Find the first ten multiples of 7.
.
.
3) Find the first ten multiples of 12.
.
.
Activity Four: Here is a good time to talk about a new key on your
calculator. Look for the key with the arrow on it. It looks like this "->".
I want you to enter a string of numbers to fill the screen. Now press the
arrow key. Press the arrow key again. What could you say the function of
this key is? When could it be of use to you? Explain below.
.
.
.
Activity Five: Now it is time to talk about rounding. You may need
to review by doing some rounding on your own.
Round the following and check with your partner to see if you agree:
1) Round 123.456 to the nearest tenth place.
2) Round 5.3481 to the nearest hundredth place.
3) Round 9.9999 to the nearest thousandth place.
4) Round 0.345 to the nearest whole number.
Did any of them give you problems? Which ones and why? Answer below.
.
.
.
.
The calculator has the capability to round. However, the task is a bit complicated
and you must still know how to round. It really comes in handy if your are
going to be entering a lot of data that needs to all be rounded to a specific
place value. Let us say we are working with our checkbook and want to round
all of our answers to the hundredth place. Keep in mind that monetary amounts
end in two numbers after the decimal place.
We can "fix" the calculator to round by pressing the blue bar
labeled "2nd." What appears on the screen when you press the blue
bar? What do you think the function of the blue bar is?
.
.
Now that you have pressed the blue bar, press the arrow key. (You should
look above the arrow key and written in blue will be the word fix.)
Now we have to tell the calculator how many places should be after the decimal
place. Since you are going to be rounding all of your answers to the nearest
hundredth enter the number 2. What shows up on the screen? Draw it
below.
.
.
The one thing you will have to remember is that you will have to use the
CE/C key to clear your screen from now on. Remember it was mentioned
earlier that there was a difference between this key and the AC/ON key.
Press the CE/C key now. What happens? Now press the AC/ON key. What
happens? Repeat the steps to have the calculator round and we will see what
happens. Don't forget to use the CE/C key to clear your screen from
now on.
.
.
Watch what happens when you enter the following number. The calculator has
been fixed to round all of the numbers you enter to the nearest hundredth
place. Enter the following and record the results.
1) $12.033 rounded to the nearest hundredth place is __________.
2) $12.875 rounded to the nearest hundredth place is __________.
3) $ 0.999 rounded to the nearest hundredth place is __________.
Now using what you have learned, see if you can have the calculator round
to the nearest tenth place. Remember how many numbers are after the decimal
place when you fix it to round. Round the following to the nearest tenth
place.
1) 12.05 rounded to the nearest tenth place is ___________.
2) 0.94 rounded to the nearest tenth place is ___________.
3) 0.99 rounded to the nearest tenth place is ___________.
Explain below how you and your partner would use the calculator to round
numbers to the nearest whole number. Test your hypothesis to see if it works
and explain the procedure below.
.
.
.
.
.
Activity Six: It is now up to you and your partner to work together
to reach a common answer. Decide the answer to the following problem. Explain
your answer below (agree on one answer):
3 + 6 x 6 -12/3
Answer:
.
.
.
Did you and your partner come up with more than one answer? We really have
to have consistency, and if you and your partner came up with different
answers than we do not. Try entering the problem in the calculator using
the following keystrokes. Fill in the blanks.:
3 + 6 = ____ x 6 = ____ - 12 = ____ / 3 = _____
.
.
Did you or your partner come up with that answer? Is that the right answer?
You will investigate one other way to enter the problem. Think about whether
you will get the same answer before you enter it. Enter the following problem
as follows:
3 + 6 x 6 - 12 / 3 = _____
.
.
Which key sequence do you think gives you the "correct" answer?
Can you explain why? Before you decide, you may want to think about order
of operations. (remember P.E.M.D.A.S)
.
.
.
Now try the following using your calculator:
1) 20 / 2 x 4 - 10 + 15 / 3 =
2) 17 - 3 / 2 + 6 x 3 =
Activity Seven: We are now going to explore another way to utilize
the calculator for a "shortcut." I want you to answer the following:
1) 7 x 7 =
2) 25 x 25 =
That was easy. However you had to use four keystrokes to come up with an
answer. Do you remember a shortcut way of representing n x n? You and your
partner should be looking on the keyboard for the exponent keys.
(There are two.) One will raise a number, x, to the second power. Write
down the key you think that is below.
.
.
The other key with raise any number, y, to any power x. Write down the key
you think that is below.
.
.
To do problems #1 and #2 from above, you can use either key. However, if
you are raising anything to the second power, it is much easier to use the
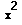 key. All you need to do is enter the
number, for example 7, and then press the
key. All you need to do is enter the
number, for example 7, and then press the 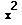 key. You
get the answer immediately. Try the following:
key. You
get the answer immediately. Try the following:
1) 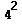 =
=
2) 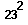 =
=
To raise any number to any power, you will need to use the  key. For example, if you wanted to solve
key. For example, if you wanted to solve  you would enter 7 - your base,
then press the
you would enter 7 - your base,
then press the 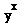 key. Then press 4, your
exponent,
key. Then press 4, your
exponent,
and finally press "=." You should get 2401. Create three exponent
problems on your own and record the three problems you tried along with
the answers below:
1)
2)
3)
Activity Eight: You are now going to play around on the calculator
and record your results. Be sure when you go to the next problem to clear
the screen using the AC/ON key to get rid of all functions. Write
down what happens when you do the following:
1) Press the blue bar labeled "2nd" and then the key marked EXP
with the symbol above it. What shows up on the screen?
_____________________________________
2) Enter the number 25. Press the blue bar labeled "2nd" and then
press the "=" key with the % symbol above it. What shows up on
screen?
_____________________________________
3) Press the blue bar labeled "2nd" and then the "."
key with the
symbol SCI above it. Now enter the number 36000 and press "=."
What shows up on the screen?
_____________________________________
4) Press the yellow bar labeled "mode" and then press the number
4.
(You should see the word HEX on it.) Now multiply 4589 by 3698.
What shows up on the screen?
_____________________________________
Activity Nine: Now you are going to learn about another key on your
calculator that can save you some time. However, I want you to see what
happens before we use the shortcut.
Word Problem: Suppose you start a new job making $4.75 an hour. If
you work 20 hours, how much money will you earn before taxes?
Solution: .
.
.
Another Word Problem: This week you worked 37 hours. How much
will you earn before taxes?
hours. How much
will you earn before taxes?
Solution: .
.
.
That wasn't bad. However, there is a way to enter your salary into the calculator
so that it is in its memory for a while. To do this you must look for the
keys labeled STO and RCL. STO is short for store. We
are going to store a number into the calculator's memory. To do this enter
the number you want to store, in our case 4.75. Now press store. What did
you see appear? What do you think it stands for?
.
.
**IMPORTANT**
Remember at the beginning it was discussed that we could use two keys to
clear the computer screen. We are going to use the CE/C key so it
will not erase your number. You may press that key now.
Now that the number is stored, to recall that number you will have to press
the RCL key. Press that key now. What showed up on the screen? Press
the CE/C key.
We can use that stored number to create a table of hours worked and money
earned. To find out how much you will make enter the number of hours multiplied
by the RCL key.
EX) If you worked 5 hours: enter 5
press the X (times) key
press the RCL key
press the = key.
What did you get?__________
Now complete the following chart:
Hours worked | money earned
1
2
3
4
5
6
7
8
9
10
11
12
13
14
Congratulations, you are now an expert at using the TI-34 calculator. There
are still many more applications for this calculator that you will
learn. These pages will serve as your reference for later so do not lose
them!