Centers of a Triangle
|
||
|
Play with the files above for a while. It looks like the medians of the triangle in the Centroid file meet in a single point, the altitudes of the triangle in the Orthocenter file meet in a single point, and the perpendicular bisectors of the triangle in the Circumcenter file meet in a single point. Can we prove this?
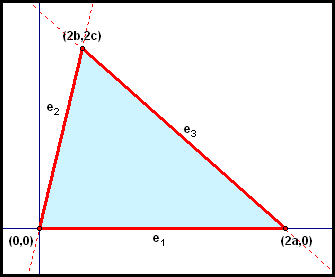
First, we need a way to describe a general triangle. Given any triangle, place coordinate axes on it so that one edge rests on the x-axis, and one vertex lies at the origin. Label the other two points (2a,0) and (2b,2c), and label the edges e1, e2, and e3, as shown:
By wisely choosing where we put the origin and onto which edge we put the x-axis, we can assume (without loss of generality) that a, b, and c are all nonnegative. In addition, note that if c = 0, we have a degenerate triangle in the form of a line along the x-axis, and if a = 0, we have a degenerate triangle in the form of a line segment along the y-axis. So we can assume that, if we are in the case of a nondegenerate triangle, we have a > 0, b ≥ 0, and c > 0. Now that we have a way of describing the triangle, let's show that the centroid, orthocenter, and circumcenter are well-defined points. That is, let's prove that the three lines defining each actually meet in a single point (a different point for each). Click here to continue.
| ||