Tangent Circles
by Emily Kennedy

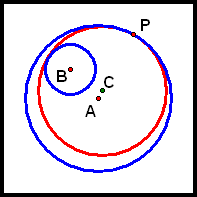
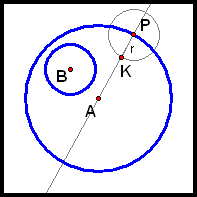
Consider two circles--one with center A and radius R (call it Circle A), and another with center B and radius r (call it Circle B).
Without loss of generality, say R ≥ r.
Also, assume Circles A and B are not tangent to each other.

Let P be a point on Circle A.

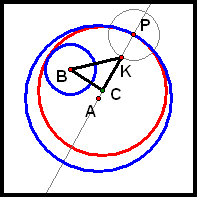
We want to construct a circle, Circle C, that is tangent to Circles A and B such that Circle B lies entirely inside Circle C, like this:

Let C be the center of this tangent circle, and let Q be its point of tangency with Circle B (we will have to determine the locations of Q and C).

Then and are radii of Circle C, so we must have CQ = CP. (*)

Let K be the point on such that AK = R - r and KP = r.

Then, from (*), we have

CQ = CP
 CB + BQ = CK + KP
 CB + r = CK + r
 CB = CK

So ΔCBK is isoceles:

Thus, C lies on the perpendicular bisector of .

So the tangent circle we want is simply the circle through P,
with center C.

In this GSP file, you can trace the center C of the tangent circle as P moves around Circle A. What do you see? Move Circles A and B around and adjust their radii. Try as many cases as you can think of, and make some conjectures before clicking here to continue.

Next (Loci) >>

Return to my page
Return to EMAT 6680 page