HamiltonHardisonÕs
Exploration of
Assignment 2: An Exploration of a Quadratic Relation
From Jim WilsonÕs Assignment
Page: Graph 
. Now, on the same axes, graph 
Describe
the new graph. Try different coefficients for the xy term. What kinds of graphs
do you generate? What coefficients mark the boundaries between the different
types of graphs? How do we know these are the boundaries? Describe what happens
to the graph when the coefficient of the xy term is close to the boundaries.
It is clear that the graph of 
is a circle.
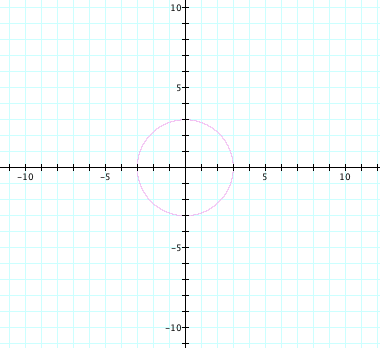
Graphing 
gives an ellipse whose major axis
appears to be on the line 
.
By graphing 
for n = .2, .4, .6, É , 1.6, 1.8, We see that we have a series of ellipses. Each has itÕs major axis lies along the
line 
. As n increases, the ellipse becomes longer and thinner.
When n=2, we have a very different graph.
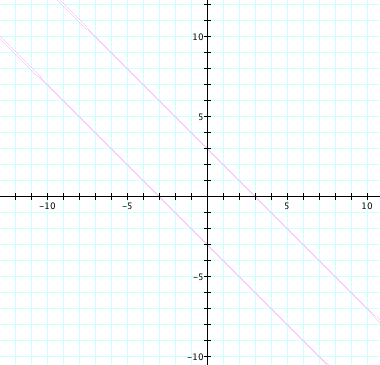
This appears to be two parallel lines.
Algebraically we have: 
By solving for (x+y); We have 
or 
. This confirms the conjecture that the
graph is no longer an ellipse, but two parallel lines.
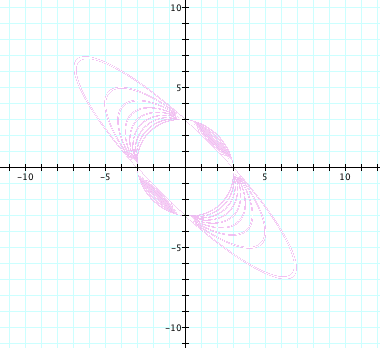
As the coefficient of the xy term moves beyond 2; we
notice a different class of graphs. The graph of 
for n = 2.2, 2.4, 2.6, É , 3.6, 3.8 is shown below.
Each curve appears to be a hyperbola whose foci lie
along the line 
. As n gets further away from 2, the curvature of the graph
becomes more exaggerated. By examining
the graph of 
, we can
somewhat safely determine that all coefficients of the xy term greater than 2
will produce hyperbolas. (Note the
difference in scale)
If negative values are
considered, the results can be summarized in the graph and table below:
|
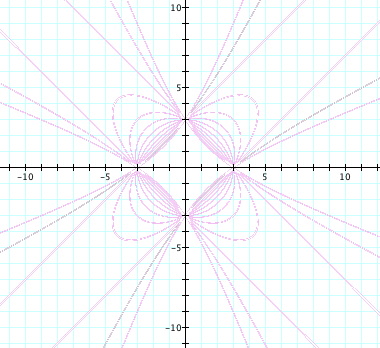
n<-2 |
Hyperbola |
|
n=-2 |
Parallel Lines |
|
-2<n<0 |
Ellipse |
|
n=0 |
Circle |
|
0<n<2 |
Ellipse |
|
n=2 |
Parallel Lines |
|
2<n |
Hyperbola |
Returnto
Hamilton Hardison's Page