There are many, many proofs of the Pythagorean Theorem. I will not even being to attempt to replicate some of those here. If you are interested in explanations of some of the many proofs, I found a website with 98 proofs: http://www.cut-the-knot.org/pythagoras/index.shtml. I believe that should satisfy your curiosity.
On this page, I am interested in detailing an alternate investigation with the Pythagorean theorem that certainly piqued my interest. I was exposed to this in a class taught by Dr. Ryan Smith.
I am sure many of you are familiar with the Pythagorean Theorem:
. Along this comes one of the literal interpretations: that the sum of the areas of the squares on the two shorter sides (legs) will add up to the area of the square that is built on the hypotenuse.
BUT, have you ever thought about if Pythagorean theorem would hold if we constructed different shapes on the sides of the triangle???
For instance, what if we constructed semicircles on the legs of the triangle? Would the sum of the areas of the semicircles built on the smaller two legs be the same as the area built on the hypotenuse? What does intuition say? How would you prove your assertion?
What about other shapes? Triangles? Rectangles? Pentagons? Hexagons? If you want to explore this for yourself first, you can use my GSP file here.
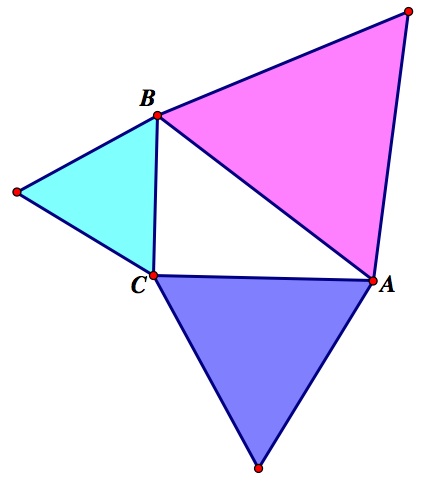
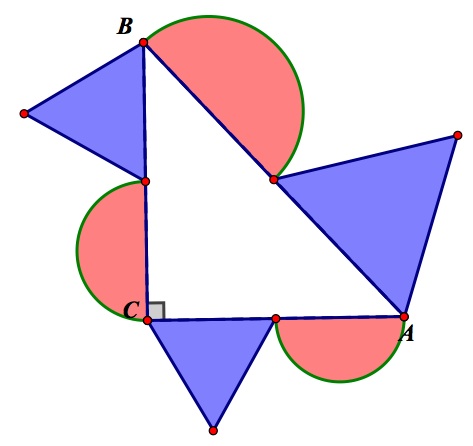
It actually works! But now, the question is WHY. I will show some proofs below as to why this works. But, first lets remember or think about what it is we are trying to prove. We started with the Pythagorean Theorem,
First, for the equilateral triangle. To find the area of a triangle, we take the product of the base and height and divide it by two. The base is obviously the side of the triangle that we are constructing the equilateral triangle on. But the height is a little more difficult to find. Using prior knowledge of special right triangles, we get the following diagram and thus the height of the triangle.
Now, we will try to show that the Pythagorean theorem relationship holds for equilateral triangles.
As you can see, when we take into account the areas of the equilateral triangles, we still end up with
For semicircles...
For rectangles... (here I chose to make the lengths twice the width, but any fixed relationship would work)
And for pentagons ... This is a little more tricky or involved just because the area formula is not as simple. First, you would need to know the interior angles of a pentagon and then some trigonometry to find the apothem, or the height of the triangle. The diagram below should help.
Due to my choice in formula for the area, this approach for the pentagon will actually work for any n-gon. So, if we wanted we could also construct hexagons, octagons, decagons, and so on.
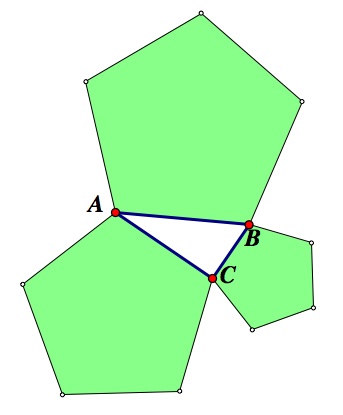
What about any similar figures? Figures that are not regular n-gons?
Figures composed of two shapes above Random Figures
The short answer is YES it does still work! With GSP, we can measure the areas and see that it still works.
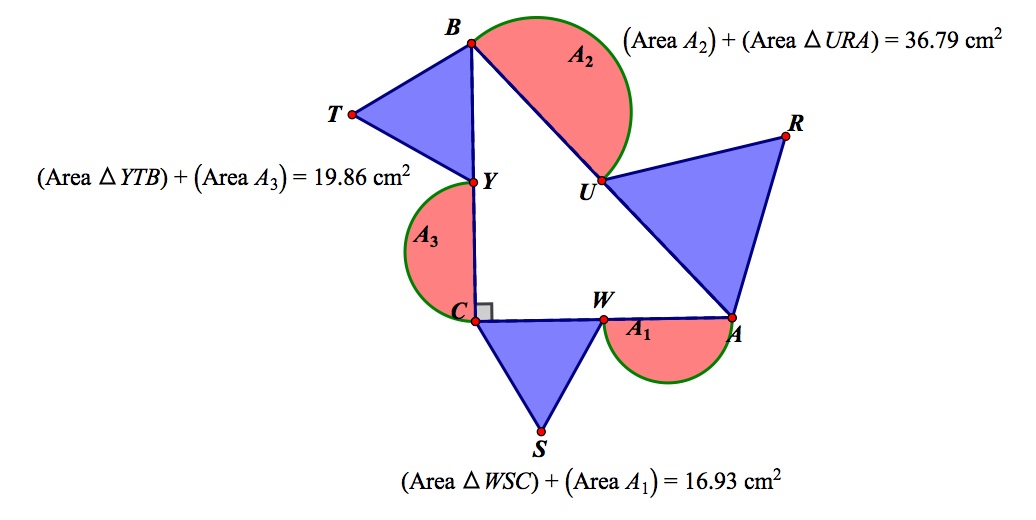
But does it makes sense that it does? After typing out the solutions above for each of the shapes, it does make sense to me. In each case, there was a similar scale factor being applied to a, b, and c. In the end, we were able to factor out that scale factor and we were left with
