Proof:
The locus of the center of the common tangent
circle to two other circles is a hyperbola.
In some cases, the locus of the center of the common tangent
circle forms a hyperbola. One of these cases occurs when the two
original circles are disjoint and the smaller circle is external
to the common tangent circle. See the picture below.

The definition of a hyperbola is the set of all points (x,y)
in the plane such that the difference of the distances from (x,y)
to two fixed points is some constant. The set of all points (x,y)
refers to the path of points formed by the center of the common
tangent circle, which I will call C. So, we must show that the
difference of the distances from C to two fixed points is some
constant to prove that the locus is hyperbola.
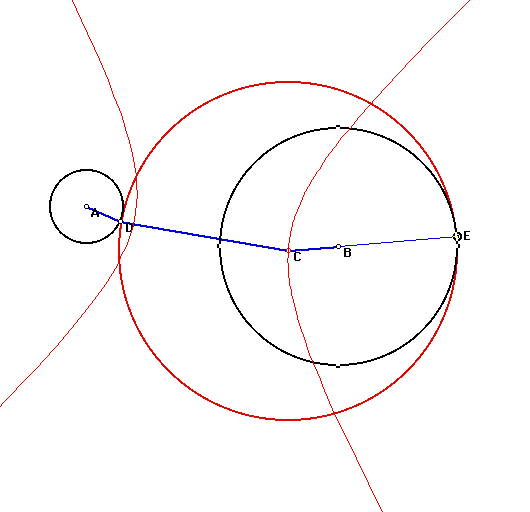
Let circle A and circle B be the two original circles. A and
B are both fixed points as the centers of the two original circles.
The red circle C is one of the common tangent circles to both
circle A and circle B. Let AD be the radius of the small circle
A, and BE be the radius of the large circle B.
Two radii of the common tangent circle C are CD and CE. Thus,
CD = CE. The segment CE = CB + BE. By substitution, CD
= CB + BE.
The segment CA = AD + CD by segment addition. Thus, CD = CA - AD.
We can set the two above equations equal to each other, since
both are equal to CD. Hence, CB + BE = CA - AD. Rewritten: BE
+ AD = CA - CB. BE + AD is the sum of the radii of the two original
circles, which is a constant. CA - CB is the difference of the
distances from C to two fixed points. Thus, the difference of
the distances from C to two fixed points is some constant. Therefore,
the path of points formed by C as E moves around the large circle
is a hyperbola.