Assignment 10

Parametric Curves

by

Behnaz Rouhani

A parametric curve in the plane is a pair of functions

x = f (t)

y = g (t)

where the two continuous functions define ordered pairs (x,y). The two equations are usually called the parametric equations of a curve. In this assignment we will investigate several of these curves.


 

This assignment is divided into three parts:

I. First we will explore the equation


II. For the second part we will investigate the parametric curve

for different values of a and b.

III. Special explorations

Case I: Let us explore the equation

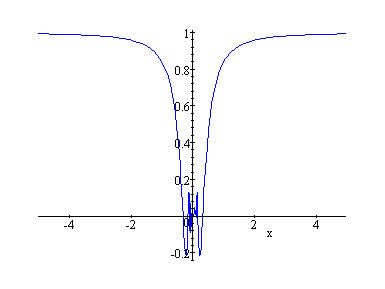
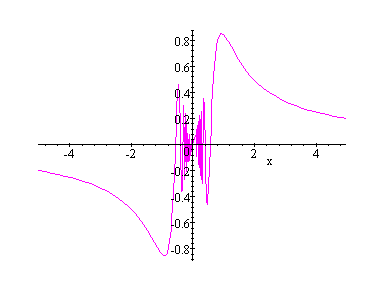
We will set to demonstrate what happens to the curve of the parametric equation as the exponent for t increases. While viewing the following curves do you see an interesting phenomenon?

Part A:


 

Part B:



 

Part C:


 
 

Part D:


 
 


Case II: We will investigate the parametric equations

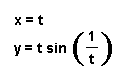
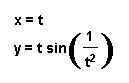
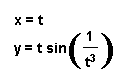
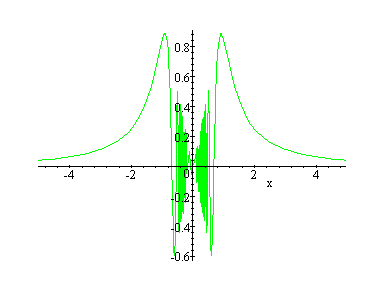
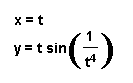
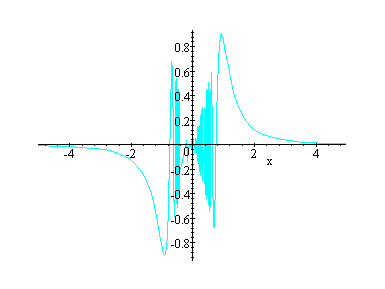
x = cos (at)

y = sin (bt)

for different values of a and b.
 

Part A: a < b, a = 1

(cost, sin2t) --- Red

(cost, sin3t) ---- Green
 
 

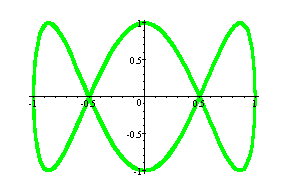
Observation: When a =1, the number of hoops is the same as b.
 

Part B: a < b, a = 2

(cos2t, sin3t) --- blue

(cos2t, sin 4t) --- magenta

(cos2t, sin8t) --- Navy blue


 

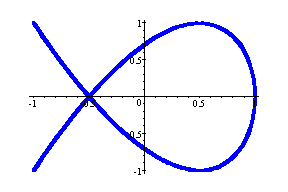
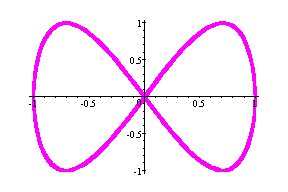
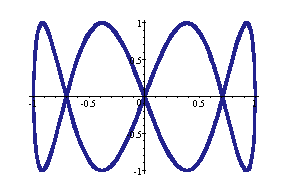
Observation: When a = 2, the number of hoops is the same as b/2.
 

Part C: a < b, a =3

(cos3t, sin4t) --- Red

(cos3t, sin7t) ---- Magenta
 
 

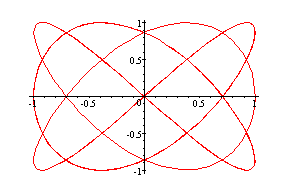
Observation: When a = 3, the number of hoops is the same as b.
 

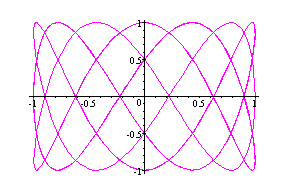
Part D: a < b, a = 4

(cos4t, sin6t) --- Green

(cos4t, sin8t) --- Yellow
 
 

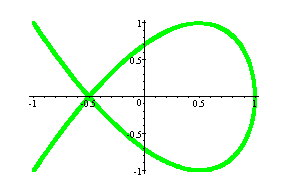
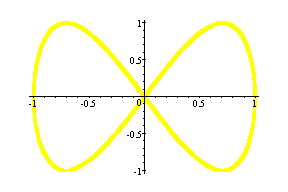
These graphs are similar to graphs of (cos2t, sin3t) and (cos2t, sin4t) respectively. Can you tell why we get the same graphs?
 

Part E: a < b, a = even number

(cos4t, sin5t) --- Brown

(cos6t, sin7t) --- Green

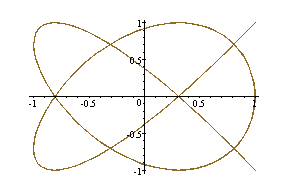
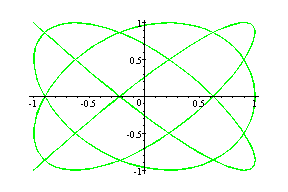
What kind of conjecture could we make about this case?

Part F: a > b, b = a/2

(cos2t, sint) ---- cyan

(cos10t, sin5t) ---- brown
 

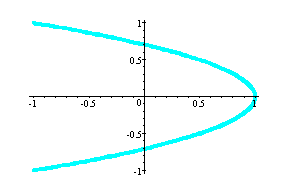
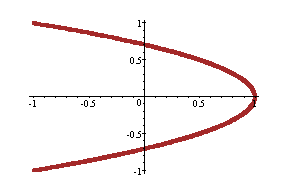
Observation: As long as coefficient of b is half of a, the graph is a parabola with vertex at (1,0).
 

Part G: a > b, a = even number, b = odd number , difference between a and b is one

(cos4t, sin3t) --- cyan

(cos6t, sin5t) --- Brown

(cos14t, sin13t) --- Blue
 

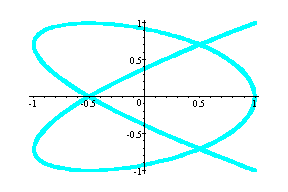
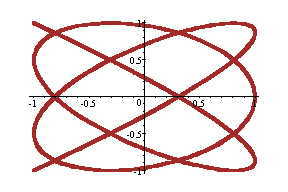
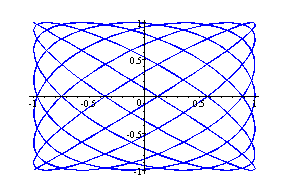
Observation: As the value of a and b increases, with a = even number, b = odd number and the difference between a and b is one, the graphs seem to be getting filled up.

Part H: a > b, a = odd number, b = even number, difference between and b is one

(cos3t, sin2t) --- Green

(cos5t, sin4t) --- Red

(cos7t, sin6t) --- Blue

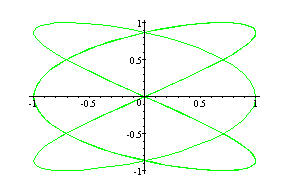
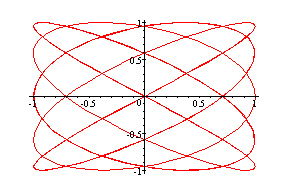
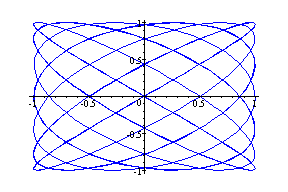
This interesting pattern occurs when simple curves are displayed as the "envelope" of a more complicated function. In practice such things happen when low frequency waves ride carrier waves broadcast from a radio station.

Notice the two blue graphs in part G and H, don't they look almost alike?

Part I: a > b, a =odd number, b = odd number, and difference between a and b is more than one

(cos7t, sin3t) --- Blue

(cos11t, sin3t) --- Green

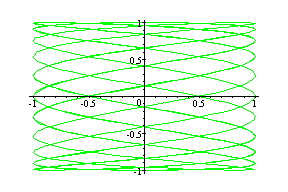
Observation: Number of hoops in each case is the same as a.

Case III: Special Investigations

We will try to explore the following special parametric curves.

Part A:


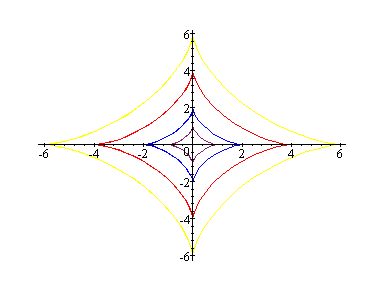
This is the graph of Astroid for different values of a = b. Here is the color coding for the graph.

Maroon graph: a = b = 1

Blue graph: a = b = 2

Red graph: a = b = 4

Yellow graph a = b = 6
 

Part B:

( t - sint, 1 - cost)


 

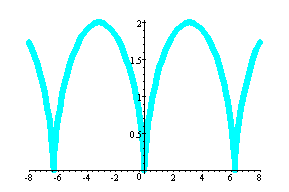
This is the graph of Cycloid.

Return to Behnaz Rouhani's page