By definition, a parabola is a set of points equidistant from a given point (called the focus), and a given line (called a directrix).
In this assignment we will attempt to construct a parabola given a fixed point for the focus, and a line (segment) for the directrix.

In order to build this parabola, we need to construct a point on the directrix. Then, construct a perpendicular line to the directrix through that point.

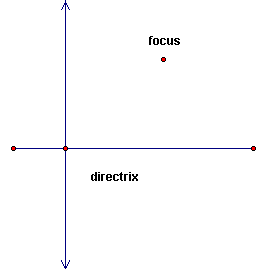
Next, we will need to construct a segment from focus to the point on the directrix. Then, construct the midpoint of that segment.

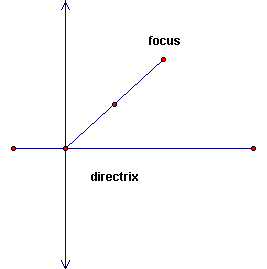
The next step is to construct the perpendicular line to the segment we have created. Subsequently, construct the point of intersection of the first perpendicular line with the second one (called a).

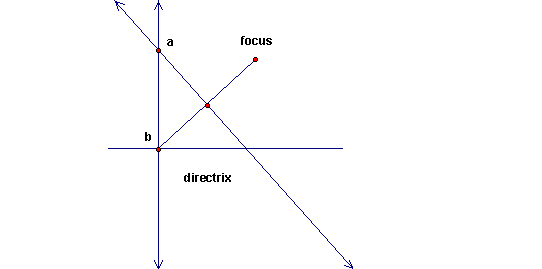
To get the parabola we could trace the point we just created (point a), and move point b along the directrix.

Or, we could create an action button to generate the parabola from an animation and trace of the constructed point a. Click here for a GSP file to view and explore.

Another approach to creating the parabola is to trace the tangent line of the parabola, and see what happens as the point b moves along the directrix. Click here for the GSP file to view and explore.

Further, we could use the locus command to generate the parabola. To do so we need to select points a & b then go to GSP menu and choose locus. Click here for a GSP file to view and explore the locus.