Tangent Circles

by Oktay Mercimek

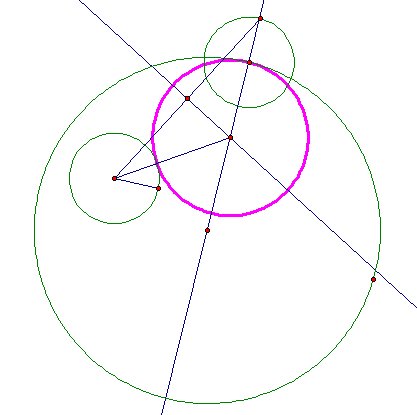
How to find purple tangent circle is described at http://jwilson.coe.uga.edu/EMT668/Asmt7/EMT668.Assign7.html and this is the gsp file for sketch Tangent Circle.

To make thi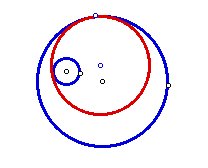s tangent circle we need to find a different way. Let's see what we know, we look for a tangent circle that is tangent to both circles.

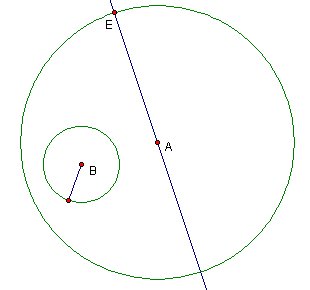
We need to start with two circles that one of them is in the other one.

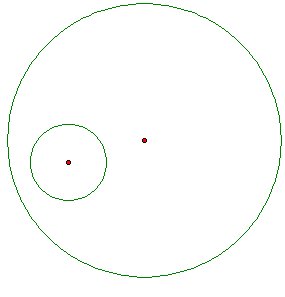
I believe we need very simple information. Firstly, remember the description of circle tangency. If two circles are tangent then they touch each other at one point that is on the line connecting two circles centers. see picture below.

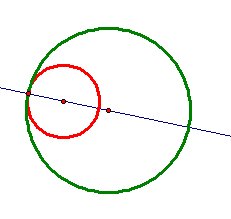
The circle that we look for must be tangent to each other.

Blue circle touches the small and the large circle at one point.

Point E must be on line CA, and point D must be on line BC.

here we know

|EC|=|DC| and we don't know exactly where to put point C. Since we don't know point C, we also don't know where the point D.

This is the picture we know,

 We know there is a ECD isosceles triangle. We don't know ED side but we know DB and we know point E

If |EF|=|BD| then |BC|=|FC| , and ECD and FCB are similar triangles. (S.A.S)

In this case point C is on perpendicular bisector of FB.

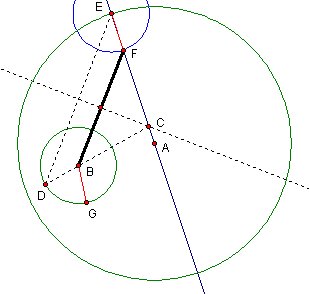
We have found the place of point C then we can draw tangent circle. The circle centered at point C and radius |CE|. You can download GSP file of this picture.

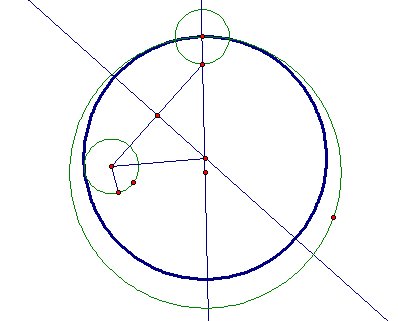
Let's use the tool "Tangent2" from last GSP file "TangentCircle2.gsp" and Place the small circle completely outside of large circle.

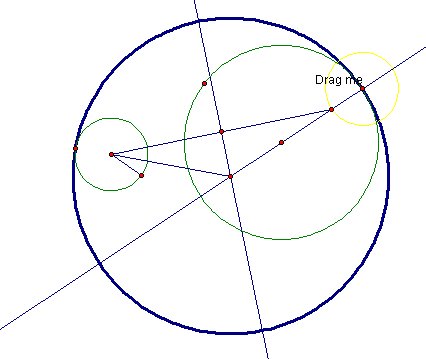
Surprisingly it works. It works for ALL situations. The picture above is the one of the four situations.

The second situation is the picture below

.

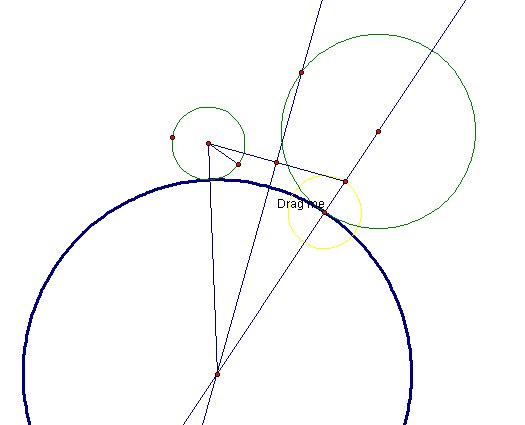The third situation is this one

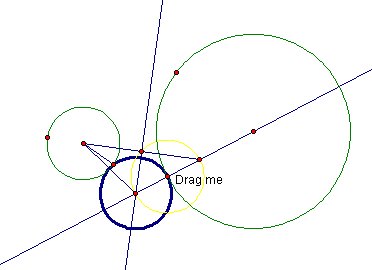
and the last situation is almost same as second one

.

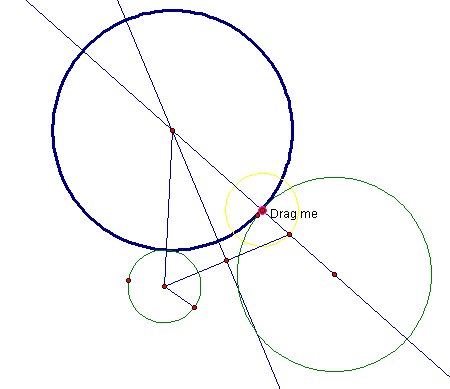So our construction also holds for this new situation.