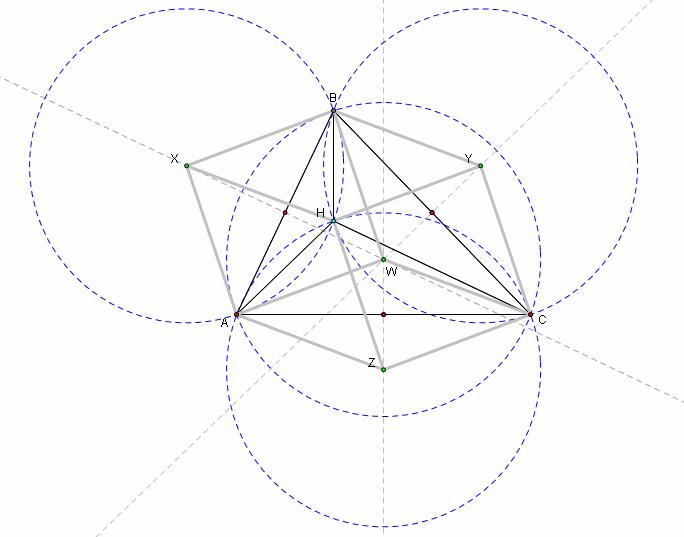
Michael Thomason's write-up for assignment eight.
Here is a triangle ABC with its
orthocenter, H.
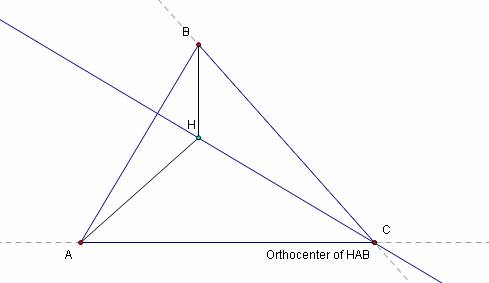
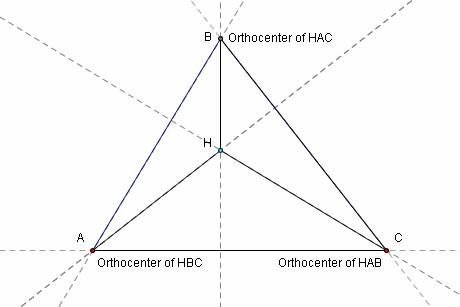
Here is the same situation with the orthocenter of HAB drawn. The orthocenter of HAB is equal to C. Click the picture and manipulate the triangle, noting that the orthocenter of HAB remains at C.
Now I will
draw in the orthocenters of HBC and HAC, which are equal to A and B, respectively.
Again, you may click the picture to manipulate it in GSP.
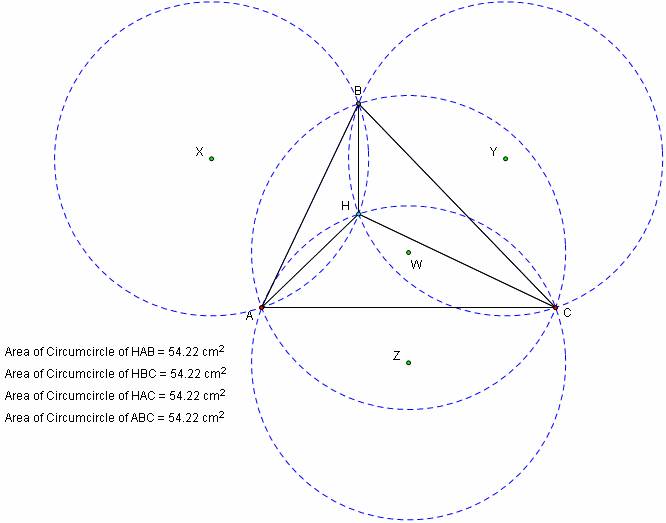
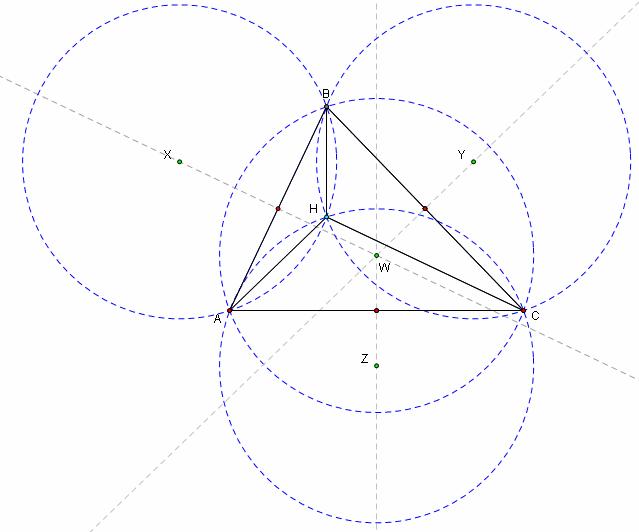
After removing the dashed lines, I have found the circumcircles of the four triangles mentioned. Again, you may click the picture to manipulate it in GSP. Note that the areas of each of the circumcircles are equal.
If each of the
circumcircles always has equal area, then they have equal radii, i.e. 
. We already know that 
because W is the
center of the circumcircle of triangle ABC.
By
construction, X and W both lie on the perpendicular bisector of 
(The center of a
circumcircle for a given triangle lies at the intersection of the triangle’s
perpendicular bisectors (click here
for more on this). Triangles ABC and ABH share a common side and therefore a
common perpendicular bisector. It is on this common perpendicular bisector that
X and W lie.). Similarly Y and W both lie on the perpendicular bisector of 
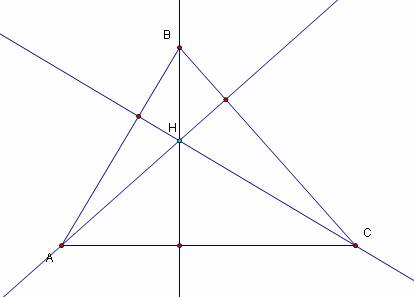
, and Z and W both lie on the perpendicular bisector of 
. The drawing below shows the perpendicular bisectors of ABC.
It appears
that not only do X and W lie on the perpendicular bisector of , but also that A and B lie on the perpendicular bisector of 
. This means that 
and 
. 
(because X is the
center of the circumcircle of triangle HAB), so 
. Similarly, the other segment lengths shaded gray below are
all equal, and the four circumcircles are all equal in area. Click the picture
to manipulate the two-dimensional representation of a cube. Click here to manipulate it with everything else hidden.
