Ceva's Theorem
Amena Warrayat
*Please maximize browser to properly view the essay*
This essay will focus on extending the topic of centroids discussed in EMAT 6680, Assignment #4.
Please click here to view the EMAT 6680 centroid write up.
Jump to Section:
I. History
II. Statement and Proofs of Ceva's Theorem
III. Corollaries of Ceva's Theorem
IV. An extension to Ceva's Theorem
V. Incorporating technology to teach Ceva's Theorem
I. History
Giovanni Ceva was a mathematican born in 1647 in Milan, Italy and is well known for publishing Ceva's Theorem in 1678. Ceva was educated in a Jesuit college in Milan and then at University of Pisa, where the work of Galileo Galilei and his followers on geometry and mechanics had a great influenced on Ceva's education and research interests. His own theorem essentially states that if three line segments are drawn from the vertices of a triangle to the opposite sides, then the three line segments are concurrent, if and only if, the product of the ratios of the newly created line segments on each side of the triangle is equal to one. Ceva also rediscovered and published Menelaus's theorem and worked on hydraulics.
II. Statement and Proofs of Ceva's Theorem
If three Cevians AE, BF, and CD are concurrent at G, then 
.
First proof (referenced from assignment #4):
Notation: A(AGF) = Area of triangle AGF.
Now, we assume that the Cevians AE, BF, and CD are concurrent at G. We will show that
Looking at triangles AGF and CGF, we see that segment GF is the height for both of these triangles. Call this height h.
Second proof of Ceva's Theorem:
Refer to the following figures:
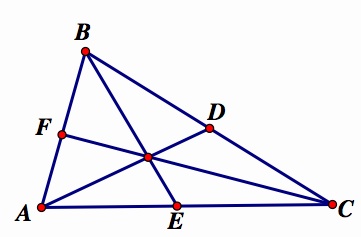and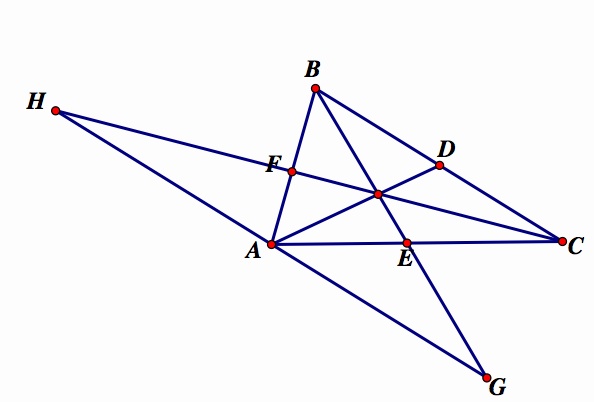 
Next, consider that instead of proving Ceva's Theorem using areas, we could do use by extending the line of AG.
Extend the lines of BE and CF beyond the triangle until they meet at GH, the line through A parallel to BC. There are several pairs of similar triangles: AHF and BCF, AEG and BCE, AGK and BDK, CDK and AHK. From these and in that order we derive the following proportions:
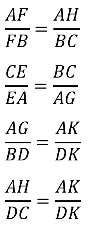
Then, from the last two equations, we conclude that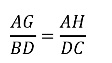 
and, hence, 
.
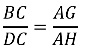Multiplying the first two equations, we get: 
Therefore, if the lines AD, BE, and CF intersect at a single point K, the equation for Ceva's Theorem holds. Which is to say that the fact of the three lines intersection at one point is sufficient for the equation of Ceva's Theorem. Now, let's show this statement is also necessary. i.e. If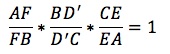 
is true, then AD, BE, and CF are concurrent.
So, assume that K is the point of intersection of BE and CF and draw the line AK until its intersection with BC at a point D'. Then, from the proved part of the theorem, it follows that.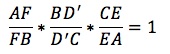
However, it's given that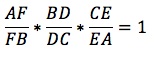 
. Setting these two equations equal to each other, we have that
.
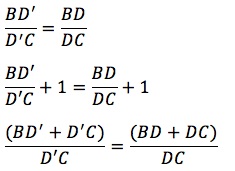Which gives that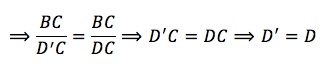 
. Therefore, D' and D are the same point. Q.E.D.
III. Corollaries of Ceva's Theorem
The cevians of triangles, such as medians, perpendicular bisectors, altitudes, and angle bisectors, intersect at a point of concurrency and can be proved using Ceva's Theorem. In this section, the statement and justification of each corollary is provided.
Statement 1: Medians of a triangle intersect at a point of concurrency.
Justification:
Let AE, BF, and CD be medians of the triangle ABC. Since the medians connect vertices to the midpoints of the edges of a triangle at opposite sides, we see that.
So each of the ratios are 1 and therefore so is their product. Using the converse of Ceva's Theorem, we have that the medians AE, BF, and CD are concurrent. Q.E.D.
Statement 2: Perpendicular Bisectors of a triangle intersect at a point of concurrency.
Justification:
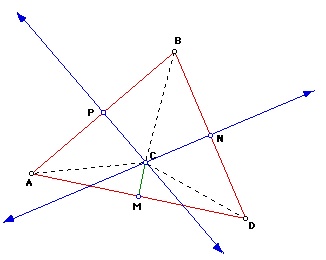
In triangle ABD, let the perpendicular bisectors of side AB and side BD intersect at point C. Now, construct a line segment from point C to side AD such that segment CM is perpendicular to side AD.
Triangles APC and BPC are congruent by the Side-Angle-Side Congruence Postulate. So segment AC equals segment BC. Similarly, triangles BNC and DNC are congruent by the Side-Angle-Side Congruence Postulate. So segment BC equals segment DC. Therefore, segment AC equals segment DC.
Thus, triangles AMC and DMC are congruent; hence segment AM equals segment MD. This shows that line MC is the perpendicular bisector of side AD.
This implies AC = BC = DC and any circle with the radius of AC will pass through all three vertices of triangle ABD. Q.E.D.
Statement 3: Atitudes of a triangle intersect at a point of concurrency.
Justification:
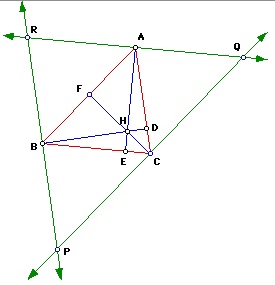
Let triangle ABC have the altitudes (as shown above) AE, BD, and CF.
Through each of the vertices A, B, and C, construct a line parallel to the opposite side. For instance, line RQ is constructed through vertex A and is parallel to segment BC.
Similarly, line RP is constructed through vertex B and is parallel to segment AC and line PQ is constructed through vertex C and is parallel to BA.
Thus, triangle PRQ is formed.
Note that segment RA equals segment BC since RACB is a parallelogram. Similarly, segment AQ equals segment BC since ABCQ is a parallelogram.
This implies that segment RA equals segment AQ.
Segment AE is perpendicular to segment RQ since segment segments BC and RQ are parallel. This implies that segment AE is the perpendicular bisector of segment RQ.
Similarly, BD is the perpendicular bisector of RP and CF the perpendicular bisector of QP.
Thus, AE, BD, and CF are concurrent since perpendicular bisectors of a triangle intersect at a point of concurrency called the circumcenter. Q.E.D.
Statement 4: Angle bisectors intersect at a point of concurrency.
Justification:
Suppose we are given a triangle ABC. Construct the angle bisectors of angle BAC and angle ABC. See below for a pictorial representation. We let K represent the intersection of these two angle bisectors.
From the point K, construct segments that are perpendicular to each of the three sides of the triangle. We labeled the intersection points on sides BC, AC, AB as D, E, and F respectively.
We have that triangles BDK and BFK are congruent by AAS since the following is true:
1) angle BDK = angle BFK since they are both right angles.
2) angle FBK = angle DBK since BK is the angle bisector.
3) BK = BK due to the reflexive property
Since corresponding parts of congruent triangles are congruent, we have now that FK = DK.
Similarly, we have that triangles AFK and AEK are congruent by AAS because:
1) angle AFK = angle AEK since they are both right angles.
2) angle FAK = angle EAK since AK is the angle bisector.
3) AK = AK by the reflexive property.
Since corresponding parts of congruent triangles are congruent, FK = KE. As a result, FK = KE = KD.
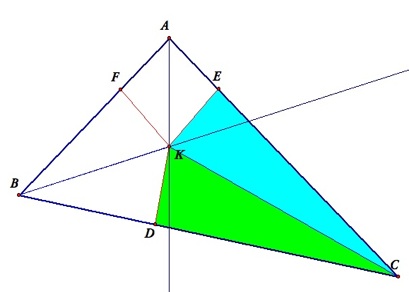
We also have that triangles CDK and CEK are congruent by Hypotenuse-Side because the following is true:
1) KE=KD
2) KC = KC due to the reflexive property
3) angle KDC = angle KEC since they are both right angles.
Since corresponding parts of congruent triangles are congruent, angle DCK = angle ECK.
Thus, the angle bisectors of the interior angles of the triangle are concurrent.
IV. An extension to Ceva's Theorem
An extension of Ceva's Theorem includes Kiepert's Hyperbola, which is the locus of points connecting the incenter to the orthocenter. Kiepert Hyperbola's is a solution to Lemoine's problem.
Lemoine's Problem essentially states that given the vertices of the equilateral triangles placed on the sides of a triangle, construct the original triangle. See the figure below for a pictorial representation. In this particular case, Lemoine's Problem involves providing vertices D, E, and F, and is seeking vertices A, B, and C.
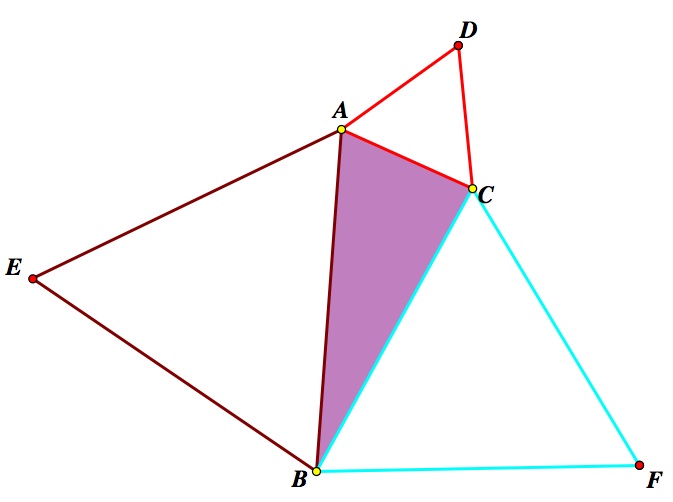
Kiepert's solution involved these steps (brief outline):
1) If on the three sides of an arbitrary triangle ABC, one describes equilateral triangles ABE, ACD, BCF, then the line segments AF, BD, CE are equal, they concur in a point P, and the angles they form one another are equal to 60°.
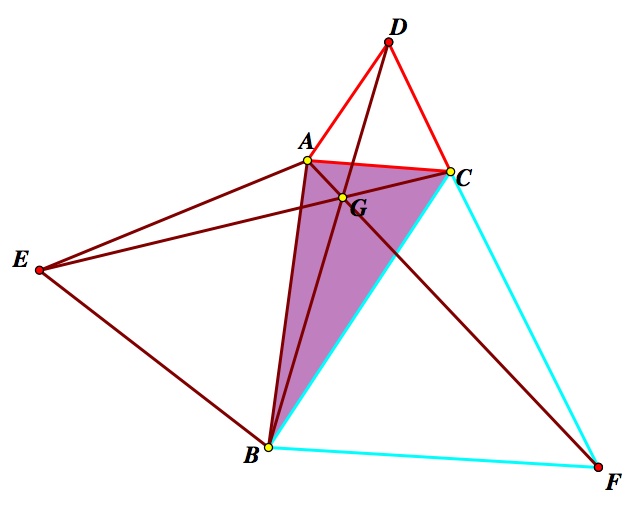
2) If on DEF, we make the same construction as that on ABC, there will be three equilateral triangles FDH, FEI, DEJ. The three equal line segments FJ, DI, EH (shown as green segments in the second image) also concur at the point G.
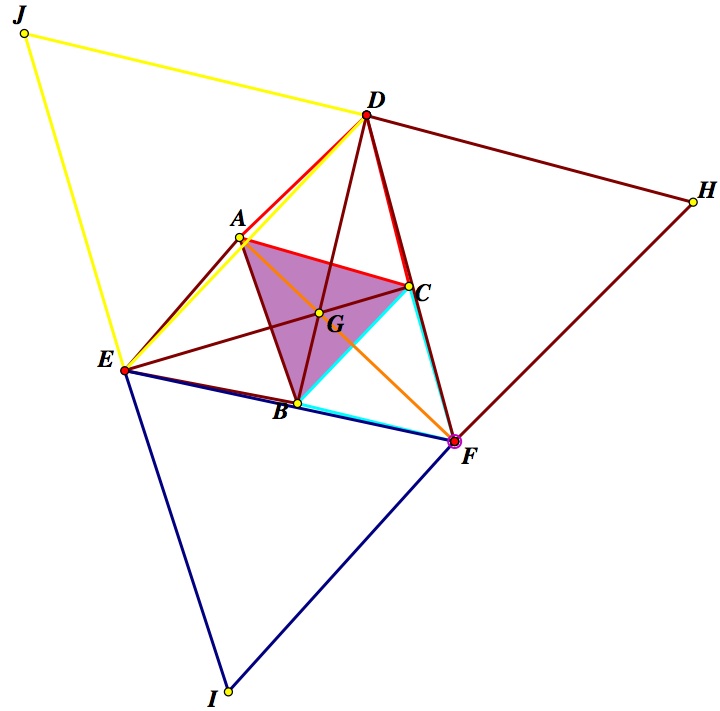
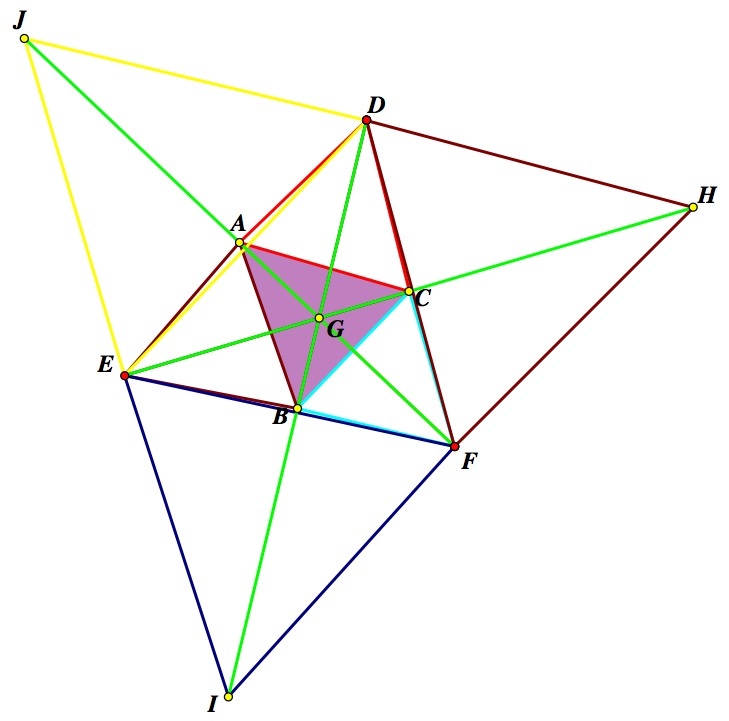
3) A, B, C are respectively the midpoints of segments FJ, DI, and EH.
For a GSP file regarding Lemoine's Problem and Kiepert's solution, click here.
V. Incorporating technology to teach Ceva's Theorem
One way to incorporate technology to teach Ceva's Theorem at the secondary level is to have the students complete an exploration of this Theorem using this GSP file. Having the students experience an exploration of Ceva's Theorem will increase the level of student engagement with this topic and motivate students to discover patterns and perhaps allow them to formulate the theorem themselves prior to having a class discussion regarding this Theorem. After the exploration, it is suggested to rigorously prove the Theorem with the class. To incorporate technology to prove Ceva's Theorem, consider using this GSP file.